Chrysler NVH.pdf

发布时间：2022-06-16 发布人：admin 分类：说明书资料大小：3.88M 资料格式：pdf 举报版权申诉

60578017-556f-41a0-ae8c-d678e27c74d0.pdf-第1页.png

第1页 / 共92页

60578017-556f-41a0-ae8c-d678e27c74d0.pdf-第2页.png

第2页 / 共92页

60578017-556f-41a0-ae8c-d678e27c74d0.pdf-第3页.png

第3页 / 共92页

60578017-556f-41a0-ae8c-d678e27c74d0.pdf-第4页.png

第4页 / 共92页

60578017-556f-41a0-ae8c-d678e27c74d0.pdf-第5页.png

第5页 / 共92页

60578017-556f-41a0-ae8c-d678e27c74d0.pdf-第6页.png

第6页 / 共92页

60578017-556f-41a0-ae8c-d678e27c74d0.pdf-第7页.png

第7页 / 共92页

60578017-556f-41a0-ae8c-d678e27c74d0.pdf-第8页.png

第8页 / 共92页

文本预览

VEHICLE NVH FUNDAMENTALS 汽车振动噪声平顺性基础 Dr. Chris Tsao

Preface The objective of this course is to give engineers at NEA Engineering an overview of NVH. The participants would be able to know: • What’s the theories behind NVH? • Which systems/components affect NVH? • What does NVH CAE do? • What are the NVH Test measures? • What are the commonly used terminologies? • What is the NVH Development Process? Cheng Cao, Ph.D. Chrysler NEA Internal Training Series 10-2009. 2

CONTENTS 1 2 3 4 5 6 7 8 9 Theory of Vibrations Vehicle NVH Overview Vehicle Vibration & Harshness Vehicle Noise NVH CAE NVH Evaluation & Measurement NVH Test Facilities NVH Development Commonly-used NVH Terms (English-Chinese) Cheng Cao, Ph.D. Chrysler NEA Internal Training Series 10-2009. 3

Theory of Vibrations Cheng Cao, Ph.D. Chrysler NEA Internal Training Series 10-2009. 4

Types of vibration Free vibration occurs when a mechanical system is set off with an initial input and then allowed to vibrate freely. Forced vibration is when an alternating force or motion is applied to a mechanical system. F Cheng Cao, Ph.D. Chrysler NEA Internal Training Series 10-2009. 5

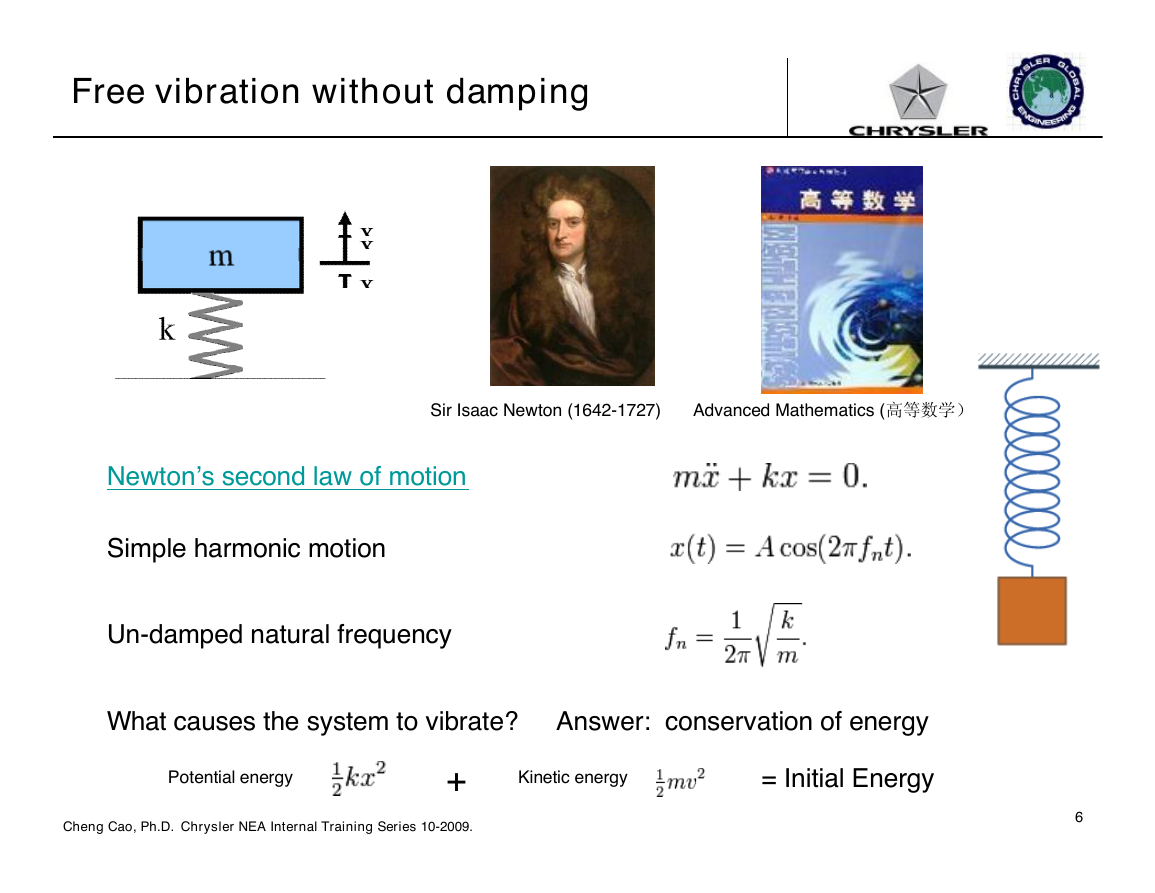
Free vibration without damping Sir Isaac Newton (1642-1727) Advanced Mathematics (高等数学） Newton’s second law of motion Simple harmonic motion Un-damped natural frequency What causes the system to vibrate? Answer: conservation of energy Potential energy + Kineticenergy = Initial Energy Cheng Cao, Ph.D. Chrysler NEA Internal Training Series 10-2009. 6

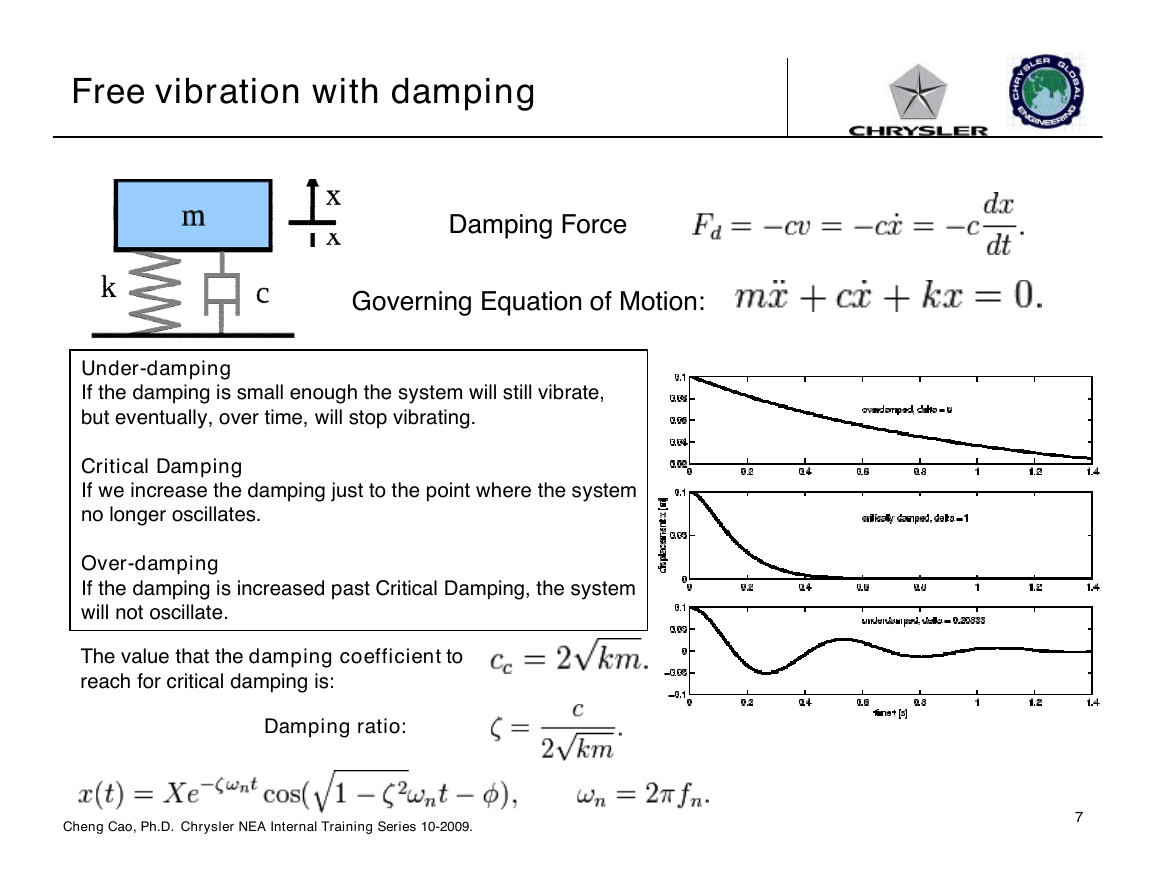
Free vibration with damping Damping Force Governing Equation of Motion: Under-damping If the damping is small enough the system will still vibrate, but eventually, over time, will stop vibrating. Critical Damping If we increase the damping just to the point where the system no longer oscillates. Over-damping If the damping is increased past Critical Damping, the system will not oscillate. The value that the damping coefficient to reach for critical damping is: Damping ratio: Cheng Cao, Ph.D. Chrysler NEA Internal Training Series 10-2009. 7

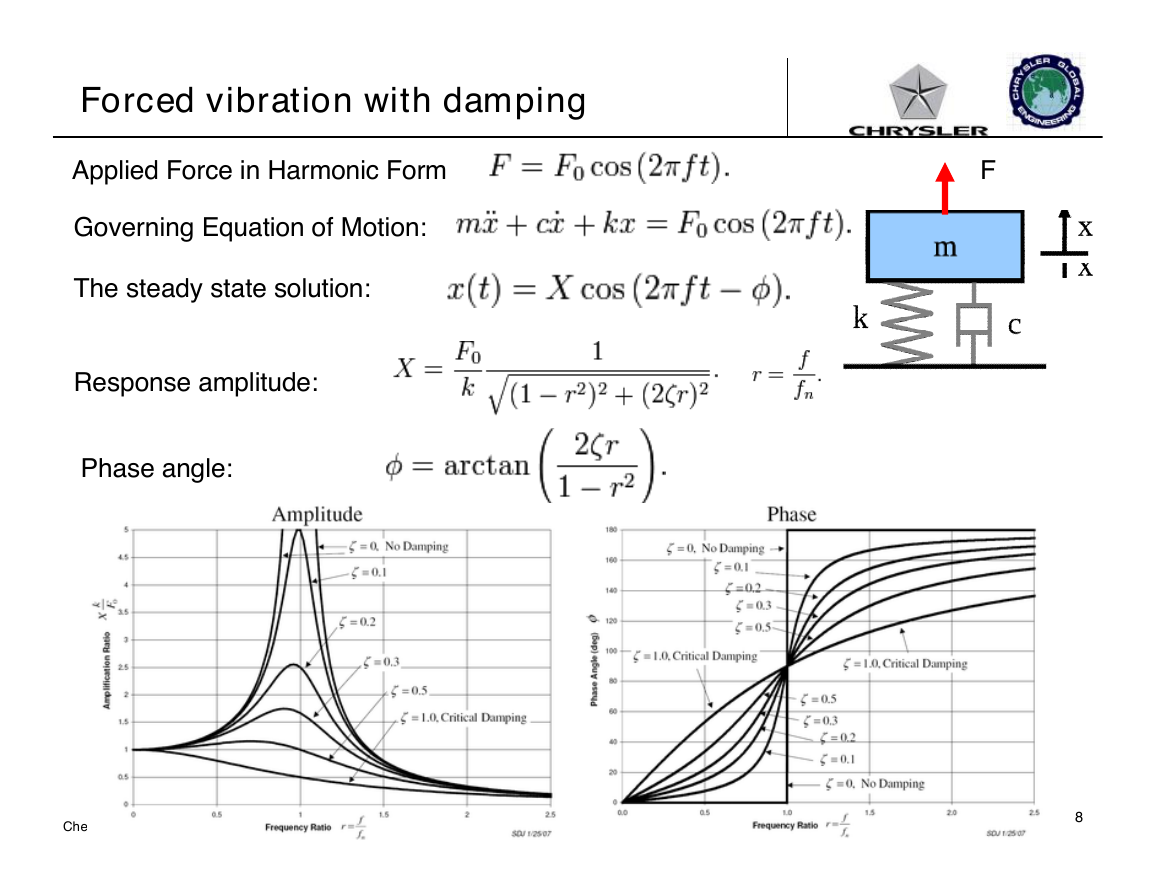
Forced vibration with damping Applied Force in Harmonic Form Governing Equation of Motion: The steady state solution: Response amplitude: Phase angle: F Cheng Cao, Ph.D. Chrysler NEA Internal Training Series 10-2009. 8

分享到：

赞收藏

资料库

Chrysler NVH.pdf

相关推荐

行业

热门标签

最新资料