Single Variable Calculus Concepts and Contexts 4th Edition.pdf

发布时间：2022-06-22 发布人：admin 分类：说明书资料大小：14.83M 资料格式：pdf 举报版权申诉

1082bc61-531f-47e9-8707-ae31105eaa3c.pdf-第1页.png

第1页 / 共795页

1082bc61-531f-47e9-8707-ae31105eaa3c.pdf-第2页.png

第2页 / 共795页

1082bc61-531f-47e9-8707-ae31105eaa3c.pdf-第3页.png

第3页 / 共795页

1082bc61-531f-47e9-8707-ae31105eaa3c.pdf-第4页.png

第4页 / 共795页

1082bc61-531f-47e9-8707-ae31105eaa3c.pdf-第5页.png

第5页 / 共795页

1082bc61-531f-47e9-8707-ae31105eaa3c.pdf-第6页.png

第6页 / 共795页

1082bc61-531f-47e9-8707-ae31105eaa3c.pdf-第7页.png

第7页 / 共795页

1082bc61-531f-47e9-8707-ae31105eaa3c.pdf-第8页.png

第8页 / 共795页

Front Cover

Title Page

Contents

Preface

To the Student

Diagnostic Tests

A Preview of Calculus

1. Functions and Models

1.1 Four Ways to Represent a Function

1.2 Mathematical Models: A Catalog of Essential Functions

1.3 New Functions from Old Functions

1.4 Graphing Calculators and Computers

1.5 Exponential Functions

1.6 Inverse Functions and Logarithms

1.7 Parametric Curves

Laboratory Project:Running Circles Around Circles

Review

Principles of Problem Solving

2. Limits and Derivatives

2.1 The Tangent and Velocity Problems

2.2 The Limit of a Function

2.3 Calculating Limits Using the Limit Laws

2.4 Continuity

2.5 Limits Involving Infinity

2.6 Derivatives and Rates of Change

Writing Project:Early Methods for Finding Tangents

2.7 The Derivative as a Function

2.8 What Does f' Say about f?

Review

Focus on Problem Solving

3. Differentiation Rules

3.1 Derivatives of Polynomials and Exponential Functions

Applied Project ■ Building a Better Roller Coaster

3.2 The Product and Quotient Rules

3.3 Derivatives of Trigonometric Functions

3.4 The Chain Rule

Laboratory Project:Bézier Curves

Applied Project:Where Should a Pilot Start Descent?

3.5 Implicit Differentiation

3.6 Inverse Trigonometric Functions and Their Derivatives

3.7 Derivatives of Logarithmic Functions

Discovery Project:Hyperbolic Functions

3.8 Rates of Change in the Natural and Social Sciences

3.9 Linear Approximations and Differentials

Laboratory Project:Taylor Polynomials

Review

Focus on Problem Solving

4. Applications of Differentiation

4.1 Related Rates

4.2 Maximum and Minimum Values

Applied Project:The Calculus of Rainbows

4.3 Derivatives and the Shapes of Curves

4.4 Graphing with Calculus and Calculators

4.5 Indeterminate Forms and l’Hospital’s Rule

Writing Project:The Origins of l’Hospital’s Rule

4.6 Optimization Problems

Applied Project ■ The Shape of a Can

4.7 Newton’s Method

4.8 Antiderivatives

Review

Focus on Problem Solving

5. Integrals

5.1 Areas and Distances

5.2 The Definite Integral

5.3 Evaluating Definite Integrals

Discovery Project:Area Functions

5.4 The Fundamental Theorem of Calculus

Writing Project:Newton, Leibniz, and the Invention of Calculus

5.5 The Substitution Rule

5.6 Integration by Parts

5.7 Additional Techniques of Integration

5.8 Integration Using Tables and Computer Algebra Systems

Discovery Project:Patterns in Integrals

5.9 Approximate Integration

5.10 Improper Integrals

Review

Focus on Problem Solving

6. Applications of Integration

6.1 More About Areas

6.2 Volumes

Discovery Project:Rotating on a Slant

6.3 Volumes by Cylindrical Shells

6.4 Arc Length

Discovery Project:Arc Length Contest

6.5 Average Value of a Function

Applied Project:Where To Sit at the Movies

6.6 Applications to Physics and Engineering

Discovery Project:Complementary Coffee Cups

6.7 Applications to Economics and Biology

6.8 Probability

Review

Focus on Problem Solving

7. Differential Equations

7.1 Modeling with Differential Equations

7.2 Direction Fields and Euler’s Method

7.3 Separable Equations

Applied Project:How Fast Does a Tank Drain?

Applied Project:Which Is Faster, Going Up or Coming Down?

7.4 Exponential Growth and Decay

Applied Project:Calculus and Baseball

7.5 The Logistic Equation

7.6 Predator-Prey Systems

Review

Focus on Problem Solving

8. Infinite Sequences and Series

8.1 Sequences

Laboratory Project:Logistic Sequences

8.2 Series

8.3 The Integral and Comparison Tests; Estimating Sums

8.4 Other Convergence Tests

8.5 Power Series

8.6 Representations of Functions as Power Series

8.7 Taylor and Maclaurin Series

Laboratory Project:An Elusive Limit

Writing Project:How Newton Discovered the Binomial Series

8.8 Applications of Taylor Polynomials

Applied Project:Radiation from the Stars

Review

Focus on Problem Solving

Appendixes

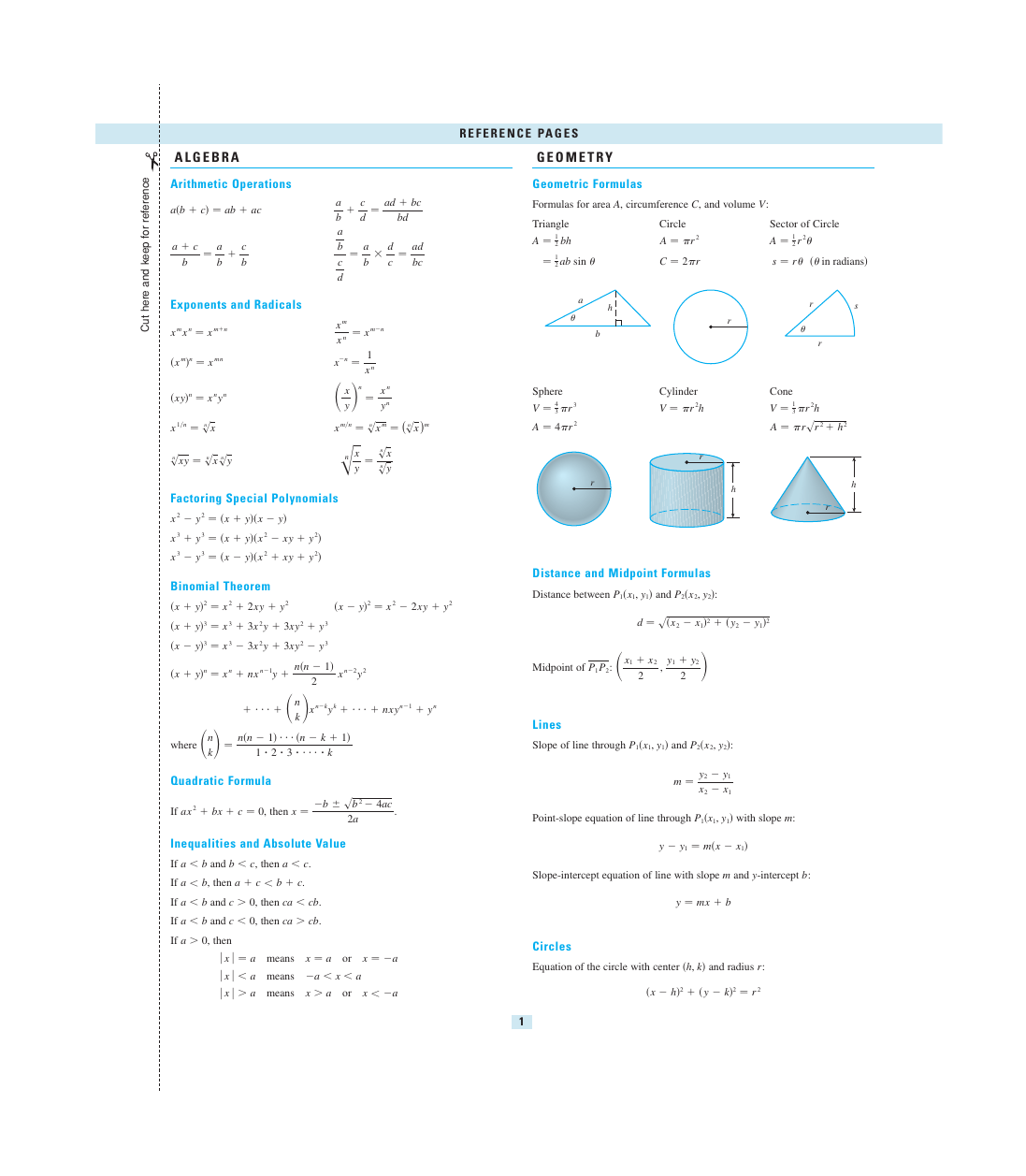
A: Intervals, Inequalities, and Absolute Values

B: Coordinate Geometry

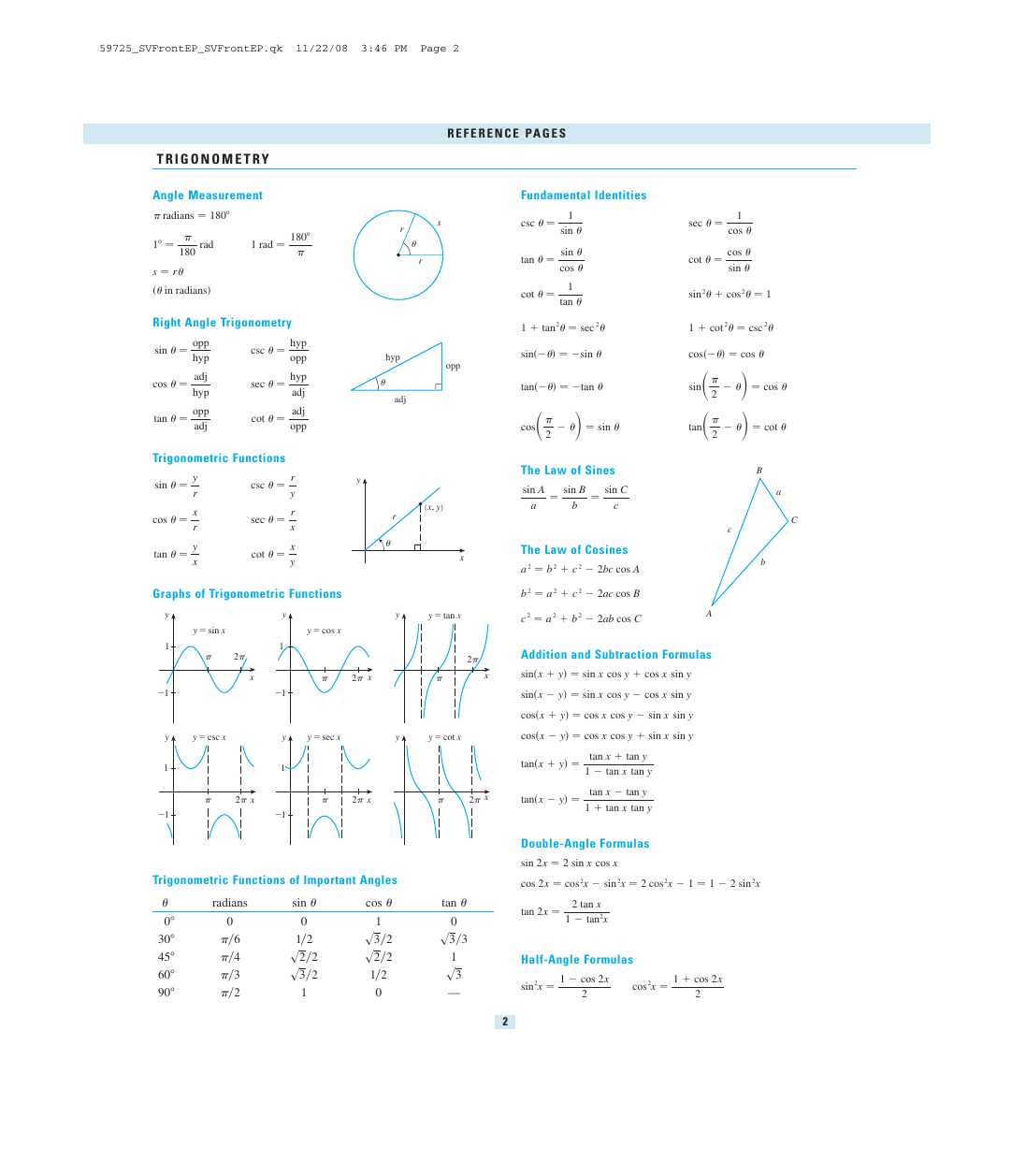
C: Trigonometry

D: Precise Definitions of Limits

E: A Few Proofs

F: Sigma Notation

G: Integration of Rational Functions by Partial Fractions

H: Polar Coordinates

I: Complex Numbers

J: Answers to Odd-Numbered Exercises

Index

e c n e r e f e r r o f p e e k d n a e r e h t u C A L G E B R A Arithmetic Operations a共b ⫹ c兲苷 ab ⫹ ac a ⫹ c b 苷 a b ⫹ c b Exponents and Radicals x mx n 苷 x m⫹n 共x m兲n 苷 x mn 共xy兲n 苷 x nyn x 1兾n 苷 sn x sn xy 苷 sn xsn y ⫹ c d 苷 ad ⫹ bc bd 苷 a b ⫻ d c 苷 ad bc a b a b c d 苷 x m⫺n x m x n x⫺n 苷 1 x n 苷 x n yn y 冉 x 冊n n冑x y 苷 sn x sn y x m兾n 苷 sn x m 苷 (sn x)m Factoring Special Polynomials x 2 ⫺ y2 苷共x ⫹ y兲共x ⫺ y兲 x 3 ⫹ y3 苷共x ⫹ y兲共x 2 ⫺ xy ⫹ y2兲 x 3 ⫺ y3 苷共x ⫺ y兲共x 2 ⫹ xy ⫹ y2兲 Binomial Theorem 共x ⫹ y兲2 苷 x 2 ⫹ 2xy ⫹ y2 共x ⫹ y兲3 苷 x 3 ⫹ 3x 2y ⫹ 3xy2 ⫹ y3 共x ⫺ y兲3 苷 x 3 ⫺ 3x 2y ⫹ 3xy2 ⫺ y3 n共n ⫺ 1兲共x ⫹ y兲n 苷 x n ⫹ nx n⫺1y ⫹ 共x ⫺ y兲2 苷 x 2 ⫺ 2xy ⫹ y2 x n⫺2y2 ⫹ ⭈ ⭈ ⭈ ⫹冉 n 2 冊x n⫺kyk ⫹ ⭈ ⭈ ⭈ ⫹ nxyn⫺1 ⫹ yn 冉n k where 冊苷 n共n ⫺ 1兲 ⭈ ⭈ ⭈ 共n ⫺ k ⫹ 1兲 k 1 ⴢ 2 ⴢ 3 ⴢ ⭈ ⭈ ⭈ ⴢ k Quadratic Formula If ax 2 ⫹ bx ⫹ c 苷 0 , then x 苷 ⫺b ⫾ sb 2 ⫺ 4ac 2a . Inequalities and Absolute Value R E F E R E N C E PA G E S G E O M E T RY Geometric Formulas Formulas for area A, circumference C, and volume V: Triangle A 苷 1 2 bh 苷 1 2 ab sin ␪ Circle A 苷 ␲r 2 C 苷 2␲r Sector of Circle A 苷 1 s 苷 r␪ 共␪ in radians兲 2 r 2␪ a ¨ h b Sphere V 苷 4 3 ␲r 3 A 苷 4␲r 2 r r h s r ¨ r Cone V 苷 1 A 苷 ␲rsr 2 ⫹ h2 3 ␲r 2h h r Cylinder V 苷 ␲r 2h r Distance and Midpoint Formulas P2共x2, y2兲 : Distance between P1共x1, y1兲 and d 苷 s共x2 ⫺ x1兲2 ⫹ 共 y2 ⫺ y1兲2 Midpoint of P1P2 : 冉 x1 ⫹ x2 2 , y1 ⫹ y2 2 冊 Lines Slope of line through P1共x1, y1兲 and P2共x2, y2兲 : m 苷 y2 ⫺ y1 x2 ⫺ x1 Point-slope equation of line through P1共x1, y1兲 with slope m: y ⫺ y1 苷 m共x ⫺ x1兲 Slope-intercept equation of line with slope m and y-intercept b: y 苷 mx ⫹ b and , then and and , then b ⬍ c a ⬍ c . a ⫹ c ⬍ b ⫹ c . c ⬎ 0 c ⬍ 0 ca ⬍ cb . ca ⬎ cb . , then , then If If If If If a ⬍ b a ⬍ b a ⬍ b a ⬍ b a ⬎ 0 , then ⱍ xⱍ 苷 a ⱍ xⱍ ⬍ a ⱍ xⱍ ⬎ a means means means or x 苷 a ⫺a ⬍ x ⬍ a x ⬎ a or x 苷 ⫺a x ⬍ ⫺a Circles Equation of the circle with center 共h, k兲 and radius r: 共x ⫺ h兲2 ⫹ 共 y ⫺ k兲2 苷 r 2 1

59725_SVFrontEP_SVFrontEP.qk 11/22/08 3:46 PM Page 2 R E F E R E N C E PA G E S T R I G O N O M E T RY Angle Measurement ␲ radians 苷 180⬚ 1 rad 苷 180⬚ ␲ 1⬚ 苷 ␲ 180 rad s 苷 r␪ 共␪ in radians兲 Right Angle Trigonometry sin ␪苷 opp hyp cos ␪苷 adj hyp tan ␪苷 opp adj csc ␪苷 hyp opp sec ␪苷 hyp adj cot ␪苷 adj opp Trigonometric Functions sin ␪苷 y csc ␪苷 r r y cos ␪苷 x sec ␪苷 r r x tan ␪苷 y cot ␪苷 x x y Graphs of Trigonometric Functions s r ¨ r opp hyp ¨ adj Fundamental Identities csc ␪苷 1 sin ␪ tan ␪苷 sin ␪ cos ␪ cot ␪苷 1 tan ␪ 1 ⫹ tan2␪苷 sec 2␪ sin共⫺␪兲苷 ⫺sin ␪ tan共⫺␪兲苷 ⫺tan ␪ cos冉 ␲ ⫺ ␪冊苷 sin ␪ 2 y r ¨ The Law of Sines sin A 苷 sin B b 苷 sin C c (x, y) a x The Law of Cosines a 2 苷 b 2 ⫹ c 2 ⫺ 2bc cos A b 2 苷 a 2 ⫹ c 2 ⫺ 2ac cos B sec ␪苷 1 cos ␪ cot ␪苷 cos ␪ sin ␪ sin2␪⫹ cos2␪苷 1 1 ⫹ cot 2␪苷 csc 2␪ cos共⫺␪兲苷 cos ␪ sin冉 ␲ tan冉 ␲ 2 ⫺ ␪冊苷 cos ␪ ⫺ ␪冊苷 cot ␪ 2 a C B b c y 1 _1 y 1 _1 y=sin x π 2π x y=csc x π 2π x y 1 _1 y 1 _1 y=cos x y y=tan x c 2 苷 a 2 ⫹ b 2 ⫺ 2ab cos C A π 2π x π 2π x y=sec x y y=cot x π 2π x π 2π x Addition and Subtraction Formulas sin共x ⫹ y兲苷 sin x cos y ⫹ cos x sin y sin共x ⫺ y兲苷 sin x cos y ⫺ cos x sin y cos共x ⫹ y兲苷 cos x cos y ⫺ sin x sin y cos共x ⫺ y兲苷 cos x cos y ⫹ sin x sin y tan共x ⫹ y兲苷 tan x ⫹ tan y 1 ⫺ tan x tan y tan共x ⫺ y兲苷 tan x ⫺ tan y 1 ⫹ tan x tan y Trigonometric Functions of Important Angles cos ␪ radians sin ␪ 0 ␲兾6 ␲兾4 ␲兾3 ␲兾2 0 1兾2 s2兾2 s3兾2 1 1 s3兾2 s2兾2 1兾2 0 ␪ 0⬚ 30⬚ 45⬚ 60⬚ 90⬚ Double-Angle Formulas sin 2x 苷 2 sin x cos x cos 2x 苷 cos2x ⫺ sin2x 苷 2 cos2x ⫺ 1 苷 1 ⫺ 2 sin2x tan 2x 苷 2 tan x 1 ⫺ tan2x Half-Angle Formulas sin2x 苷 1 ⫺ cos 2x 2 cos2x 苷 1 ⫹ cos 2x 2 tan ␪ 0 s3兾3 1 s3 — 2

59726_SV_FM_FM_pi-xxiii.qk 11/22/08 3:08 PM Page i Single Variable Calculus Concepts and Contexts | 4e

59726_SV_FM_FM_pi-xxiii.qk 11/22/08 3:08 PM Page ii Calculus and the Architecture of Curves The cover photograph shows the DZ Bank in Berlin, designed and built 1995–2001 by Frank Gehry and Associates. The interior atrium is dominated by a curvaceous four- story stainless steel sculptural shell that suggests a prehistoric creature and houses a central con- ference space. The highly complex structures that Frank Gehry designs would be impossible to build without the computer. The CATIA software that his archi- tects and engineers use to produce the computer models is based on principles of calculus—ﬁtting curves by matching tangent lines, making sure the curvature isn’t too large, and controlling parametric surfaces. “Consequently,” says Gehry, “we have a lot of freedom. I can play with shapes.” The process starts with Gehry’s initial sketches, which are translated into a succes- sion of physical models. (Hundreds of different physical models were constructed during the design of the building, ﬁrst with basic wooden blocks and then evolving into more sculptural forms.) Then an engineer uses a digitizer to record the coordinates of a series of points on a physical model. The digitized points are fed into a computer and the CATIA software is used to link these points with smooth curves. (It joins curves so that their tangent lines coincide; you can use the same idea to design the shapes of letters in the Laboratory Project on page 208 of this book.) The architect has considerable free- dom in creating these curves, guided by displays of the curve, its derivative, and its curvature. Then the curves are y r h e G . O k n a r F f o y s e t r u o C y r h e G . O k n a r F f o y s e t r u o C y r h e G . O k n a r F f o y s e t r u o C

59726_SV_FM_FM_pi-xxiii.qk 11/22/08 3:08 PM Page iii y r h e G . O k n a r F f o y s e t r u o C y r h e G . O k n a r F f o y s e t r u o C m o c . e v i h c r a r e y a m s a m o h t connected to each other by a parametric surface, and again the architect can do so in many possible ways with the guidance of displays of the geometric characteristics of the surface. The CATIA model is then used to produce another physical model, which, in turn, suggests modiﬁcations and leads to additional computer and physical models. The CATIA program was developed in France by Dassault Systèmes, originally for designing airplanes, and was subsequently employed in the automotive industry. Frank Gehry, because of his complex sculptural shapes, is the ﬁrst to use it in architecture. It helps him answer his ques- tion, “How wiggly can you get and still make a building?”

59726_SV_FM_FM_pi-xxiii.qk 11/22/08 3:08 PM Page iv

59726_SV_FM_FM_pi-xxiii.qk 11/22/08 3:08 PM Page v Single Variable Calculus Concepts and Contexts | 4e James Stewart McMaster University and University of Toronto Australia . Brazil . Japan . Korea . Mexico . Singapore . Spain . United Kingdom . United States

分享到：

赞收藏

资料库

Single Variable Calculus Concepts and Contexts 4th Edition.pdf

相关推荐

考试认证

热门标签

最新资料