网格质量，提高网格质量的方法.pdf

发布时间：2022-06-08 发布人：admin 分类：说明书资料大小：0.43M 资料格式：pdf 举报版权申诉

csx231007-3851211-4744302543455414070.pdf-第1页.png

第1页 / 共9页

csx231007-3851211-4744302543455414070.pdf-第2页.png

第2页 / 共9页

csx231007-3851211-4744302543455414070.pdf-第3页.png

第3页 / 共9页

csx231007-3851211-4744302543455414070.pdf-第4页.png

第4页 / 共9页

csx231007-3851211-4744302543455414070.pdf-第5页.png

第5页 / 共9页

csx231007-3851211-4744302543455414070.pdf-第6页.png

第6页 / 共9页

csx231007-3851211-4744302543455414070.pdf-第7页.png

第7页 / 共9页

csx231007-3851211-4744302543455414070.pdf-第8页.png

第8页 / 共9页

文本预览

Mesh Metric Mesh Metric 2010-01-12 1 By Eric

Element Quality Element Quality Element Quality t Q lit El This metric is based on the ratio of the volume to the edge length for a given element. Range: 0 – 1 Rule: 见下图。 1最好，0最差。网格体积为0或者为负体积 0 差正方体网格 1 好 2010-01-12 2 By Eric

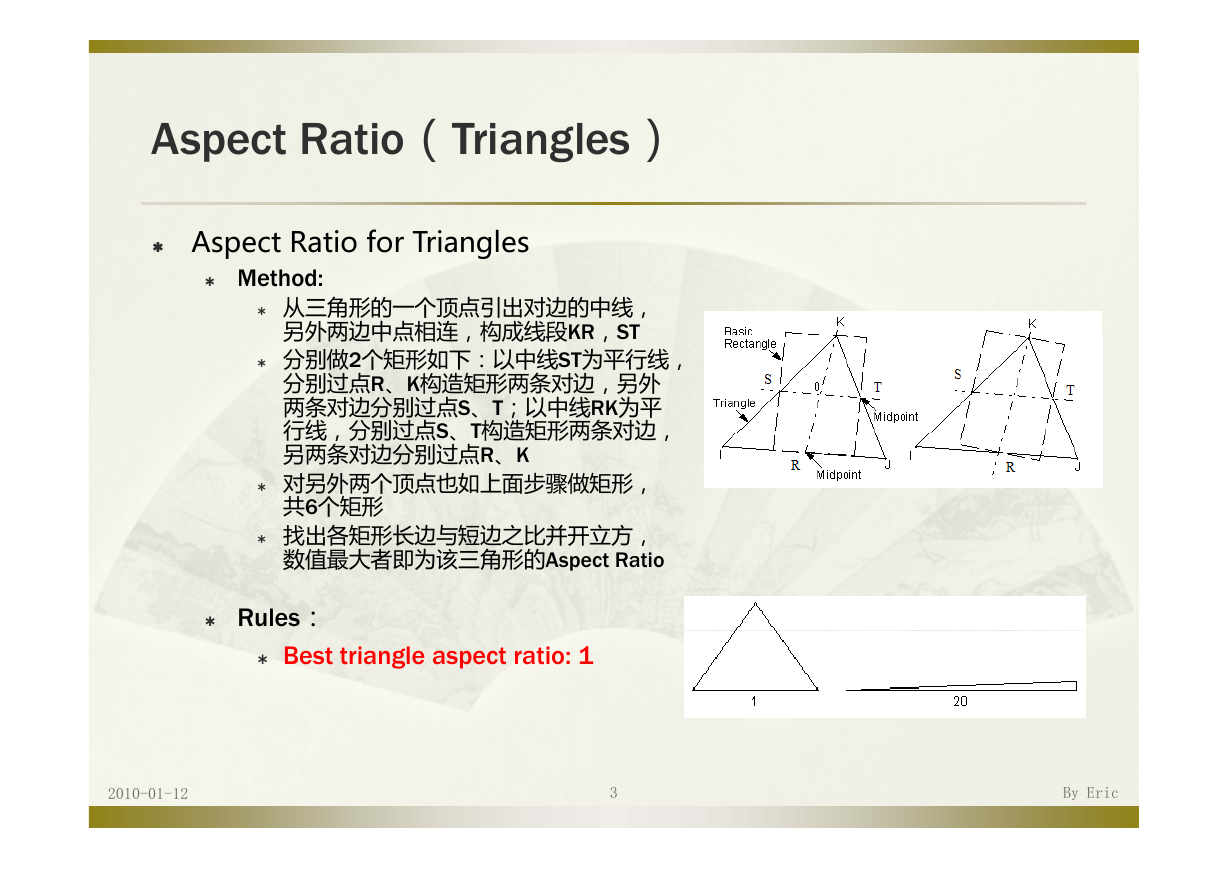
Aspect Ratio（Triangles） Aspect Ratio（Triangles） Aspect Ratio for Triangles T i l t R ti A Method: f 从三角形的一个顶点引出对边的中线，另外两边中点相连构成线段KR ST 另外两边中点相连，构成线段KR，ST 分别做2个矩形如下：以中线ST为平行线，分别过点R、K构造矩形两条对边，另外两条对边分别过点S、T；以中线RK为平行线分别过点S T构造矩形两条对边行线，分别过点S、T构造矩形两条对边，另两条对边分别过点R、K 对另外两个顶点也如上面步骤做矩形，共6个矩形找出各矩形长边与短边之比并开立方找出各矩形长边与短边之比并开立方，数值最大者即为该三角形的Aspect Ratio Rules： Best triangle aspect ratio: 1 2010-01-12 3 By Eric

Aspect Ratio (Quadrilaterals) Aspect Ratio (Quadrilaterals) Aspect Ratio Calculation for Quadilaterals l f Q dil t t R ti C l l ti A Method: 如果单元不在一个平面上，各个节点将被投影到节点坐标平均值所在的平面上画出两条矩形对边中点的连线，相交于一点O 以交点O为中心，分别过4个中点构造2个矩形。找出两个矩形长边和短边之比的最大值，即为四边形的Aspect Ratio。最，即为形个形和 p Rule：矩形网格最佳边长比为1，即该矩形为正方形。 2010-01-12 4 By Eric

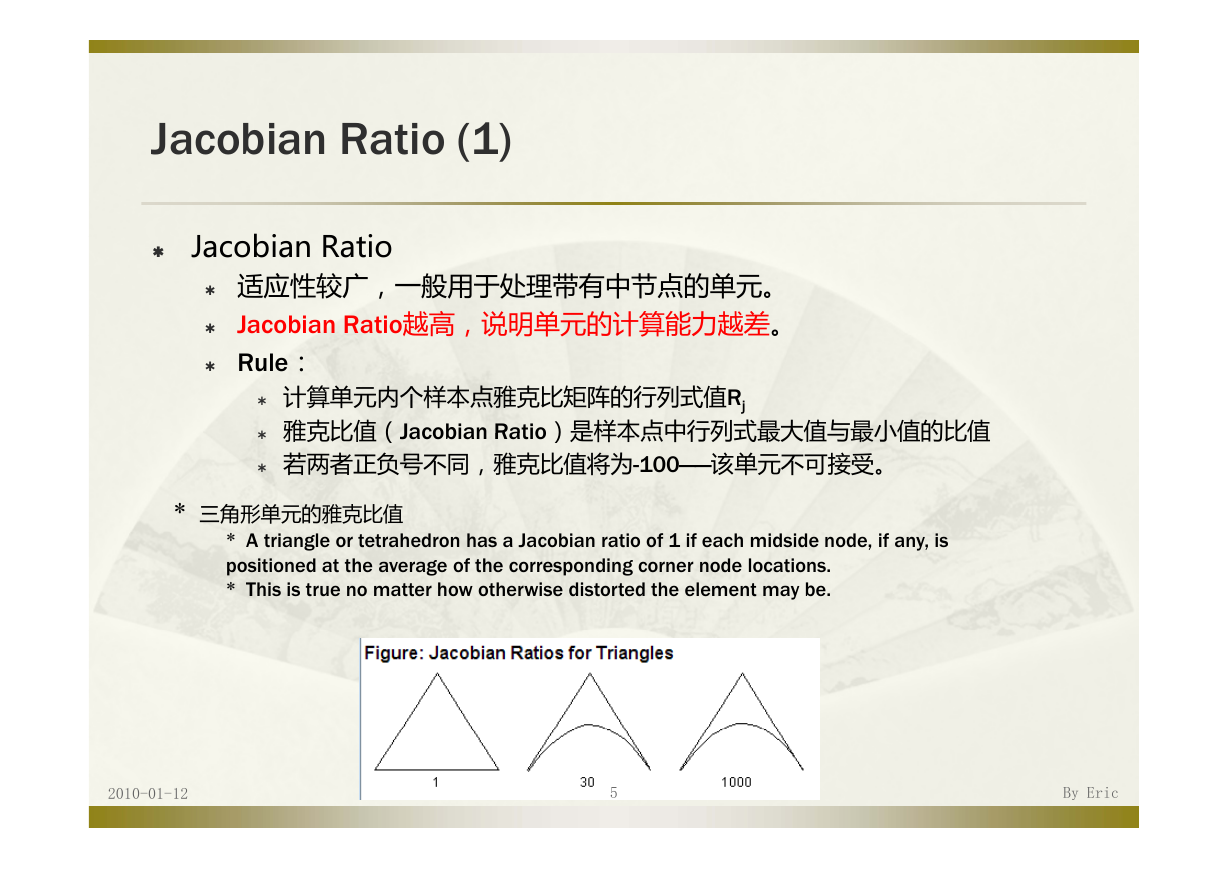
Jacobian Ratio (1) Jacobian Ratio (1) bi J R ti Jacobian Ratio 适应性较广，一般用于处理带有中节点的单元。 Jacobian Ratio越高，说明单元的计算能力越差。越高，说明单元的计算能力越差。 Rule：计算单元内个样本点雅克比矩阵的行列式值Rj 雅克比值（Jacobian Ratio）是样本点中行列式最大值与最小值的比值雅克比值（Jacobian Ratio）是样本点中行列式最大值与最小值的比值若两者正负号不同，雅克比值将为-100——该单元不可接受。 * 三角形单元的雅克比值 * A triangle or tetrahedron has a Jacobian ratio of 1 if each midside node, if any, is positioned at the average of the corresponding corner node locations. * This is true no matter how otherwise distorted the element may be. 2010-01-12 5 By Eric

Jacobian Ratio (2) Jacobian Ratio (2) 四边形单元的雅克比值：四边形单元的雅克比值 Any rectangle or rectangular d parallelepiped having no midside t nodes, or having midside nodes at the midpoints of its edges, has a Jacobian ratio of 1. h i g id id d Moving midside nodes toward or Moving midside nodes toward or away from each other can increase the Jacobian ratio. 六面体单元雅克比值：六面体单元雅克比值 A quadrilateral or brick has a Jacobian ratio of 1 if l i ll ll ( ) i (a) its opposing faces are all parallel to f each other, and (b) each midside node, if any, is positioned at the average of the corresponding corner node locations. corresponding corner node locations. 2010-01-12 6 By Eric

Warping Factor Warping Factor Warping factor W i Computed and tested for some quadrilateral shell elements, and the t f quadrilateral faces of bricks, wedges, and pyramids. A high factor may indicate a condition the underlying element formulation cannot handle well, or may simply hint at a mesh generation flaw. Rule: Rule: Best: 0 （此时为平面，无扭曲） 3D网格的扭曲系数分别比较六个面的扭曲系数，取最大值 2010-01-12 7 d 45° l ti Twisting the top face of a unit cube by 22.5° and 45° relative to the base produces warping factors of about 0.2 and 0.4, respectively. th b d t By Eric

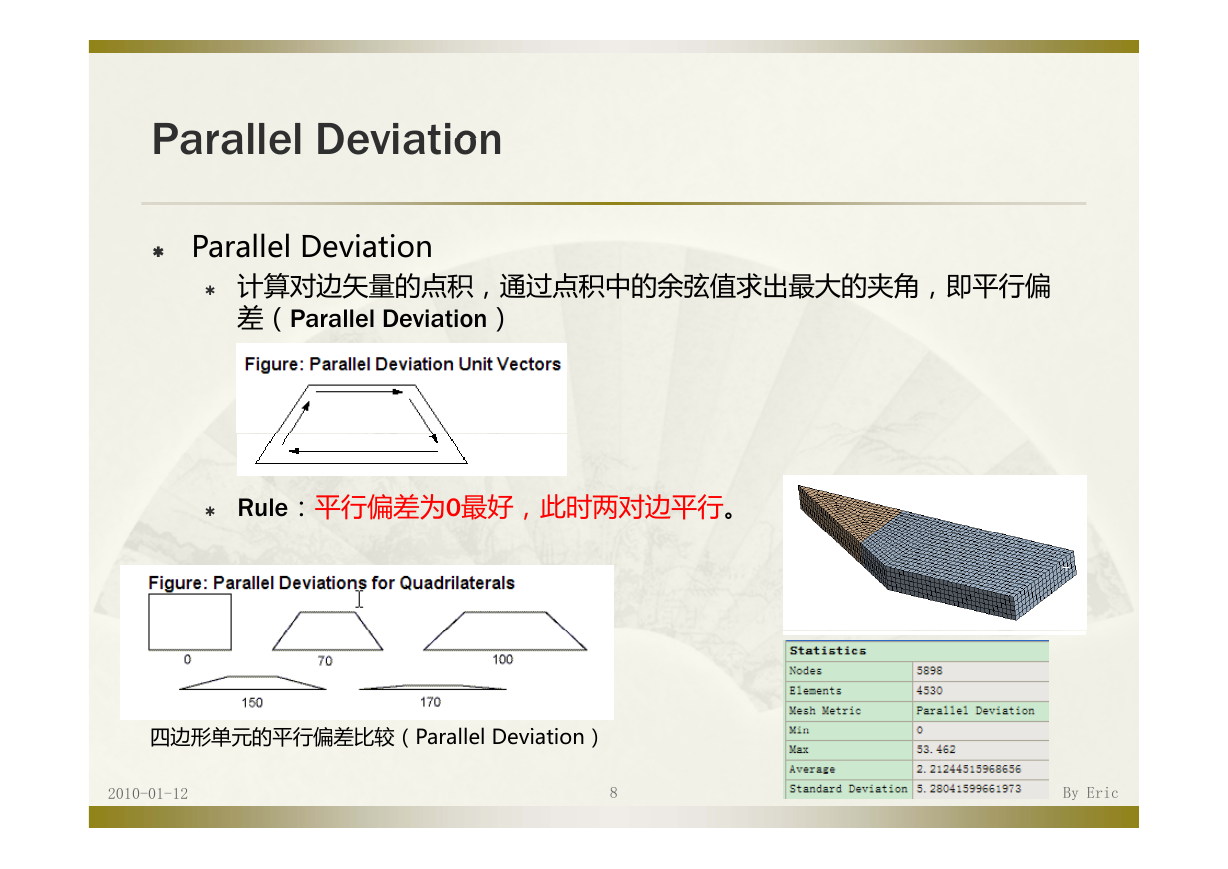
Parallel Deviation Parallel Deviation Parallel Deviation P 计算对边矢量的点积，通过点积中的余弦值求出最大的夹角，即平行偏 l D i ti ll 差（Parallel Deviation） Rule：平行偏差为0最好，此时两对边平行。四边形单元的平行偏差比较（P i ）四边形单元的平行偏差比较（Parallel Deviation） l D i ll 2010-01-12 8 By Eric

分享到：

赞收藏

资料库

网格质量，提高网格质量的方法.pdf

相关推荐

课程资源

热门标签

最新资料