第1页 / 共62页
第2页 / 共62页
第3页 / 共62页
第4页 / 共62页
第5页 / 共62页
第6页 / 共62页
第7页 / 共62页
第8页 / 共62页
Gilbert_Strang-Linear_Algebra_and_Its_Applications_4ed_solutions.pdf
Solutions to Selected Exercises
Problem Set 1.2, page 9
s
-
1
1. The lines intersect at (x, y) = (3, 1). Then 3(column 1) + I(column 2) = (4, 4).
3. These "planes" intersect in a line in four-dimensional space. The fourth plane nor-
mally intersects that line in a point. An inconsistent equation like u + w = 5 leaves
no solution (no intersection).
+
-
r
5. The two points on the plane are (1, 0, 0, 0) and (0, 1, 0, 0).
7. Solvable for (3, 5, 8) and (1, 2, 3); not solvable for b = (3, 5, 7) orb = (1, 2, 2).
9. Column 3 = 2(column 2) - column 1.Ifb = (0, 0, 0), then (u, v, w) _ (c, -2c, c).
11. Both a = 2 and a = -2 give a line of solutions. All other a give x = 0, y = 0.
13. The row picture has two lines meeting at (4, 2). The column picture has 4(1, 1) +
.
t
i
r
-
i
1
-
1
2(-2, 1) = 4(column 1) + 2(column 2) = right-hand side (0, 6).
15. The row picture shows four lines. The column picture is infour-dimensional space.
No solution unless the right-hand side is a combination of the two columns.
17. If x, y, z satisfy the first two equations, they also satisfy the third equation. The line
L of solutions contains v = (1, 1, 0), w = (2, 1, 1), and u = 1v + 1w, and all
combinations cv + dw with c + d = 1.
2
2
2
r
-
1
19. Column 3 = column 1; solutions (x, y, z) _ (1, 1, 0) or (0, 1, 1) and you can add
C
3
'
any multiple of (-1, 0, 1); b = (4, 6, c) needs c = 10 for solvability.
21. The second plane and row 2 of the matrix and all columns of the matrix are changed.
The solution is not changed.
23. u = 0, v = 0; w = 1, because 1(column 3) = b.
Problem Set 1.3, page 15
1. Multiply by £ = z = 5, and subtract to find 2x + 3y = 1 and -6y = 6. Pivots
2, -6.
3. Subtract -1 times equation 1 (or add 1 times equation 1). The new second equation
is 3y = 3. Then y = 1 and x = 5. If the right-hand side changes sign, so does the
solution: (x, y) = (-5, -1).
l
f
.
2
-
-
S
I
2
N
5. 6x + 4y is 2 times 3x + 2y. There is no solution unless the right-hand side is
2. 10 = 20. Then all points on the line 3x + 2y = 10 are solutions, including (0, 5)
and (4, -1).
7. If a = 2, elimination must fail. The equations have no solution. If a = 0, elimination
stops for a row exchange. Then 3y = -3 gives y = -1 and 4x + 6y = 6 gives
x = 3.
9. 6x - 4y is 2 times (3x - 2y). Therefore, we need b2 = 2b1. Then there will be
infinitely many solutions. The columns (3, 6) and (-2, -4) are on the same line.
Solutions to Selected Exercises
429
2x - 3y = 3
11. 2x - 3y = 3
x = 3
y + z = 1 gives y + z = 1 and y= 1
2y - 3z = 2
z = 0
Subtract 2 x row 1 from row 2
Subtract 1 x row 1 from row 3
Subtract 2 x row 2 from row 3.
13. The second pivot position will contain -2 - b. If b = -2, we exchange with row
3. If b = -1 (singular case), the second equation is -y - z = 0. A solution is
(1, 1, -1).
- 5z =0
15. If row 1 = row 2, then row 2 is zero after the first step; exchange the zero row with
row 3 and there is no third pivot. If column 1 = column 2, there is no second pivot.
17. Row 2 becomes 3y - 4z = 5, then row 3 becomes (q + 4)z = t - 5. If q = -4,
the system is singular - no third pivot. Then, if t = 5, the third equation is 0 = 0.
Choosing z = 1, the equation 3y - 4z = 5 gives y = 3 and equation 1 gives
x=-9.
l
f
.
,
,
°
f
19. The system is singular if row 3 is a combination of rows 1 and 2. From the end view,
the three planes form a triangle. This happens if rows 1+ 2 = row 3 on the left-hand
side but not the right-hand side: for example, x + y + z = 0, x - 2y - z = 1,
2x - y = 9. No two planes are parallel, but still no solution.
21. The fifth pivot is s . The nth pivot is ("+1)1) .
23. Triangular system
u+ v+ w= 2
2v + 2w = -2
2w= 2
u = 3
Solution v = -2.
w= 1
25. (u, v, w) = (3/2, 1/2,. -3). Change to +1 would make the system singular (2 equal
columns).
27. a = 0 requires a row exchange, but the system is nonsingular: a = 2 makes it singular
(one pivot, infinity of solutions); a = -2 makes it singular (one pivot, no solution).
29. The second term be + ad is (a + b)(c + d) - ac - bd (only 1 additional
n
-
'
A
.
'
multiplication).
.
,
31. Elimination fails for a = 2 (equal columns), a = 4 (equal rows), a = 0 (zero
i
v
.
column).
Problem Set 1.4, page 26
1.
17
4 ,
17
5
-2 ,
3.
4
[f].
With sides to (2, 1) and (0, 3), the parallelogram goes to (2, 4).
3. Inner products 54 and 0, column times row gives -6 -10 -2
3
21
5
35
1
7
5. Ax = (0, 0, 0), so x = (2, 1, 1) is a solution; other solutions are cx = (2c, c, c).
'
-
`
7. Examples: Diagonal
1
0
0
0
2
0
0
0
7
, symmetric
1
3
4
skew-symmetric
4
0
3
-3 0
0 .
-4 0 0
4
3
2 0 , triangular
0
7
1
0
0
3
2
0
4
0
7
430
Solutions to Selected Exercises
9. (a) all
(b) fil = ail/all
(d) second pivot a22 -
a21-a12.
all
(c) new aid is aid - ail`a11
all
11. The coefficients of rows of B are 2, 1, 4 from A. The first row of AB is [6 3].
1
],B=[g jc=[? ]D=AE=F=[
0
13. A= [1
15. AB1=B1Agivesb=c=0.AB2=B2Agivesa=d.SoA=aI.
17. A(A + B) + B(A + B), (A + B)(B + A), A2 + AB + BA + B2 always equal
-1].
+
-
I
1
(A + B)2.
19b] p q] _ [a+ [b[r s] -
p + br
21. AA; B_ 1 ()C = 0 o] = zero matrix.
c +dr c +ds
aq + bsc
d
d
r
s
23. E32E21b = (1, -5, -35) but E21E32b = (1, -5, 0). Then row 3 feels no effect
from row 1.
25. Changing a33 from 7 to 11 will change the third pivot from 5 to 9. Changing a33
from 7 to 2 will change the pivot from 5 to no pivot.
D
C
B
27. To reverse E31i add 7 times row 1 to row 3. The matrix is R31 = 0
7
1
0
1
0
0
0 .
1
29. E13=
1
0
0
31. E21 has f21
a+ b+ c= 4
33. a + 2b + 4c = 8
a+3b+9c=14
1
1
0
1 0 ; 0
0
1
1
0
1
0
1
2
0 ; E31 E13 = 0
1
1
0
1
0
1
0 . Test on the identity matrix!
1
E32 has 32 = - , E43 has f43 = - .Otherwise the E's match I .
I
M
A
gives
a=2
b = 1.
c=1
35. (a) Each column is E times a column of B.
[1 2 4- 2 4 8Rows
(b) EB = [11
2
Ol]
2
1
4
of EB are combinations of rows of B, so they are multiples of [1 2 4].
37. (row 3) x is
39. BA=3Iis5by5,AB=5Iis3by3,ABD=5Dis3byl,ABD:No,A(B+C):
a3 j xj, and (A2)11 = (row 1)
(column 1) =
No.
41. (a) B = 41.
(b) B = 0.
(d) Every row of B is 1, 0, 0.
0
0
(c) B = 0 1
0
1
1
0
0
43. (a) mn (every entry).
(b) mnp.
(c) n3 (th is is n2 dot product s).
1
45. 2 3 3 0] + 4 [1 2 1] = 6 6 0 + 4 8 4 = 10
2
1
6
6
0
1
2
1
7
l 0
[
0
01
]
3
3
0
3
3
14
8
0
4
1
0
47. A times,B is A
1
Solutions to Selected Exercises
431
I B, [-] [ I
I ],
I
I I [_].
49. The (2, 2) block S = D - CA-1 B is the Schur complement: blocks in d - (cb/a).
51. A times X = [x1 x2 x3] will be the identity matrix I = [Ax, Axe Ax3].
53.
[a+b a+b
(c + d c + dj agrees with [a + c b + d] when b = c and a = d.
ra+c b+d
55. 2x + 3y + z + 5t = 8 is Ax = b with the 1 by 4 matrix A = [2 3 1 5]. The
solutions x fill a 3D "plane" in four dimensions.
57. The dot product [1 4 5] y = (1 by 3)(3 by 1) is zero for points (x, y, z) on a
x
z
plane x + 4y + 5z = 0 in three dimensions. The columns of A are one-dimensional
vectors.
59. A * v = [3 4 5f and v' * v = 50; v * A gives an error message.
8
3
61. M= 1 5
7
6
4
9
2
5+u
5-u-v
5+v
5-u+v
5+u-v
5
5-v
5+u+v ;
5-u
M3(1, 1, 1) = (15, 15, 15); M4(1, 1, 1, 1) = (34, 34, 34, 34) because the numbers
1 to 16 add to 136, which is 4(34).
Problem Set 1.5, page 39
1. U is nonsingular 'when no entry on the main diagonal is zero.
3.
0
1
1
0
0
0
0
1
1
0
1
0-
0
1
-2,
-1 1
0
= 0 1 0 ; -2 1
0
1
2
0
-1 1-1 1
(E-1F j 1G_1)(GFE) -_- E-1F-1FE = E-lE = I; also (GFE)(E-1F-1G-1) =I.
2
2
-1
2
3
7; after elimination, 0
-1
0
0
1 0= I also.
-1 -1 1
0
1
-
0
2
-
r.
1
-
-
/
0
1
-
+
O
0
3
7
-1
U
v
w
2
N
O
0
O
0
K
3
5
0
3
5
0
0
0
1
1
-1
1
3
0
1
5. LU=
7. FGH=
1
0
0
0
2
0
0
0
1
2
1
0
00 ;HGF= 4
1
8
0
2
4
0
0
1
2
0
0
1
9. (a) Nonsingular when dld2d3 0 0.
[01
downward: Lc = b gives c = 1. Then
(b) Suppose d3 ; 0: Solve Lc = b going
d1
0
0
-d1
d2
0
0
-d2
d3
01
u
v = 10 gives
w
1
l/d3
x= 1/d3
1/d3
1
432
Solutions to Selected Exercises
5
11. Lc = b going downward gives c = -2 ; Ux = c upward gives x = -2
0
0
s
'
'
2
13. Permutation
rows 2 and 3
permutation
rows 1 and 2
15. PA =LDUis
PA = LDUis
17. L becomes
1
1
2
1
0
0
r
l
o
0
1
0
1
0
0
0
1
0
0
0
1
1
0
0
'
-
1
,
0
0
0
0
1
'
1,
0
1
0
0
0
0
00
0
0
1
0
1
0
0
1
2
1
2
1
2
u
v = -3
4
w
u
v
w
1
0
3
-
1
1
1
.
1
1
0
1 = 0 1
4
2
3
1
1
2
4 2 = 1
2
1
1
0
1
0
0
0
1
0
0
1
0
0
o 0
0
0
n
1
'
-
-1
o
0
+
1
0
0
1
0
0
0
0
-1 0
0
0
1
0
0
1
0
0
0
1
0
2
1
0
1
1
1
1
0
0
0
0 . MATLAB and other codes use PA = LU.
n
i
t
1
19. a = 4 leads to a row exchange; 3b + lOa = 40 leads to a singular matrix; c = 0
r
-
1
leads to a row exchange; c = 3 leads to a singular matrix.
21. 231 = 1 and 232 = 2 (233 = 1): reverse steps to recover x + 3y + 6z = 11 from
Ux = c: 1 times (x + y + z = 5) + 2 times (y + 2z = 2) + 1 times (z = 2) gives
x+3y+6z=11.
`
/
'
23.
1
0
0 -2 1
1
~
1
-0
2 1
N
0
°
1
1
1
1
A= 0 2 3 =U.
. 0 0 -6
A= 2 1 0 U=E211E321U=LU.
1
0
0
o
0
o
m
2
1
2 by 2: d = 0 not allowed;
1 1 2= 2 1
0
1
1
1
m n l
1
2
1
25.
27.
A= 0 3 9 hasL=landD=
2
4
0 0
8
7
o
a
S
g
e
f h
i
12
d=1, e=1, then 2=1
f = 0 is not allowed
no pivot in row 2.
1
7
A= L U has U = A (pivots on
2
the diagonal); A= LDU has U= D-lA = 0 1
0
0
1
4
3
1
with is on the diagonal.
a
b
b
b
a
b
a
b
c
c
c d
a
1
'
-
F
1
1
1
1
1
1
1
1
1-
a
a
a
b-a b-a b-a
d-c
c-b c-b Need b
a
1 11
1
1
+
1
-
F
1
-
1
0
ra
a
1 = LIU; a a+b
b
0
1
0
b
b+c
( same U).
0
1
0
1 0 c = 5 gives c = 1
4
4
1
1
6
rl
0
1
1
1 1 x= 1
1 0 0 1
= (same L)
b
3
gives x = 0
1
1
0
a
a
c.
cj
d
a
a
a
a
a
1
1
0
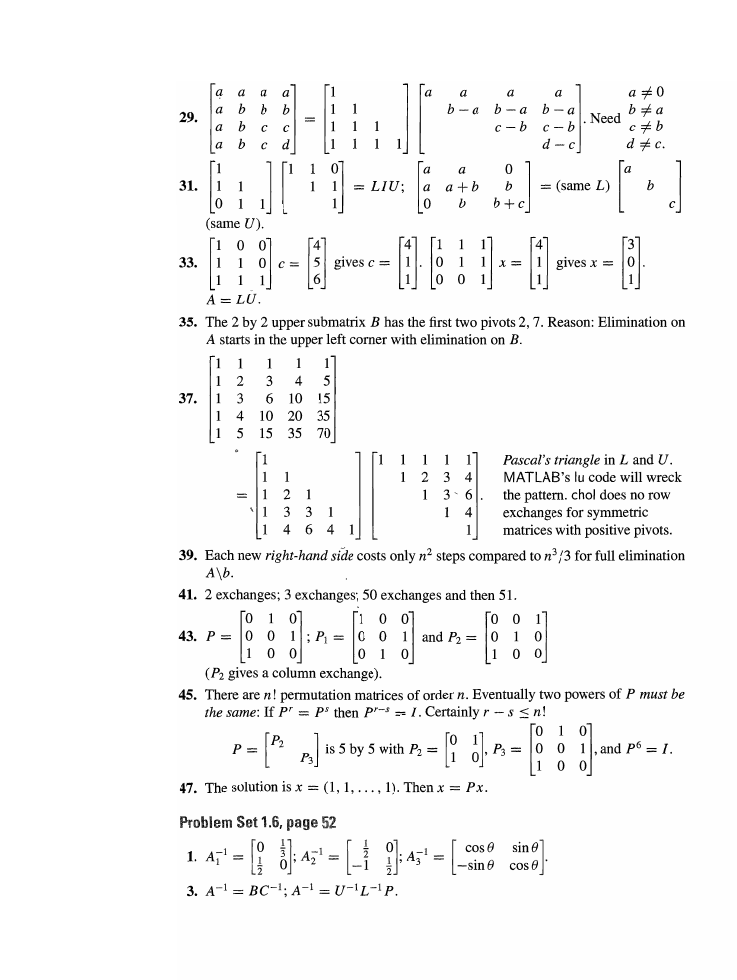
1
1
29.
31.
33.
35.
37.
A = LU.
The 2 by 2 upper submatrix B has the first two pivots 2, 7. Reason: Elimination on
A starts in the upper left corner with elimination on B.
R
D
C
1
1
1
1
1
,
-
,
1
2
3
4
5
1
3
6
10
15
1
1
1
1
.
-
1
a
'
.
-
-
+
1
4
10
20
35
1
5
15
35
70
Fl
1
1
1
2
1
1
2
3
4
1
3
1
6 4
1
1
l
4
3
3, 61
4
1
1
Pascal's triangle in L and U.
MATLAB's lu code will wreck
the pattern. chol does no row
exchanges for symmetric
matrices with positive pivots.
39. Each new right-hand side costs only n2 steps compared to n3/3 for full elimination
v
.
,
A\b.
41. 2 exchanges; 3 exchanges; 50 exchanges and then 51.
0
1
43. P= 0 0
0
1
0
1
0
0
o
1
; P1 = 0 0
o
m
0
1
0
0
0
1 and P2 = 0 1
0
0
1
1
0
0
(P2 gives a column exchange).
45. There are n! permutation matrices of order n. Eventually two powers of P must be
G
1
.
the same: If Pr = Ps then Pr-s = I. Certainly r - s < n!
P= [P2
0
N
.
LLL
l
P3J
is 5 by 5 with P2 =
0
.
N
r
L1
L
]'3=[? 0 1 andP6=l.
0
0
010
47. The solution is x = (1, 1, ... , 1). Then x = Px.
Problem Set 1.6, page 52
0
1. A11 = 2
3. A-1 = BL
A21
1
0 '
C-1; A-1 = U-1L-1 P.
0
1 A31' [-sin6 cos8'
sin 01
cos 8
_
434
Solutions to Selected Exercises
5.
7.
A(AB) = (move parentheses) = (A2)(B) = I.
1/2
1/2
-!/2 1/2
1/2
/3/21' [1 0all have A2 = I.
0
1
9. If row 3 of A-1 were (a, b, c, d), then A-1 A = I would give 2a = 0, a + 3b = 0,
4a +f 8b = 1. This has no solution.
11. (a)
+
-
M
10 01l 1+ 0
10
01
01
(b)
[0
0
0
0
] + [0
01] - [0
1]
-Oll
1 _
0
-1 0] - -1 1
1
1
(B-' + A-')-' = B(A + B)-'A.
6
13. ATB = 8; BTA = 8; ABT = [2 2]; BAT = [6
15. (a) n(n + 1)/2 entries on and above diagonal.
p
(
'
2
2]°
(b) (n - 1)n/2 entries above
diagonal.
17. (a) The inverse of a lower (upper) triangular matrix is still lower (upper) triangu-
lar. Multiplying lower (upper) triangular matrices gives a lower (upper) triangular
(b) The main diagonals of Li 1 L2 D2 and D1 U, U2 1 are the same as those
matrix.
of D2 and D1 respectively. L- 1L2D2 = D1 U,U2so we have D1 = D2. By com-
paring the off-diagonals of L i1 L2 D2 = D1 U1 U2both matrices must be diagonal.
Li1L2D2 = D2, D1U1U2 1 = Dl, D, is invertible so L11L2 = I, U,UU l = I.
Then L, = L2, U1 = U2-
19.
1
3
5
0
1
1
0
0
1
1
0
0
0
3
0
0
0
2
1
0
0
5
3
1 1;
0
1 b/a
1
1
[b/a
a
0
11 [0 d - (b2/a)] [0
0
] -
1
LDLT.
21. From B(I - AB) = (I - BA)B we get (I - BA)-1 = B(I - AB)-1B-1, an
explicit inverse provided B and I - AB are invertible. Second approach: If I - BA
is not invertible, then BAx = x for some nonzero x. Therefore ABAx = Ax, or
ABy = y, and I - AB could not be invertible. (Note that y = Ax is nonzero, from
BAx = x.)
23.
25.
[z] °
.1]
so A-1 = 0 2
.2]'
[y]
(a) In Ax = (1, 0, 0), equation 1 + equation 2 - equation 3 is 0 = 1.
right-hand sides must satisfy b, + b2 = b3.
third pivot.
(b) The
(c) Row 3 becomes a row of zeros-no
1].
27. If B exchanges rows 1 and 2 of A, then B-1 exchanges columns 1 and 2 of A-'.
29. If A has a column of zeros, so does BA. So BA = I is impossible. There is no A-1.
F1
[ I
31.
+
-
W
F
-1
1
1r1
1JI
L 1
1
1J
1
lrl
rl
rl
i
1] =[ 0 -1
1
= E;
1]
then 1
1
1
1
1
is L = E-',after reversing the order of these 3 elementary matrices
and changing -1 to +1.
Solutions to Selected Exercises
435
33. A * ones(4,1) gives the zero vector, so A cannot be invertible.
35.
37.
1
2
[
+
-
r
[3
I
0
0
3
7
1
0
8
0
a
1
0
b
c
1
0
1
01]
1
0
0
39.
[
2
0
2
2
0
1
1
0
[
2
0
1
0
3
1
1
0
-2 1
O
l -
2
[0
-
1]
01
[0
8
o
-3 -1]
o
w
1 0 - 0 1
0 0
a
1
0
1
0
1
0
0
0
0
1
0
0 -
1 O]
0
0
1
0
0
1
1 0 -b
-c
0
0
"
1
-
r
0
1
1 -a ac-b
-c
0
0
1
0
0 -
[o
1
1
1/2
0
= [I A-']
.
1
43. A-1 =
1
0
0
LO
diagonal.
I0],
45.
[-C
41. Not invertible for c = 7 (equal columns), c = 2 (equal rows), c = 0 (zero column).
1 OH 01
0
1
1
1
0
1
0
0
. The 5 by 5 A-1 also has is on the diagonal and super-
[-DACA-1 D 1]>
and [-D
47. For Ax = b with A = ones(4, 4) = singular matrix and b = ones(4, 1), A\b
will pick x = (1, 0, 0, 0) and pinv(A) * b will pick the shortest solution x =
(1, 1, 1, 1)/4.
01
49. AT = [0 3], A-1 = [_1
1/3], (A'-')T = (AT)-1 = [1
-3]' AT = A and
then A-1 = c [O
_1] = (A-')T = (AT)-1.
51.
((AB)-1)'r = (B`'A-I)T = (A-1)T(B-I)T; (U`1)T is lower triangular.
53. (a) xTAy = a22 = 5.
(b) xT A = [4
5
6].
(c) Ay = [].
55. (Px)T(Py) = xT PT Py = xTy because pTp = I ; usually Px y = X. PT y
0
0
1
1
0
0
0
1
0
1
2
3
1
2
1,
21
3
0
0
1
1
0
0
0
1
0
1
1
2
57. PAPT recovers the symmetry.
59. (a) The transpose of RTAR is RTATRTT = RTAR = n by n.
(b) (RTR)jJ = (column j of R) (column j of R) = length squared of column j.
相关推荐
2023年江西萍乡中考道德与法治真题及答案.doc
2012年重庆南川中考生物真题及答案.doc
2013年江西师范大学地理学综合及文艺理论基础考研真题.doc
2020年四川甘孜小升初语文真题及答案I卷.doc
2020年注册岩土工程师专业基础考试真题及答案.doc
2023-2024学年福建省厦门市九年级上学期数学月考试题及答案.doc
2021-2022学年辽宁省沈阳市大东区九年级上学期语文期末试题及答案.doc
2022-2023学年北京东城区初三第一学期物理期末试卷及答案.doc
2018上半年江西教师资格初中地理学科知识与教学能力真题及答案.doc
2012年河北国家公务员申论考试真题及答案-省级.doc
2020-2021学年江苏省扬州市江都区邵樊片九年级上学期数学第一次质量检测试题及答案.doc
2022下半年黑龙江教师资格证中学综合素质真题及答案.doc
