湍流数值模拟.pdf

发布时间：2022-06-01 发布人：admin 分类：说明书资料大小：4.61M 资料格式：pdf 举报版权申诉

upjzk-7857901-4744302542950809307.pdf-第1页.png

第1页 / 共67页

upjzk-7857901-4744302542950809307.pdf-第2页.png

第2页 / 共67页

upjzk-7857901-4744302542950809307.pdf-第3页.png

第3页 / 共67页

upjzk-7857901-4744302542950809307.pdf-第4页.png

第4页 / 共67页

upjzk-7857901-4744302542950809307.pdf-第5页.png

第5页 / 共67页

upjzk-7857901-4744302542950809307.pdf-第6页.png

第6页 / 共67页

upjzk-7857901-4744302542950809307.pdf-第7页.png

第7页 / 共67页

upjzk-7857901-4744302542950809307.pdf-第8页.png

第8页 / 共67页

文本预览

Simulation of Turbulent Flows - LES & DES with FLUENT Sung-Eun Kim Principal Engineer June 9, 2004

Agenda What is LES? Why LES? What about the cost? What does FLUENT offer? Best-practice LES with FLUENT Closing Remarks

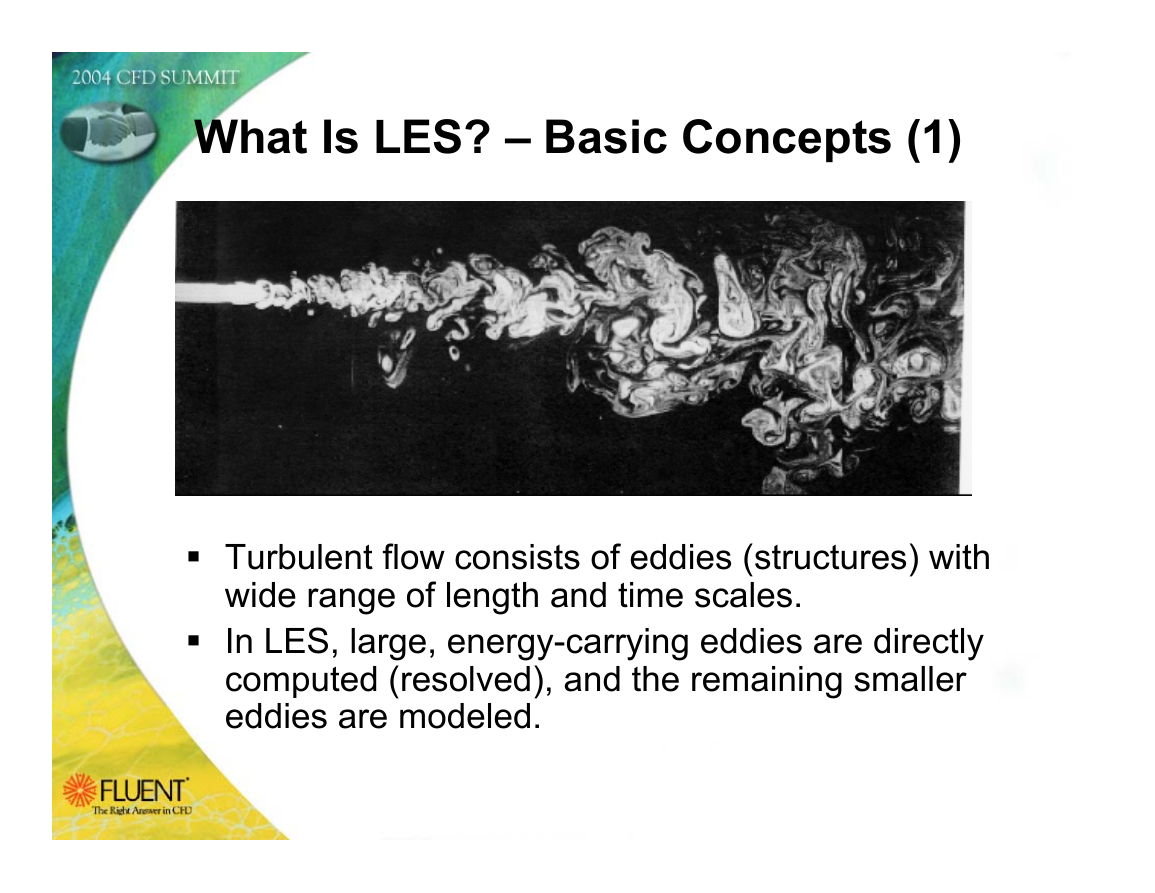
What Is LES? – Basic Concepts (1) Turbulent flow consists of eddies (structures) with wide range of length and time scales. In LES, large, energy-carrying eddies are directly computed (resolved), and the remaining smaller eddies are modeled.

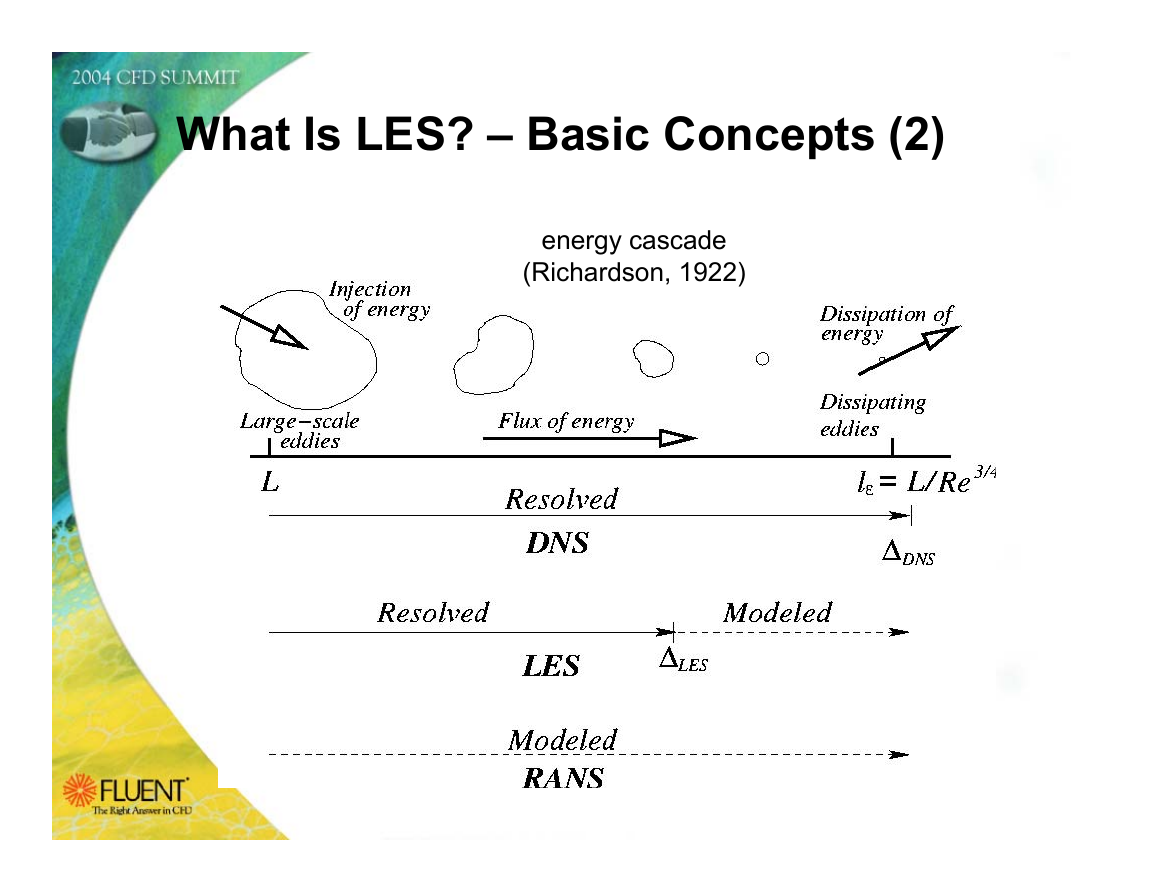
What Is LES? – Basic Concepts (2) energy cascade (Richardson, 1922)

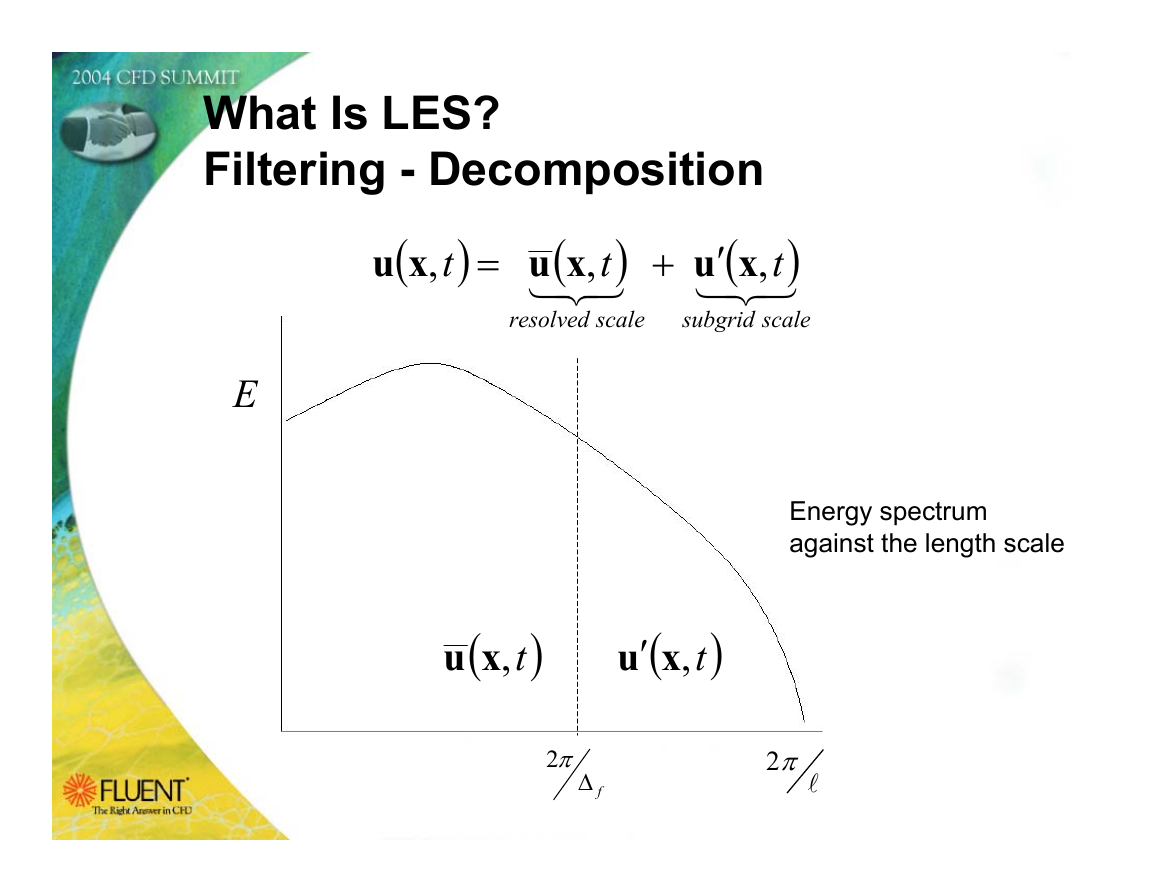
What Is LES? Filtering - Decomposition ( ( ) xu xu t t , , ′+ 321321 subgrid scale resolved scale ( xu , = ) t ) E Energy spectrum against the length scale ( )t,xu ( )t,xu′ π2 f∆ π2 l

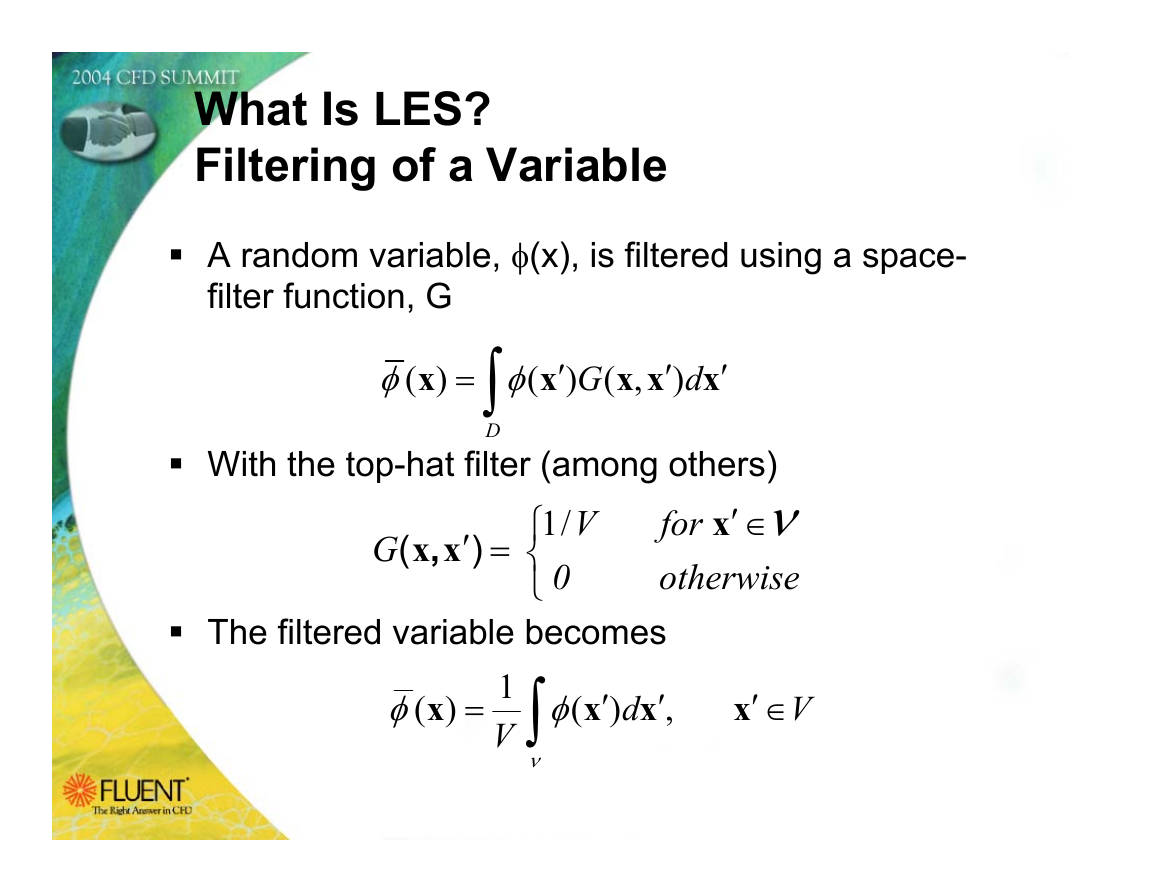
What Is LES? Filtering of a Variable A random variable, φ(x), is filtered using a space- filter function, G φ x )( = ( φ x G ) ′ xx ) ,( ′ x d ′ ∫ D With the top-hat filter (among others) ν for ∈′ otherwise V /1  ) =′  0  The filtered variable becomes , xx x G ( φ x )( = 1 V ∫ ν ( φ x ) ′ d x x , ′ ∈′ V

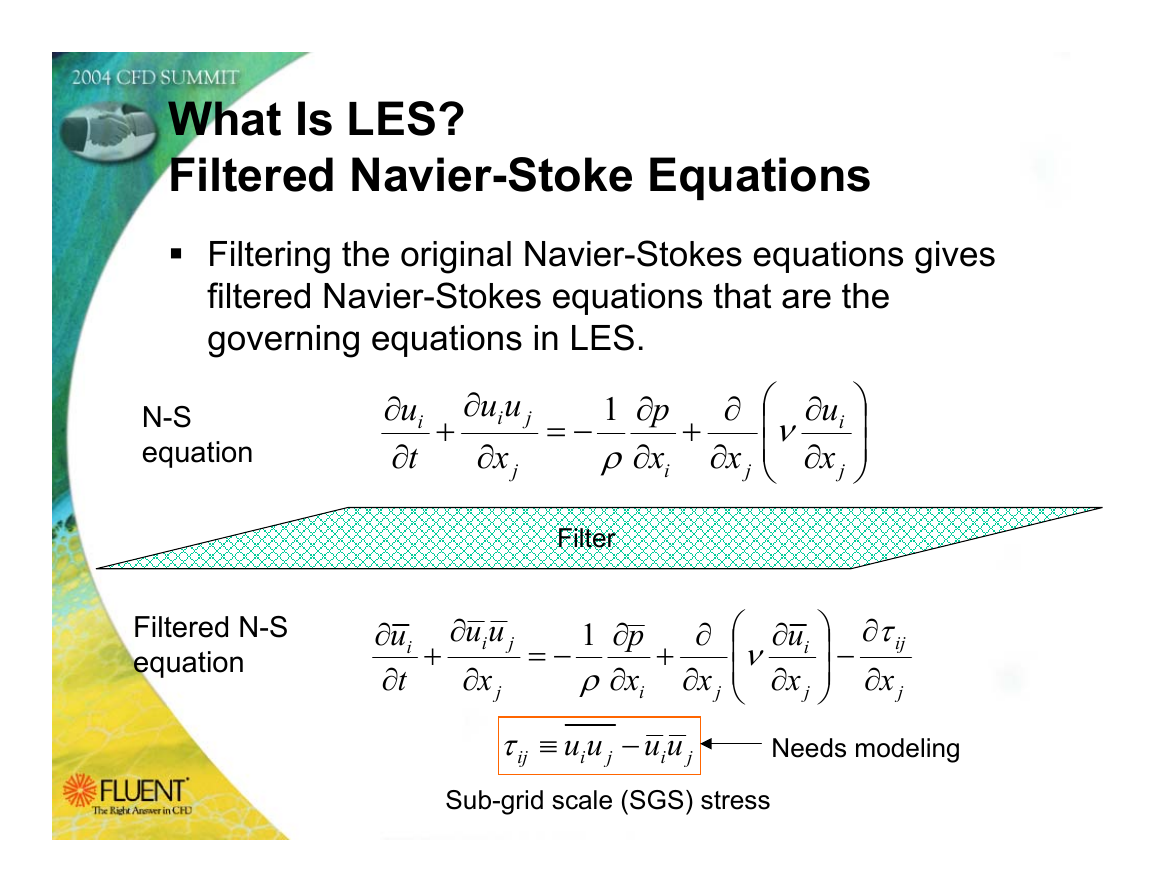
What Is LES? Filtered Navier-Stoke Equations Filtering the original Navier-Stokes equations gives filtered Navier-Stokes equations that are the governing equations in LES. N-S equation i u ∂ t ∂ + j uu ∂ i x ∂ j −= 1 ρ p ∂ x ∂ i + ∂ x ∂ j  u ∂  ν  x ∂  i j     Filter Filtered N-S equation i u ∂ t ∂ + j uu ∂ i x ∂ j −= 1 ρ ∂ x ∂   ν   u ∂ x ∂ i j   −  ∂ τ ij x ∂ j j + p ∂ x ∂ i uu i − ≡τ ij uu i j j Needs modeling Sub-grid scale (SGS) stress

Agenda What is LES? Why LES? What about the cost? What does FLUENT offer? Best-practice LES with FLUENT Closing Remarks

分享到：

赞收藏

资料库

湍流数值模拟.pdf

相关推荐

行业

热门标签

最新资料