第1页 / 共7页
第2页 / 共7页
第3页 / 共7页
第4页 / 共7页
第5页 / 共7页
第6页 / 共7页
第7页 / 共7页
人工超表面用于全息漏波天线.pdf
Title
Figure 1
Figure 2
Figure 3
Figure 4
Table 1 Detailed scanning properties for one of the four-beam radiations
Figure 5 The simulation results of the four-beam 2D frequency scanning, in which the radiation patterns are scanned in both azimuth and elevation directions with the change of operating frequencies.
Figure 6
References
Table 2 The detailed parameters of double-beam scanning in theoretical analysis
OPEN
SUBJECT AREAS:
APPLIED PHYSICS
MATERIALS FOR DEVICES
Received
10 September 2014
Accepted
16 October 2014
Published
5 November 2014
Correspondence and
requests for materials
should be addressed to
T.J.C. (tjcui@seu.edu.
cn)
Frequency-Controls of Electromagnetic
Multi-Beam Scanning by Metasurfaces
Yun Bo Li, Xiang Wan, Ben Geng Cai, Qiang Cheng & Tie Jun Cui
State Key Laboratory of Millimeter Waves, School of Information Science and Engineering, Southeast University, Nanjing 210096,
China.
We propose a method to control electromagnetic (EM) radiations by holographic metasurfaces, including to
producing multi-beam scanning in one dimension (1D) and two dimensions (2D) with the change of
frequency. The metasurfaces are composed of subwavelength metallic patches on grounded dielectric
substrate. We present a combined theory of holography and leaky wave to realize the multi-beam radiations
by exciting the surface interference patterns, which are generated by interference between the excitation
source and required radiation waves. As the frequency changes, we show that the main lobes of EM radiation
beams could accomplish 1D or 2D scans regularly by using the proposed holographic metasurfaces shaped
with different interference patterns. This is the first time to realize 2D scans of antennas by changing the
frequency. Full-wave simulations and experimental results validate the proposed theory and confirm the
corresponding physical phenomena.
I n recent years, it has been shown that extreme controls of electromagnetic (EM) waves can be achieved by
using metasurfaces. Due to advantages of smaller physical space and less lossy structures than bulk metama-
terial1–6, metasurfaces have found great potential applications in both microwave and optical frequencies.
Similar to metamaterials, the metasurfaces are also modeled as sub-wavelength textures and several analysis
methods have been established. Typically, the surface impedance or effective surface refractive index can be
calculated analytically using the transverse resonance approach7, from which surface-wave waveguides8 and
lenses9–11 have been designed. To reduce the computational time in eigen-mode simulations, a fast method
was presented to calculate the surface impedance efficiently12. Later, the printed-circuit tensor impedance sur-
face13 has been analyzed using the modified transverse resonance technique and idealized tensor impedance
boundary condition. Another method to model metasurface or metafilm is the generalized sheet transition
condition14, in which the small scatterers are characterized as electric and magnetic polarization densities.
Based on this method, reflection and transmission coefficients of metafilms or metasurfaces have been
obtained15–16, promoting the study of meta-transmission arrays17–19. To control EM waves with more flexibility,
the generalized Snell’s law was proposed20 by introducing the abrupt phases on the interface of the two media.
Based on similar ideas, a gradient-index metasurface was presented to convert propagating waves to surface waves
efficiently21 and convert the circularly-polarized light to cross-polarized light22,23.
On the other hand, due to the low profile and low loss, metasurfaces have great advantages in the design of
surface aperture antennas. Based on the original analysis of sinusoidally-modulated reactance surface24, the
holographic antenna composed of surface impedance was proposed25, which shapes the monopole source as a
pencil beam in the far-field region and changes the linearly-polarized source to circularly-polarized radiation by
using anisotropic surface impedance units. Later, a spiral leaky-wave antenna26 based on the modulated isotropic
surface impedance implemented by corrugated dielectric or metallic patches on a grounded substrate can
generate circularly polarized waves. Then the anisotropic tensor surface impedance formed by isotropic
textures was used to realize circularly-polarized isoflux radiations27 for space-to-ground data link applications
in the X-band. In study of microwave imaging, a concept of metamaterial aperture has been presented for
computational imaging28–29, in which the random-aperture leaky-wave antennas composed of complementary
electric-inductor-capacitor elements have been used to generate multi- beam radiation patterns. The metasurface
antennas can also be tunable30–31 and conformal32–33 so that they are more functional and practical in many
engineering applications.
In this work, we propose to control extreme EM radiations by using holographic metasurfaces, which can
radiate multiple beams with the frequency sweeping in one dimension (1D) or two dimensions (2D) without
using the complicated beam-forming network34 and anisotropic structures35–36. The proposed metasurfaces are
composed of sub-wavelength quasi-periodic metallic patches on a grounded dielectric substrate, which can be
SCIENTIFIC REPORTS | 4 : 6921 | DOI: 10.1038/srep06921
1
shaped by mapping the variation of patch gaps to the surface imped-
ance under the particular holographic interference. Once the interfer-
ence pattern is recorded by the interference between reference waves
and multi-beam radiations on the metasurface aperture, according to
microwave holography theory, the metasurface excited by the reference
waves generated by a monopole antenna can reproduce the multiple
beams as we desire. We demonstrate that the radiation waves generated
by the holographic metasurface can accomplish 2D scans regularly
with the change of frequency, which has not been realized in the earlier
holographic metasurfaces and leaky-wave antennas.
Results
Theory and analysis. The surface impedance is defined as the ratio of
electric to magnetic fields near a surface. Hence, the perfectly electric
conducting (PEC) and perfectly magnetic conducting (PMC) surfaces
are physically
surfaces,
respectively. We can design a metasurface by forming quasi-periodic
gradient distribution of surface impedance. According to microwave
holography theory, the desired radiation beam can be produced by
using a reference wave to excite holographic interferogram, which is
generated by the interference between the reference wave and radiation
wave. The interferogram equation is given as37
and high-impedance
zero-impedance
Y~ jyref
zyobjj
ð1Þ
ð2Þ
in which Yref is the reference wave and Yobj is the object wave. To
design the surface-aperture radiations, we use the distribution of
surface impedance to embody the whole surface interferogram. We
rewrite Eq. (1) to describe the distribution of surface impedance as
Z~j ½XzM Re(y
ref yobj)
where X and M indicate the average value of surface impedance and the
modulation depth, respectively, and ‘‘*’’ represents the conjugate
operation. To build up the object wave (or radiation wave), we
should use the reference wave to excite the interference pattern in
determining (y
~yobjjyrefj2.
ref yobj)yref
In our design, the basic unit of metasurface is a sub-wavelength
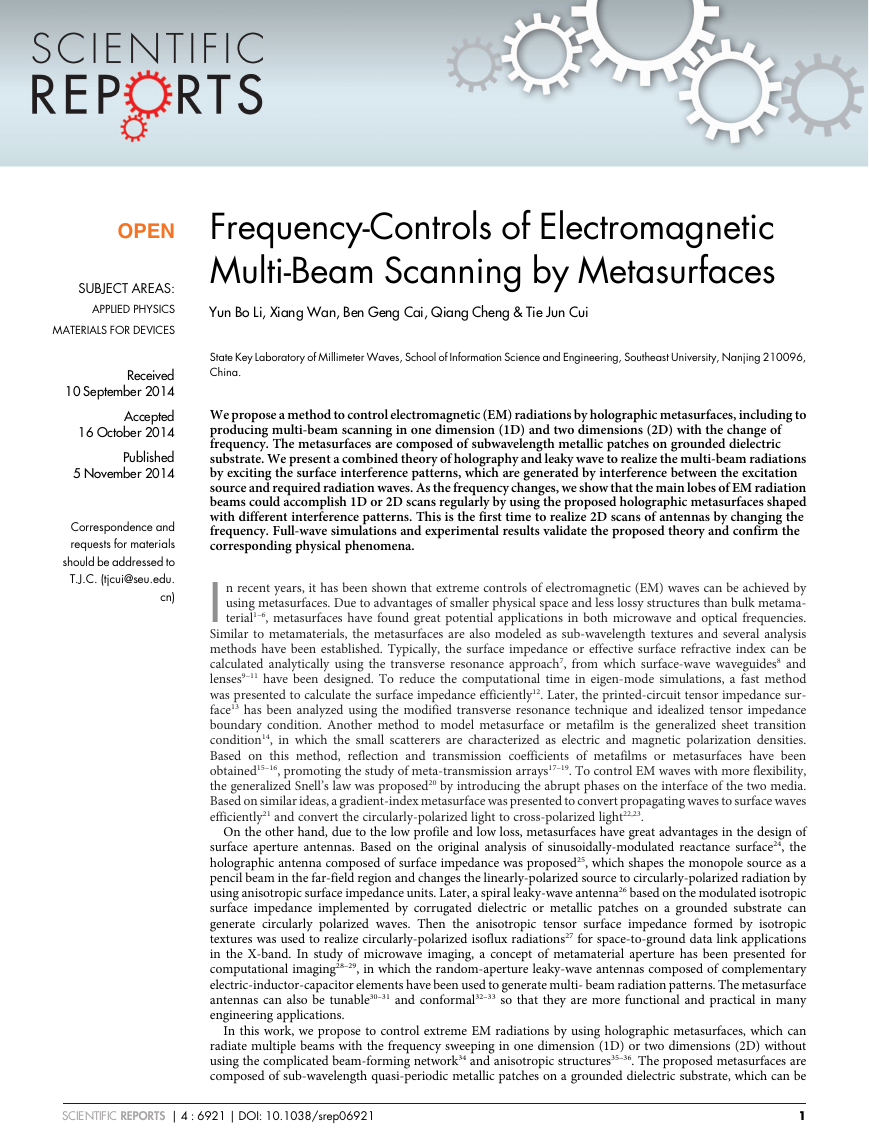
metallic patch on a grounded substrate, as shown in Fig. 1(a). We
extract the surface impedance of the metasurface via its dispersion
curve. When the surface-wave vector kt passes through a unit cell, the
phase difference across the unit is w 5 kta, which can be acquired
under eigen-mode simulations, where a is the period of the unit cell.
www.nature.com/scientificreports
Then the surface refractive index is given as n 5 c/vt 5 ktc/vt, where c
is the light speed in free space and vt is the surface angular frequency.
Combining with the equation of surface impedance Z 5 jZ0kz/k of
the transverse-magnetic (TM) modes, we obtain the relation between
the unit phase difference and surface impedance as
q
ffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffi
1{w2c2=a2v2
Zs~Z0
ð3Þ
where Z0 is the impedance in free space. Therefore, once the unit
phase difference is acquired through the eigen-mode simulation, we
can calculate the surface impedance. The gap between square patches
(see the orange area in Fig. 1(a)) determines the value of surface
impedance. When slowly varying the gap sizes to obtain different
surface impedances, the structure is considered as quasi-periodic.
From the nine dispersion curves with changing gap sizes shown in
Fig. 1(b), we calculate nine values of surface impedance at 17 GHz,
from which the following equation is fitted using cubic polynomials
to describe the relationship between surface impedance and gap size:
g~{1:2763:10({9):Z3
s
z8:509:10({7):Z2
s
{
1:9586:10({4):Zsz0:0158
ð4Þ
Then we determine the values of X and M as 197.5 and 36.5, respect-
ively, from the simulated values of surface impedance.
According to the holographic antenna theory, once the interfer-
ence pattern is recorded by the interaction between reference wave
and multi-beam radiation wave on the metasurface, we can recon-
struct the multiple beams as we desire. We choose a monopole
antenna placed in the center of metasurface to produce the reference
wave, which is written as
yref
~ e{jk0n r
ð5Þ
where n is the effective surface refractive index and r is the distance
from the origin to the surface radiation unit. For double-beam radi-
ation, the object wave (i.e. the desired radiation wave) can be defined as
~ e{j~k1~r ze{j~k2 ~r
yobj
~e{j(xk0 sin h1 cos w1zyk0 sin h1 sin w1)ze{j(xk0 sin h2 cos w2zyk0 sin h2 sin w2)
ð6Þ
in which ~k1 and ~k2 are wave-number vectors of the two beams with the
I is the
elevation and azimuth angles h1/2 and w1/2, respectively, and r
Figure 1 | (a) Structure of the metallic patch on a grounded dielectric substrate, in which the blue and orange areas are dielectric and metal. The period of
square lattice is a53 mm, and the gap size varies from 0.2 to 1.0 mm. F4B is chosen as the dielectric substrate with the relative permittivity 2.2 and
the thickness 1.57 mm. (b) The dispersion curves of unit cells with different gap sizes. The black oblique line is the light line.
SCIENTIFIC REPORTS | 4 : 6921 | DOI: 10.1038/srep06921
2
spatial location vector. In particular, when h1 5 h2 5 30u and Q1 5 45u,
ffiffi
Q2 5 135u, Eq. (6) is rewritten as
p
2
4 k0(x zy)zej
ffiffi
p
2
4 k0(xzy)
~ e{j
ð7Þ
yobj
in which the positive and negative exponential terms indicate the
radiation waves propagating to the two sides of the normal direction
on the metasurface. To obtain the double-beam radiation shaped by
Eq. (7), the interferogram generated by Eq. (2) should be excited by the
reference wave defined in Eq. (5).
We will show later that the proposed holographic metasurface is
equivalent to the 2D leaky-wave structure. To radiate EM waves more
efficiently, we define the ‘‘-1’’ order leaky-wave radiation without other
Floquet modes participating the interference by synthetically consider-
ing the unit design and radiation frequency. In fact, the forward and
backward waves in leaky waves mean that the radiation directions are
uniform and non-uniform with the propagation of surface waves. If we
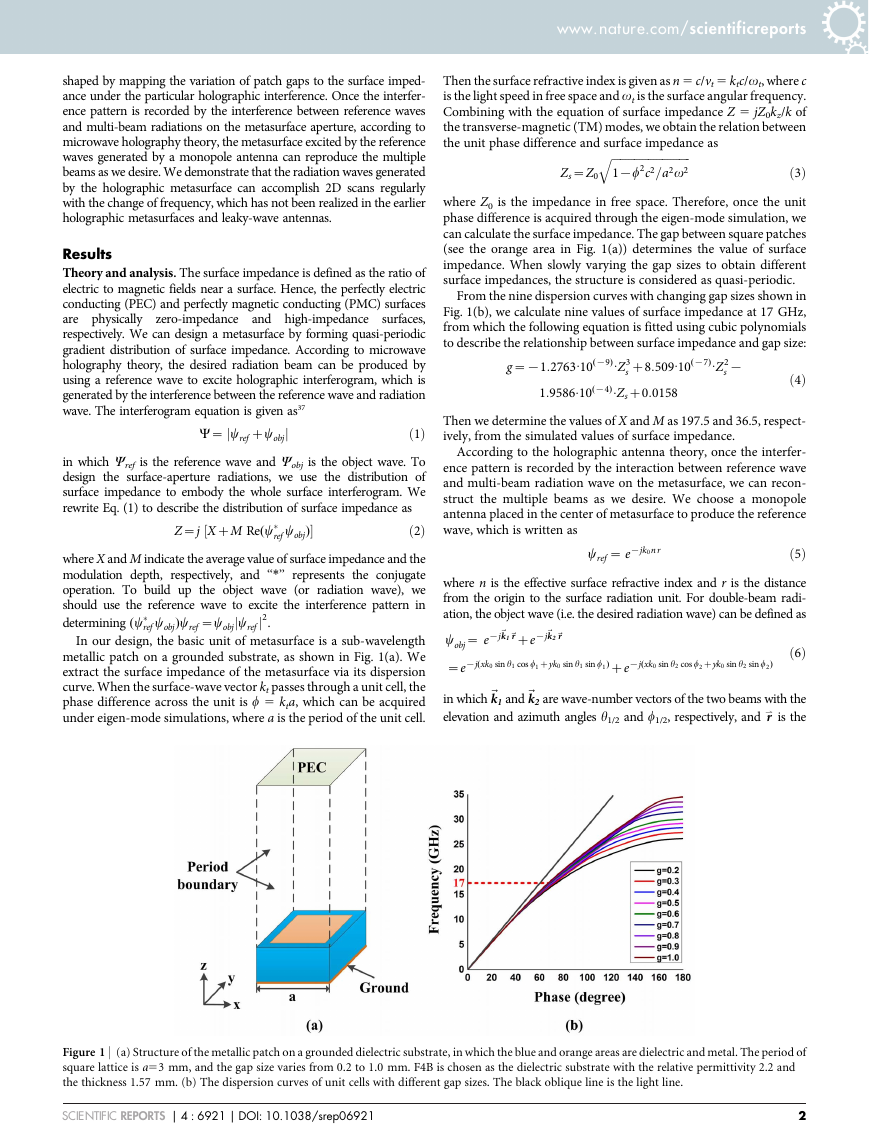
place the monopole antenna in the center of metasurface as excitation,
the full-wave simulation results reveal that the leaky-wave (object-
wave) radiation pattern may generate the sag like ‘‘rabbit’s ears’’ in
the far fields, as shown in Fig. 2(a). This phenomenon is caused by
the slightly radiating deviation of forward and backward modes, which
is attributed to the inaccuracy of effective surface refractive index that
determines the distribution of surface currents. The surface currents
excited by the monopole antenna will not be completely the same as
the reference wave so that the forward and backward waves have
different radiation directions, as illustrated in Fig. 2(a).
The optimization of surface refractive index n can avoid the ‘‘rab-
bit’s ear’’ phenomenon by enforcing h1 5 h2 in Fig. 2(a), but it is more
complicated. Here, we present a side-feeding method, as shown in
Fig. 2(b). The interferogram shaped by the holographic metasurface
is generated by Eq. (2), which is combined with Eq. (4) to determine
the relation to describe the gap size versus position on the metasur-
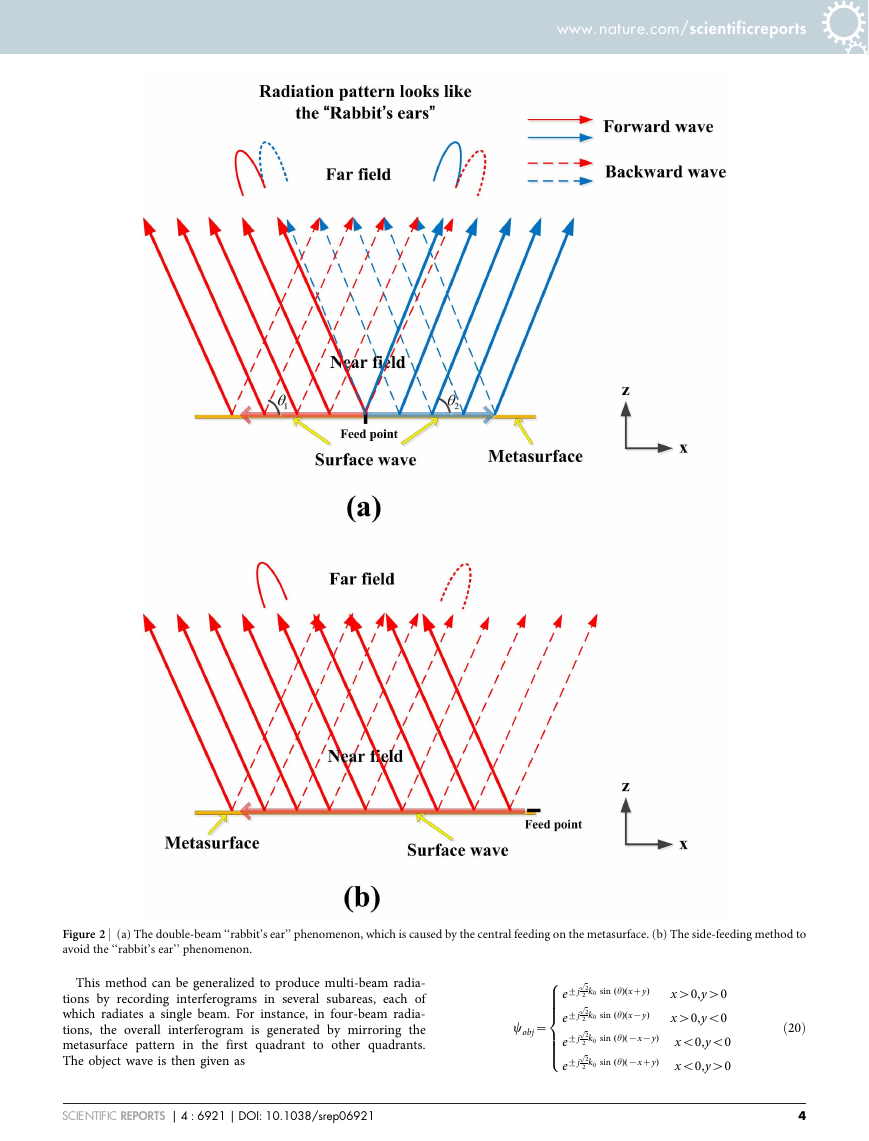
face. Fig. 3(a) illustrates the interferogram generated by the interfer-
ence between double-beam radiation (Eq. (7)) and reference (Eq. (5))
waves at 17 GHz. Based on the full-wave simulations by commercial
software, CST Microwave Studio, the reproduced waves are demon-
strated in Fig. 3(c). We also show that the double-beam radiations
can make 1D scan with the change of frequency (16–18 GHz), as
clearly observed in Figs. 3(b) – (d).
To explain the phenomenon of frequency scanning by the 2D meta-
surface, we now show the equivalence of the holographic reproduction
and ‘‘-1’’-order leaky-wave radiation. For simplicity, we analyze a sin-
gle-beam radiation defined by Yobj 5 e2jkxsin(h), which will interference
with reference wave Yref 5 e2jknx to generate the interferogram as
ref yobj)~j½XzM cos (k0nx{k0x sin (h))
Z~j ½XzMRe(y
ð8Þ
To obtain periodicity of the holographic distribution of surface imped-
ance along the x direction, we define k0nx-k0x sin(h)5 2p, and the
corresponding period is determined as
2p
ð9Þ
a~
k0n{k0 sin (h)
www.nature.com/scientificreports
where k9, n9 and h9 are the same as those in Eq. (8) but under different
operating frequencies.
We introduce the concept of surface-wave phase gradient to
explain the above phenomenon. For simplicity, we still analyze the
~ e{j sin (h)k0½x cos (Q) zy sin (Q)
single-beam radiation defined by yobj
and yref
~ e{jk0nr, which generate the interferogram as
ð12Þ
ref yobj)
Z~j ½XzMRe(y
~j ½XzM cos½k0nr{ sin (h)k0½x cos (Q)zy sin (Q)
Here, we assume that the sinusoidal phase distribution (Re(y
ref yobj))
of interferogram generated by the impedance units is approximately
invariant by changing frequencies since the higher- and lower-imped-
ance areas of the interferogram are fixed. Hence we only need to
concern the object wave term in processing the holographic reproduc-
tion. If we excite the interferogram by reference wave defined as
r, where k9 and n9are the wave number in free space
y
and effective refractive index, then the phase of surface-wave front
ref (y
ref yobj) becomes W 5 k9n9r2k0nr 1 sin(h)k0[x cos(Q) 1 y
y
sin(Q)]. By calculations, the surface-wave phase gradient =W is given as
+W~+½k
~(k
r{k0nrzk0x sin (h) cos (Q)zk0y sin (h) sin (Q)
{k0n)^rzk0 sin (h) cos (Q)^xzk0 sin (h) sin (Q)^y
0
~ e{jk
ð13Þ
n
0
0
ref
n
n
0
0
0
0
0
where ^r, ^x and ^y are unit vectors. Hence the surface wave front
interference
surface waves
is
~ e{jk0 sin (h)½x cos (Q)zy sin (Q), and the phase
y1
of wave front in the x direction is written as
the
{k0n)r and y2
generated
0
~ e{j(k
two
by
of
n
0
Wx~(k
~(k
0
0
0
0
n
n
{k0n)r cos (Q)zk0x sin (h) cos (Q)
{k0n)xzk0x sin (h) cos (Q)
ð14Þ
where Q is the angle between r and x directions. Thus, the x component
of effective surface wave number at the particular frequency is
0
sx
k
0
~k
0
n
{k0nzk0 sin (h) cos (Q)
ð15Þ
which is corresponding to the wave number of the ‘‘-1’’ order leaky-
wave. Likewise, the y component of effective surface wave number at
the particular frequency is
0
sy
k
0
0
n
~k
{k0nzk0 sin (h) sin (Q)
ð16Þ
Thus, the amplitude and azimuth angle of the effective surface wave
number are given as
q
ffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffi
k02
zk02
sy
sx
0
s
k
~
0
w
~ arctan (k
0
0
sy=k
sx)
ð17Þ
ð18Þ
ð19Þ
which can be rewritten as
ection is expressed as
As a consequence, the elevation angle of the main radiation dir-
sin (h)~n{ 2p
k0a
ð10Þ
The above result is exactly the same as that in the ‘‘-1’’-order leaky-
wave radiation12. Here, h is the radiation direction of the holographic
reproduction. It is obvious that the object wave can accomplish the
beam scanning controlled by frequency, and the corresponding radi-
ation direction is given as
0
0
)~ k
0
n
sin (h
{2p=a
k0
0
~n
{ k0(n{ sin (h))
k0
ð11Þ
0
h
~ arcsin (k
0
0
s=k
)
(18),
ffiffi
According to Eq.
if we change the object wave as
p
2
~ e+
2 jk0(x zy) sin (h) (Q 5 45u; the interferogram is defined in
yobj
the area of x.0 and y.0), in which ‘‘1’’ and ‘‘2’’ represent the
backward and forward modes of leaky waves, we find that the azi-
muth angle of main radiation direction will be unchanged with fre-
0
quencies under the circumstance of k
sy, which can accomplish
1D scanning controlled by frequency, as shown in the simulation
results in Fig. 3.
~k
0
sx
SCIENTIFIC REPORTS | 4 : 6921 | DOI: 10.1038/srep06921
3
www.nature.com/scientificreports
Figure 2 | (a) The double-beam ‘‘rabbit’s ear’’ phenomenon, which is caused by the central feeding on the metasurface. (b) The side-feeding method to
avoid the ‘‘rabbit’s ear’’ phenomenon.
8>>>>><
>>>>>:
ffiffi
p
ffiffi
2
e+j
2 k0 sin (h)(xzy)
p
ffiffi
2
e+j
2 k0 sin (h)(x{y)
p
ffiffi
2
e+j
2 k0 sin (h)({x{y)
p
2
e+j
2 k0 sin (h)({xzy)
This method can be generalized to produce multi-beam radia-
tions by recording interferograms in several subareas, each of
which radiates a single beam. For instance, in four-beam radia-
tions, the overall
interferogram is generated by mirroring the
metasurface pattern in the first quadrant to other quadrants.
The object wave is then given as
~
yobj
SCIENTIFIC REPORTS | 4 : 6921 | DOI: 10.1038/srep06921
xw0,yw0
xw0,yv0
xv0,yv0
xv0,yw0
ð20Þ
4
www.nature.com/scientificreports
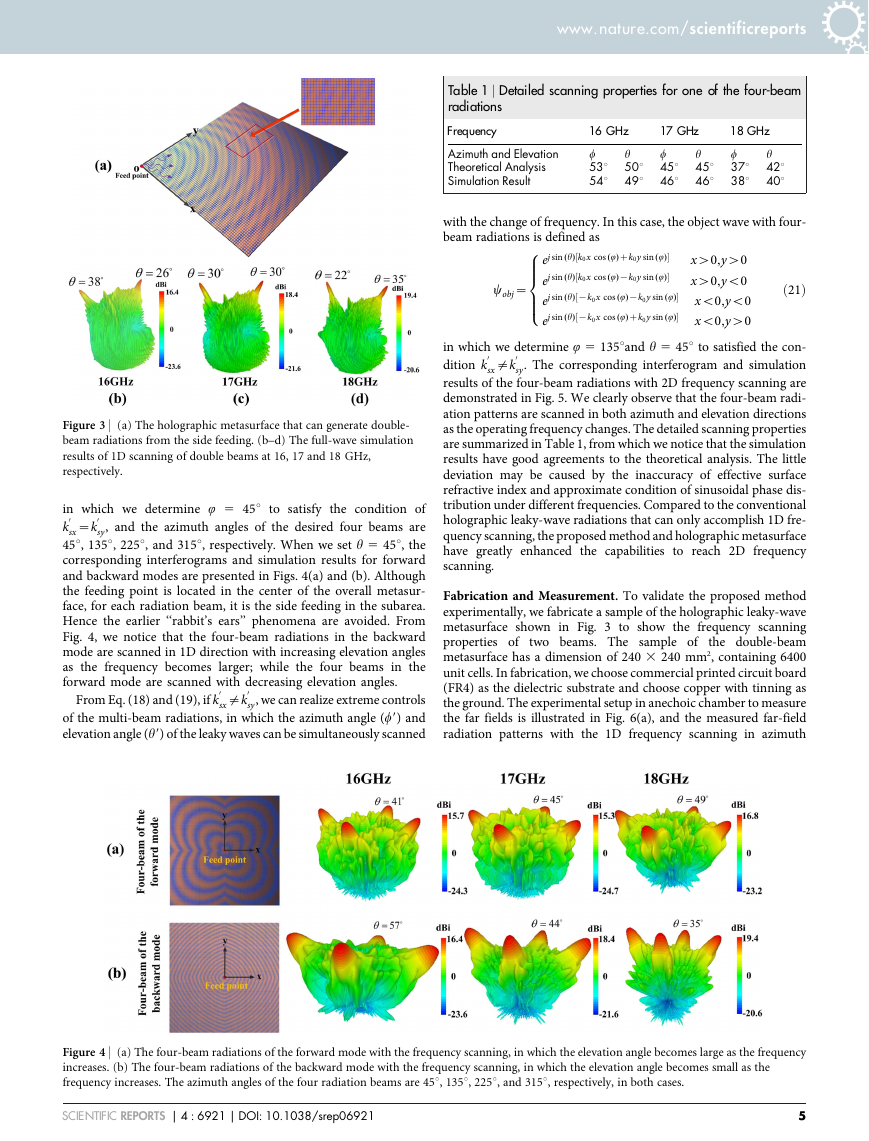
Table 1 | Detailed scanning properties for one of the four-beam
radiations
Frequency
16 GHz
17 GHz
18 GHz
Azimuth and Elevation
Theoretical Analysis
Simulation Result
w
53u
54u
h
50u
49u
w
45u
46u
h
45u
46u
w
37u
38u
h
42u
40u
with the change of frequency. In this case, the object wave with four-
beam radiations is defined as
8>>><
>>>:
~
yobj
ej sin (h)½k0x cos (Q)zk0y sin (Q)
ej sin (h)½k0x cos (Q){k0y sin (Q)
ej sin (h)½{k0x cos (Q){k0y sin (Q)
ej sin (h)½{k0x cos (Q)zk0y sin (Q)
xw0,yw0
xw0,yv0
xv0,yv0
xv0,yw0
ð21Þ
Figure 3 | (a) The holographic metasurface that can generate double-
beam radiations from the side feeding. (b–d) The full-wave simulation
results of 1D scanning of double beams at 16, 17 and 18 GHz,
respectively.
~k
in which we determine Q 5 45u to satisfy the condition of
0
0
sy, and the azimuth angles of the desired four beams are
k
sx
45u, 135u, 225u, and 315u, respectively. When we set h 5 45u, the
corresponding interferograms and simulation results for forward
and backward modes are presented in Figs. 4(a) and (b). Although
the feeding point is located in the center of the overall metasur-
face, for each radiation beam, it is the side feeding in the subarea.
Hence the earlier ‘‘rabbit’s ears’’ phenomena are avoided. From
Fig. 4, we notice that the four-beam radiations in the backward
mode are scanned in 1D direction with increasing elevation angles
as the frequency becomes larger; while the four beams in the
forward mode are scanned with decreasing elevation angles.
From Eq. (18) and (19), if k
0
=k
sy, we can realize extreme controls
of the multi-beam radiations, in which the azimuth angle (w9) and
elevation angle (h9) of the leaky waves can be simultaneously scanned
0
sx
0
sx
=k
in which we determine Q 5 135uand h 5 45u to satisfied the con-
0
dition k
sy. The corresponding interferogram and simulation
results of the four-beam radiations with 2D frequency scanning are
demonstrated in Fig. 5. We clearly observe that the four-beam radi-
ation patterns are scanned in both azimuth and elevation directions
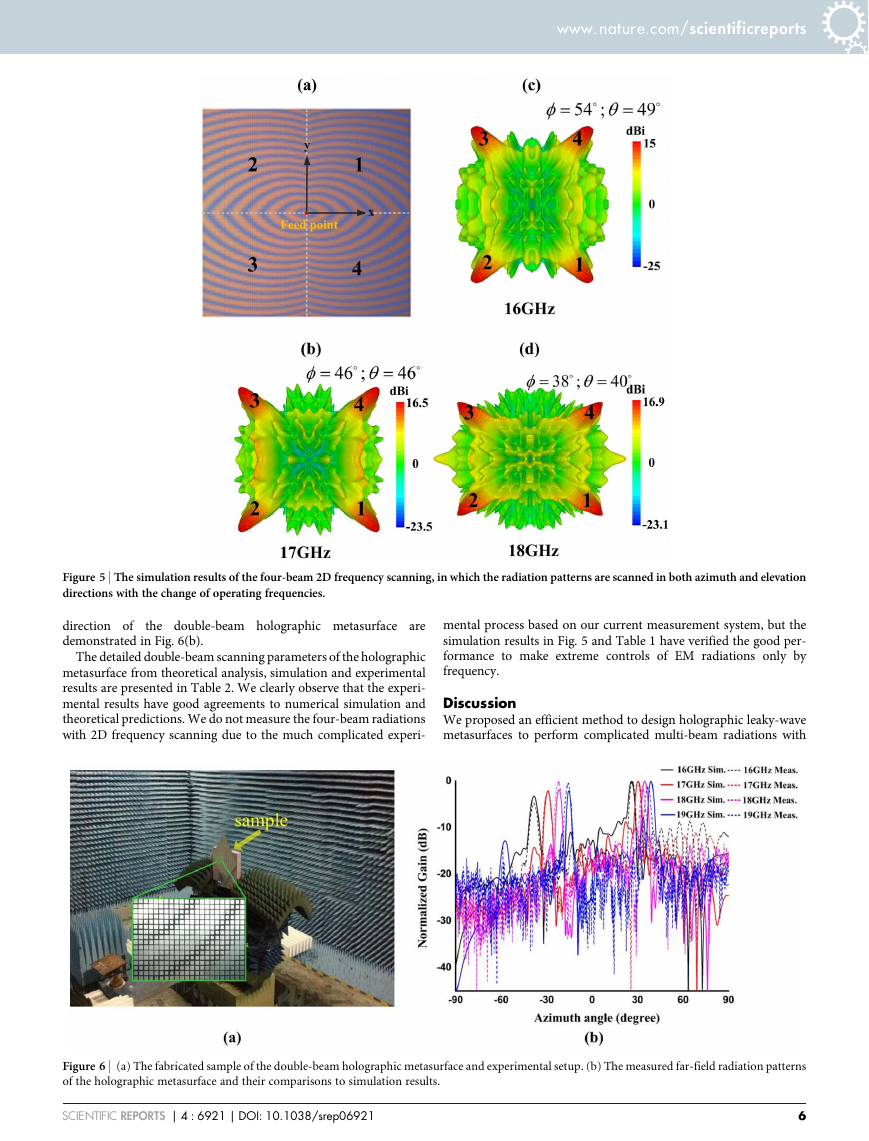
as the operating frequency changes. The detailed scanning properties
are summarized in Table 1, from which we notice that the simulation
results have good agreements to the theoretical analysis. The little
deviation may be caused by the inaccuracy of effective surface
refractive index and approximate condition of sinusoidal phase dis-
tribution under different frequencies. Compared to the conventional
holographic leaky-wave radiations that can only accomplish 1D fre-
quency scanning, the proposed method and holographic metasurface
have greatly enhanced the capabilities to reach 2D frequency
scanning.
two beams. The sample of
Fabrication and Measurement. To validate the proposed method
experimentally, we fabricate a sample of the holographic leaky-wave
metasurface shown in Fig. 3 to show the frequency scanning
properties of
the double-beam
metasurface has a dimension of 240 3 240 mm2, containing 6400
unit cells. In fabrication, we choose commercial printed circuit board
(FR4) as the dielectric substrate and choose copper with tinning as
the ground. The experimental setup in anechoic chamber to measure
the far fields is illustrated in Fig. 6(a), and the measured far-field
radiation patterns with the 1D frequency scanning in azimuth
Figure 4 | (a) The four-beam radiations of the forward mode with the frequency scanning, in which the elevation angle becomes large as the frequency
increases. (b) The four-beam radiations of the backward mode with the frequency scanning, in which the elevation angle becomes small as the
frequency increases. The azimuth angles of the four radiation beams are 45u, 135u, 225u, and 315u, respectively, in both cases.
SCIENTIFIC REPORTS | 4 : 6921 | DOI: 10.1038/srep06921
5
www.nature.com/scientificreports
Figure 5 | The simulation results of the four-beam 2D frequency scanning, in which the radiation patterns are scanned in both azimuth and elevation
directions with the change of operating frequencies.
direction of
demonstrated in Fig. 6(b).
the double-beam holographic metasurface
are
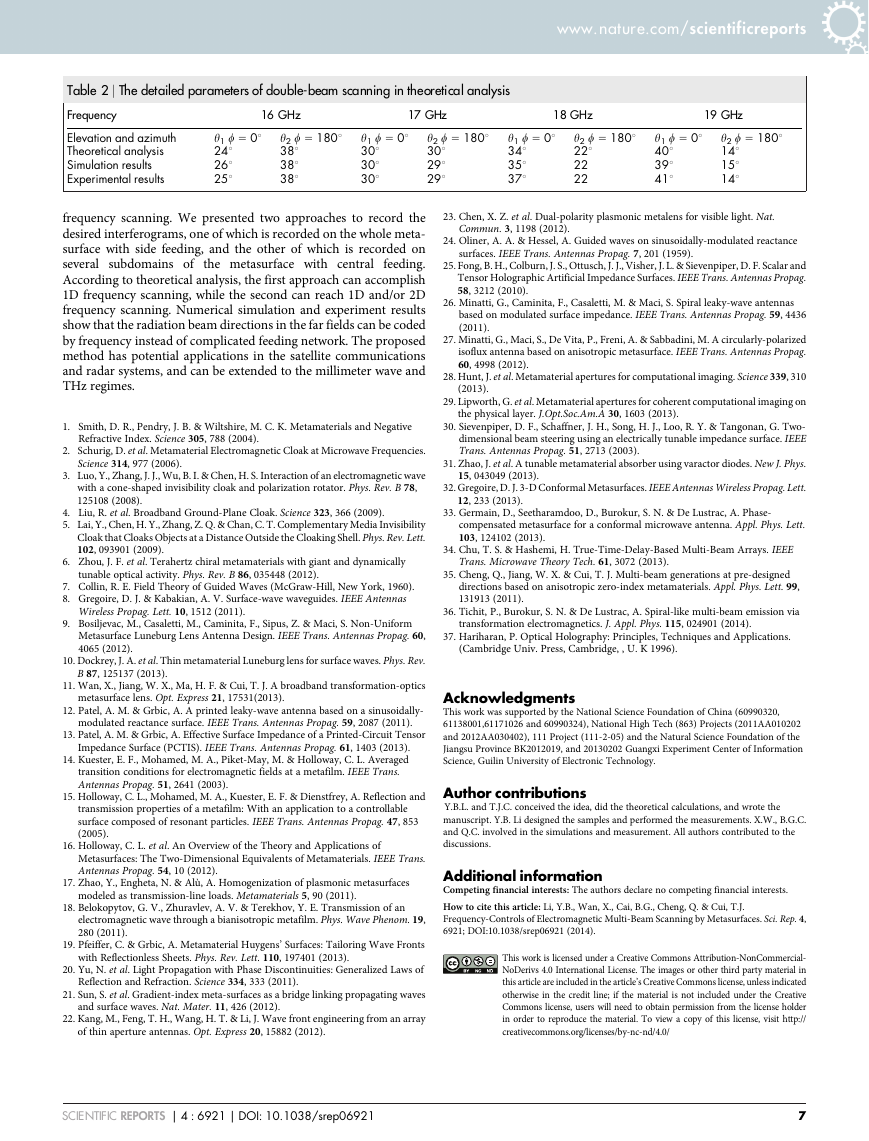
The detailed double-beam scanning parameters of the holographic
metasurface from theoretical analysis, simulation and experimental
results are presented in Table 2. We clearly observe that the experi-
mental results have good agreements to numerical simulation and
theoretical predictions. We do not measure the four-beam radiations
with 2D frequency scanning due to the much complicated experi-
mental process based on our current measurement system, but the
simulation results in Fig. 5 and Table 1 have verified the good per-
formance to make extreme controls of EM radiations only by
frequency.
Discussion
We proposed an efficient method to design holographic leaky-wave
metasurfaces to perform complicated multi-beam radiations with
Figure 6 | (a) The fabricated sample of the double-beam holographic metasurface and experimental setup. (b) The measured far-field radiation patterns
of the holographic metasurface and their comparisons to simulation results.
SCIENTIFIC REPORTS | 4 : 6921 | DOI: 10.1038/srep06921
6
www.nature.com/scientificreports
Table 2 | The detailed parameters of double-beam scanning in theoretical analysis
Frequency
Elevation and azimuth
Theoretical analysis
Simulation results
Experimental results
16 GHz
17 GHz
18 GHz
19 GHz
h1 w 5 0u
24u
26u
25u
h2 w 5 180u
38u
38u
38u
h1 w 5 0u
30u
30u
30u
h2 w 5 180u
30u
29u
29u
h1 w 5 0u
34u
35u
37u
h2 w 5 180u
22u
22
22
h1 w 5 0u
40u
39u
41u
h2 w 5 180u
14u
15u
14u
the metasurface with central
frequency scanning. We presented two approaches to record the
desired interferograms, one of which is recorded on the whole meta-
surface with side feeding, and the other of which is recorded on
several subdomains of
feeding.
According to theoretical analysis, the first approach can accomplish
1D frequency scanning, while the second can reach 1D and/or 2D
frequency scanning. Numerical simulation and experiment results
show that the radiation beam directions in the far fields can be coded
by frequency instead of complicated feeding network. The proposed
method has potential applications in the satellite communications
and radar systems, and can be extended to the millimeter wave and
THz regimes.
1. Smith, D. R., Pendry, J. B. & Wiltshire, M. C. K. Metamaterials and Negative
Refractive Index. Science 305, 788 (2004).
2. Schurig, D. et al. Metamaterial Electromagnetic Cloak at Microwave Frequencies.
Science 314, 977 (2006).
23. Chen, X. Z. et al. Dual-polarity plasmonic metalens for visible light. Nat.
Commun. 3, 1198 (2012).
24. Oliner, A. A. & Hessel, A. Guided waves on sinusoidally-modulated reactance
surfaces. IEEE Trans. Antennas Propag. 7, 201 (1959).
25. Fong, B. H., Colburn, J. S., Ottusch, J. J., Visher, J. L. & Sievenpiper, D. F. Scalar and
Tensor Holographic Artificial Impedance Surfaces. IEEE Trans. Antennas Propag.
58, 3212 (2010).
26. Minatti, G., Caminita, F., Casaletti, M. & Maci, S. Spiral leaky-wave antennas
based on modulated surface impedance. IEEE Trans. Antennas Propag. 59, 4436
(2011).
27. Minatti, G., Maci, S., De Vita, P., Freni, A. & Sabbadini, M. A circularly-polarized
isoflux antenna based on anisotropic metasurface. IEEE Trans. Antennas Propag.
60, 4998 (2012).
28. Hunt, J. et al. Metamaterial apertures for computational imaging. Science 339, 310
(2013).
29. Lipworth, G. et al. Metamaterial apertures for coherent computational imaging on
the physical layer. J.Opt.Soc.Am.A 30, 1603 (2013).
30. Sievenpiper, D. F., Schaffner, J. H., Song, H. J., Loo, R. Y. & Tangonan, G. Two-
dimensional beam steering using an electrically tunable impedance surface. IEEE
Trans. Antennas Propag. 51, 2713 (2003).
31. Zhao, J. et al. A tunable metamaterial absorber using varactor diodes. New J. Phys.
3. Luo, Y., Zhang, J. J., Wu, B. I. & Chen, H. S. Interaction of an electromagnetic wave
15, 043049 (2013).
with a cone-shaped invisibility cloak and polarization rotator. Phys. Rev. B 78,
125108 (2008).
4. Liu, R. et al. Broadband Ground-Plane Cloak. Science 323, 366 (2009).
5. Lai, Y., Chen, H. Y., Zhang, Z. Q. & Chan, C. T. Complementary Media Invisibility
Cloak that Cloaks Objects at a Distance Outside the Cloaking Shell. Phys. Rev. Lett.
102, 093901 (2009).
6. Zhou, J. F. et al. Terahertz chiral metamaterials with giant and dynamically
tunable optical activity. Phys. Rev. B 86, 035448 (2012).
7. Collin, R. E. Field Theory of Guided Waves (McGraw-Hill, New York, 1960).
8. Gregoire, D. J. & Kabakian, A. V. Surface-wave waveguides. IEEE Antennas
Wireless Propag. Lett. 10, 1512 (2011).
9. Bosiljevac, M., Casaletti, M., Caminita, F., Sipus, Z. & Maci, S. Non-Uniform
Metasurface Luneburg Lens Antenna Design. IEEE Trans. Antennas Propag. 60,
4065 (2012).
10. Dockrey, J. A. et al. Thin metamaterial Luneburg lens for surface waves. Phys. Rev.
B 87, 125137 (2013).
11. Wan, X., Jiang, W. X., Ma, H. F. & Cui, T. J. A broadband transformation-optics
metasurface lens. Opt. Express 21, 17531(2013).
12. Patel, A. M. & Grbic, A. A printed leaky-wave antenna based on a sinusoidally-
modulated reactance surface. IEEE Trans. Antennas Propag. 59, 2087 (2011).
13. Patel, A. M. & Grbic, A. Effective Surface Impedance of a Printed-Circuit Tensor
Impedance Surface (PCTIS). IEEE Trans. Antennas Propag. 61, 1403 (2013).
14. Kuester, E. F., Mohamed, M. A., Piket-May, M. & Holloway, C. L. Averaged
transition conditions for electromagnetic fields at a metafilm. IEEE Trans.
Antennas Propag. 51, 2641 (2003).
15. Holloway, C. L., Mohamed, M. A., Kuester, E. F. & Dienstfrey, A. Reflection and
transmission properties of a metafilm: With an application to a controllable
surface composed of resonant particles. IEEE Trans. Antennas Propag. 47, 853
(2005).
16. Holloway, C. L. et al. An Overview of the Theory and Applications of
Metasurfaces: The Two-Dimensional Equivalents of Metamaterials. IEEE Trans.
Antennas Propag. 54, 10 (2012).
17. Zhao, Y., Engheta, N. & Alu`, A. Homogenization of plasmonic metasurfaces
modeled as transmission-line loads. Metamaterials 5, 90 (2011).
18. Belokopytov, G. V., Zhuravlev, A. V. & Terekhov, Y. E. Transmission of an
electromagnetic wave through a bianisotropic metafilm. Phys. Wave Phenom. 19,
280 (2011).
19. Pfeiffer, C. & Grbic, A. Metamaterial Huygens’ Surfaces: Tailoring Wave Fronts
with Reflectionless Sheets. Phys. Rev. Lett. 110, 197401 (2013).
20. Yu, N. et al. Light Propagation with Phase Discontinuities: Generalized Laws of
Reflection and Refraction. Science 334, 333 (2011).
21. Sun, S. et al. Gradient-index meta-surfaces as a bridge linking propagating waves
and surface waves. Nat. Mater. 11, 426 (2012).
22. Kang, M., Feng, T. H., Wang, H. T. & Li, J. Wave front engineering from an array
of thin aperture antennas. Opt. Express 20, 15882 (2012).
32. Gregoire, D. J. 3-D Conformal Metasurfaces. IEEE Antennas Wireless Propag. Lett.
12, 233 (2013).
33. Germain, D., Seetharamdoo, D., Burokur, S. N. & De Lustrac, A. Phase-
compensated metasurface for a conformal microwave antenna. Appl. Phys. Lett.
103, 124102 (2013).
34. Chu, T. S. & Hashemi, H. True-Time-Delay-Based Multi-Beam Arrays. IEEE
Trans. Microwave Theory Tech. 61, 3072 (2013).
35. Cheng, Q., Jiang, W. X. & Cui, T. J. Multi-beam generations at pre-designed
directions based on anisotropic zero-index metamaterials. Appl. Phys. Lett. 99,
131913 (2011).
36. Tichit, P., Burokur, S. N. & De Lustrac, A. Spiral-like multi-beam emission via
transformation electromagnetics. J. Appl. Phys. 115, 024901 (2014).
37. Hariharan, P. Optical Holography: Principles, Techniques and Applications.
(Cambridge Univ. Press, Cambridge, , U. K 1996).
Acknowledgments
This work was supported by the National Science Foundation of China (60990320,
61138001,61171026 and 60990324), National High Tech (863) Projects (2011AA010202
and 2012AA030402), 111 Project (111-2-05) and the Natural Science Foundation of the
Jiangsu Province BK2012019, and 20130202 Guangxi Experiment Center of Information
Science, Guilin University of Electronic Technology.
Author contributions
Y.B.L. and T.J.C. conceived the idea, did the theoretical calculations, and wrote the
manuscript. Y.B. Li designed the samples and performed the measurements. X.W., B.G.C.
and Q.C. involved in the simulations and measurement. All authors contributed to the
discussions.
Additional information
Competing financial interests: The authors declare no competing financial interests.
How to cite this article: Li, Y.B., Wan, X., Cai, B.G., Cheng, Q. & Cui, T.J.
Frequency-Controls of Electromagnetic Multi-Beam Scanning by Metasurfaces. Sci. Rep. 4,
6921; DOI:10.1038/srep06921 (2014).
This work is licensed under a Creative Commons Attribution-NonCommercial-
NoDerivs 4.0 International License. The images or other third party material in
this article are included in the article’s Creative Commons license, unless indicated
otherwise in the credit line; if the material is not included under the Creative
Commons license, users will need to obtain permission from the license holder
in order to reproduce the material. To view a copy of this license, visit http://
creativecommons.org/licenses/by-nc-nd/4.0/
SCIENTIFIC REPORTS | 4 : 6921 | DOI: 10.1038/srep06921
7
相关推荐
2023年江西萍乡中考道德与法治真题及答案.doc
2012年重庆南川中考生物真题及答案.doc
2013年江西师范大学地理学综合及文艺理论基础考研真题.doc
2020年四川甘孜小升初语文真题及答案I卷.doc
2020年注册岩土工程师专业基础考试真题及答案.doc
2023-2024学年福建省厦门市九年级上学期数学月考试题及答案.doc
2021-2022学年辽宁省沈阳市大东区九年级上学期语文期末试题及答案.doc
2022-2023学年北京东城区初三第一学期物理期末试卷及答案.doc
2018上半年江西教师资格初中地理学科知识与教学能力真题及答案.doc
2012年河北国家公务员申论考试真题及答案-省级.doc
2020-2021学年江苏省扬州市江都区邵樊片九年级上学期数学第一次质量检测试题及答案.doc
2022下半年黑龙江教师资格证中学综合素质真题及答案.doc
