Microstrip Antenna Technology.pdf-资料库

2 IEEE TRANSACTIONS ON ANTENNAS AND PROPAGATION, VOL. "-29, NO. 1, JANUARY 1981 Microstrip Antenna Technology KEITH R. CARVER, MEMBER, IEEE, AND JAMES w. MINK, MEMBER, IEEE with the relation resonant freqnency of Absfruct-A survey of microstrip antenna elements is presented, with emphasis on theoretical and practical design techniques. Available substrate materials are reviewed along between dielectric constant tolerance and microstrip patches. Several theoretical analysis techniques are summarized, including transmission-line and modal-expansion (cavity) techniques as well as numerical methods such as the method of moments and fmite-element techniques. Practical procedures are given for both standard rectangular and circular patches, as well as variations on those designs including circularly polarized microstrip patches. The quality, bandwidth, and efficiency factors of typical patch designs are discussed. Microstrip dipole and conformal antennas are summarized. Finally, critical needs for fnrther research and development for this antenna are identified. T INTRODUCTION HE PURPOSES of this paper are to describe analytical and experimental design approaches for microstrip antenna the state for the current provide a reference review of as it affects the describes several of relatively new of to a les- This elements, and to provide a comprehensive survey of the state of microstrip antenna element technology. A companion paper [ 1 ] discussed microstrip array design techniques. Taken together, these papers state of development of microstrip elements and arrays elements at a time when advancements in this technology are being reported primarily in a wide variety technical reports and private communications, and ser extent in this TRANSACTIONS and other journals. paper begins with a materials technology antennas, and then to the analysis of rectangular and circular patches, as well as patches of other shapes and microstrip dipoles. Design curves are presented for both rectangular and circular patch shapes, and for A dis- cussion of the bandwidth and efficiency of the elements is presented with the patch size, shape, substrate thickness, and material properties as parameters. Several practical techniques are outlined for modifying as conformal arrays, feeds for dishes, purpose applications dual-frequency communication systems, etc. The paper con- cludes with suggestions for future critical needs in the further development of the antenna. design of microstrip theoretical approaches linearly and circularly polarized elements. the basic element for such special of printed circuit The microstrip antenna concept dates back about 26 years to work in the U.S.A. by Deschamps [ 21 and in France by Gutton and Baissinot [ 3 ] . Shortly thereafter, Lewin [ 991 investigated radiation from stripline discontinuities. Additional studies were undertaken in the late studied basic rectangular and square configurations. However, other than the original Deschamps 1960's by Kaloi, who report, work was not Manuscript received March 5, 1980; revised July 22, 1980. K. R. Carver is with the Physical Science Laboratory, New Mexico J. W. Mink is with the U.S. Army Research Office, Research Tri- State University, Las Cruces, NM 80003. angle Park, NC 27709. early 1970's, when a con- was patented by Munson and several-wavelength long reported in the literature until the ducting strip radiator separated from a ground plane by a [4]. This half- dielectric substrate was described by Byron wavelength wide strip was fed by coaxial connections at periodic intervals along both radiat- ing edges, and was used as an array for Project Camel. Shortly thereafter, a microstrip element [SI and data on basic rectangular and circular microstrip [ 6 ] . Weinschel [7] de- patches were published by Howell veloped several microstrip geometries for use with cylindrical S-band arrays on rockets. Sanford [ 81 showed that the micro- strip element could be used in conformal array designs for L-band communication from a KC-135 aircraft to the ATS-6 satellite. Additional work on basic microstrip patch elements was reported in 1975 by Garvin et d . [ 91, Howell [ 101, Weinschel [ 111, and Janes and Wilson [ 121. The early work by Munson on the development of microstrip antennas for use on rockets and mis- as low-profie flush-mounted antennas siles showed that this was a practical concept for use in many antenna system problems, and thereby gave birth to a new antenna industry. Mathematical modeling of the basic microstrip radiator [ 131, [ 141. The radiation pattern of transmission- the center of a was initially carried out by the application line analogies to simple rectangular patches fed at of a radiating wall circular patch was analyzed and measurements reported by Carver [ 151. The first mathematical analysis of a wide variety of microstrip patch shapes was published in 1977 by Lo e t d . [ 161, who used the. modal-expansion technique to analyze rectangular, circular, semicircular, and triangular patch shapes. Similar comprehensive reports on advanced analysis techniques were published by Derneryd [ 141, [ 171, Shen and Long [ 181, and Carver and Coffey [ 191. By 1978 the microstrip patch antenna was becoming much more widely known and used in a variety companied by increased attention munity to improved mathematical models used for design. In October 1979, the meeting devoted designs, array configurations, held at New Mexico State University (NMSU), Las Cruces, under cosponsorship of NMSU's Physical Science Laboratory [ 201. was ac- by the theoretical com- to microstrip antenna materials, practical of communication systems. This the U.S. Army Research Office first international and theoretical models was which could be and The terms stripline and microstrip are often encountered in the literature, in connection with both transmission lines and antennas. A stripline or triplate device is a sandwich of three parallel conducting layers separated di- electric substrates, the center conductor of which is analogous to the center conductor of a coaxial transmission line. If the to a resonant slot cut orthogonally center conductor couples in the upper conductor, the device is said to be a stripline on this radiator [ 21.1. Although there are many variations printed-circuit stripline slot antenna, these are outside the scope of this paper and will not be considered further. by two thin By contrast a microstrip device in its simplest form con- 0018-926X/81/0100-0002$00.75 0 1981 IEEE

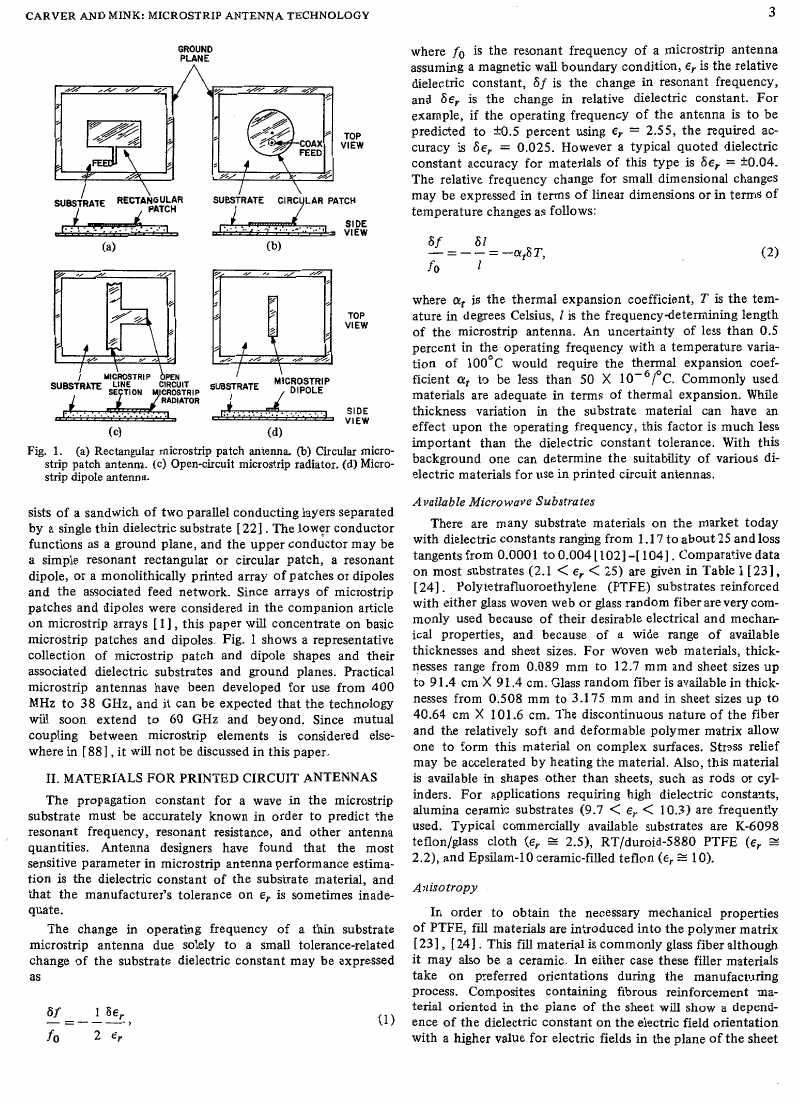
MICROSTRIP CARVER AND MINK: ANTENNA TECHNOLOGY 3 GROUND PLANE TOP VIEW TOP VIEW Fig. 1. (a) Rectangular microstrip patch antenna. (b) Circular micro- (d) Micro- strip patch antenna. (c) Open-circuit microstrip radiator. strip dipole antenna. or circular patch, a resonant sists of a sandwich of two parallel conducting layers separated by a single thin dielectric substrate [ 221. The lowfr conductor functions as a ground plane, and the upper conductor may be a simple resonant rectangular dipole, or a monolithically printed array of patches or dipoles and the associated feed network. Since arrays of microstrip patches and dipoles were considered in the companion article on microstrip arrays [ 11 , this paper will concentrate on basic microstrip patches and dipoles. Fig. 1 shows a representative collection of microstrip patch and dipole shapes and their associated dielectric microstrip antennas have been developed for use from MHz to 38 GHz, and it can be expected that the technology will soon extend to 60 GHz and beyond. coupling between microstrip elements where in [ 88 ] , it will not be discussed in this paper. Since mutual is considered else- substrates and ground planes. Practical 400 11. MATERIALS FOR PRINTED CIRCUIT ANTENNAS The propagation constant for a wave in the microstrip to predict the resistance, and other antenna substrate must be accurately known in order resonant frequency, resonant quantities. Antenna designers have found that the most sensitive parameter in microstrip antenna performance estima- tion is the dielectric constant of the substrate material, and that the manufacturer’s tolerance on E, is sometimes inade- quate. The change in operating frequency microstrip antenna due solely change of the substrate dielectric constant may be as of a thin substrate to a small tolerance-related expressed of a microstrip antenna where fo is the resonant frequency assuming a magnetic wall boundary condition, E, is the relative Sf is the change in dielectric constant, resonant frequency, and & E , is the change in relative dielectric constant. For example, if the operating frequency of the antenna is to be predicted to k0.5 percent using E, = 2.55, the required ac- curacy is & E , = 0.025. However a of this type is 8 ~ , = k0.04. constant accuracy for materials The relative frequency change for small dimensional changes may be expressed in terms of linear dimensions or in terms of temperature changes as follows: typical quoted dielectric where a, is the thermal expansion coefficient, T is the tem- ature in degrees Celsius, 2 is the frequencydetermining length of the microstrip antenna. An uncertainty of less than 0.5 percent in the operating frequency with a temperature varia- tion of 100°C would require ficient a, to be less than 50 X 10-6/oC. Commonly materials are adequate in terms thickness variation in the substrate material can have an effect upon the operating frequency, this factor is much less important than the dielectric constant tolerance. With this background one can determine the suitability of various di- electric materials for use in printed circuit antennas. used of thermal expansion. While the thermal expansion coef- Available Microwave Substrates There are many substrate materials on the market today because of a wide range of available sizes. For Woven web materials, thick- with dielectric constants ranging from 1.17 to about 25 and loss tangents from 0.0001 to 0.004[ 10214 1041. Comparative data on most substrates (2.1 < E , < 25) are given in Table I [ 23 1, [ 241. Polytetrafluoroethylene (PTFE) substrates reinforced with either glass woven web or glass random fiber are very com- monly used because of their desirable electrical and mechan- ical properties, and thicknesses and sheet nesses range from 0.089 mm to 12.7 mm and sheet sizes up to 9 1.4 cm X 9 1.4 cm. Glass random fiber is available in thick- sizes up to nesses from 0.508 mm 40.64 cm x 10 1.6 cm. The discontinuous nature of the fiber and the relatively soft and deformable polymer matrix allow one to form this material relief may be accelerated by heating the material. Also, this material is available in shapes as rods or cyl- high dielectric constants, inders. For applications requiring (9.7 < E, < 10.3) are frequently alumina ceramic substrates (E, = used. Typical commercially available substrates are K-6098 teflon/glass cloth 2.2), and Epsilam-10 ceramic-filled teflon ( E , ( E , Z 2.5), RT/duroid-5880 PTFE on complex surfaces. Stress to 3.175 mm and in sheet other than sheets, such IO). Anisotropy In order to obtain the necessary mechanical properties of PTFE, fill materials are introduced into the polymer matrix [ 231, 1241. This fill material is commonly glass fiber although it may also be a ceramic. In either case these filler materials take on preferred orientations during the manufacturing process. Composites containing fibrous reinforcement ma- terial oriented in the piane of the sheet will show a depend- ence of the dielectric constant on the electric field orientation with a higher value for electric fields in the plane of the sheet

4 JEEE TRANSACTIONS ON ANTENNAS AND PROPAGATION, VOL. AP-29, NO. 1, JANUARY 1981 AN OVERVIEW OF MAJOR MICROWAVE SUBSTRATES (AFTER [23]) TABLE I (X-Band) (X-Band) Product PTFE unreinforced PTFE glass woven web PTFE glass random fiber FTFE quartz reinforced Cross linked poly styrene/ woven quartz Cross linked poly styrene/ ceramic powder-filled Cross linked poly styrene/ glass reinforced Irradiated polyolefin Irradiated polyolefin/ glass reinforced Polyphenylene oxide (PPO) Saicone resion ceramic powder-filled Sapphire Alumina ceramic Glass bonded mica Hexcell (laminate) Air with/rexolite standoffs Fused quartz Er 2.10 2.17 2.33 2.45 2.55 2.17 2.35 2.47 2.65 3 to 15 2.62 2.32 2.42 2.55 3 to 25 9.0 9.7 to 10.3 7.5 1.17 to 1.40 at 1.4 GHz 3.78 tan6 0.0004 0.0009 0.0015 0.0018 0.0022 0.0009 0.0015 0.0006 0.0005 from 0.00005 to 0.0015 0.001 0.0005 0.001 0.00016 from 0.0005 0.0001 0.0004 0.0020 - 0.001 Dimensional Temperature poor excellent very good fair excellent good fair good poor fair poor fair to medium excellent excellent excellent excellent excellent "C -21 to +260 -27 to +260 -27 to +260 -21 to +260 -21 to +260 -21 to +260 -27 to +110 -21 to +110 -27 to +lo0 -27 to +lo0 -27 to +193 -27 to +268 -24 to +371 to 1600 unclad -27 to +593 -27 to +260 . unclad - TYPICAL DIELECTRIC CONSTANT VERSUS MAJOR AXIS ORIENTATION OF THE ELECTRIC FIELD TABLE 11 (Percent) Value Direction Direction Direction X fiber PTFE 2.347 Random Ceramic PTFE Glass cloth PTFE 2.432 2.454 10.68 2.88 2.4 Y 2 Quoted - 6 Er Er 10.70 2.88 10.40 3 2.35 f 0.04 10.5 f 0.25 2.4 2.45 f 0.04 1.7 1.6 is a function of the difference in dielectric than when the field is transverse to the sheet. The magnitude of this effect constants between the fiber orientation and the volume ratio of the fiber to polymer. Typical examples of this effect are shown in Table 11. As one can see from Table 11, the value of the dielectric is essentially the value constant quoted by the manufacturer for the case where is perpendicular to the the electric field sheet. Usually this orientation of the electric field is the one needed for antenna engineers. However, the designer needs to insure the proper to be aware of this material property operation of the antenna system or for the proper interpre- tation of material measurements. In dielectric constant measurements are typically made using stripline resonator techniques. around the strip, there the measurements. 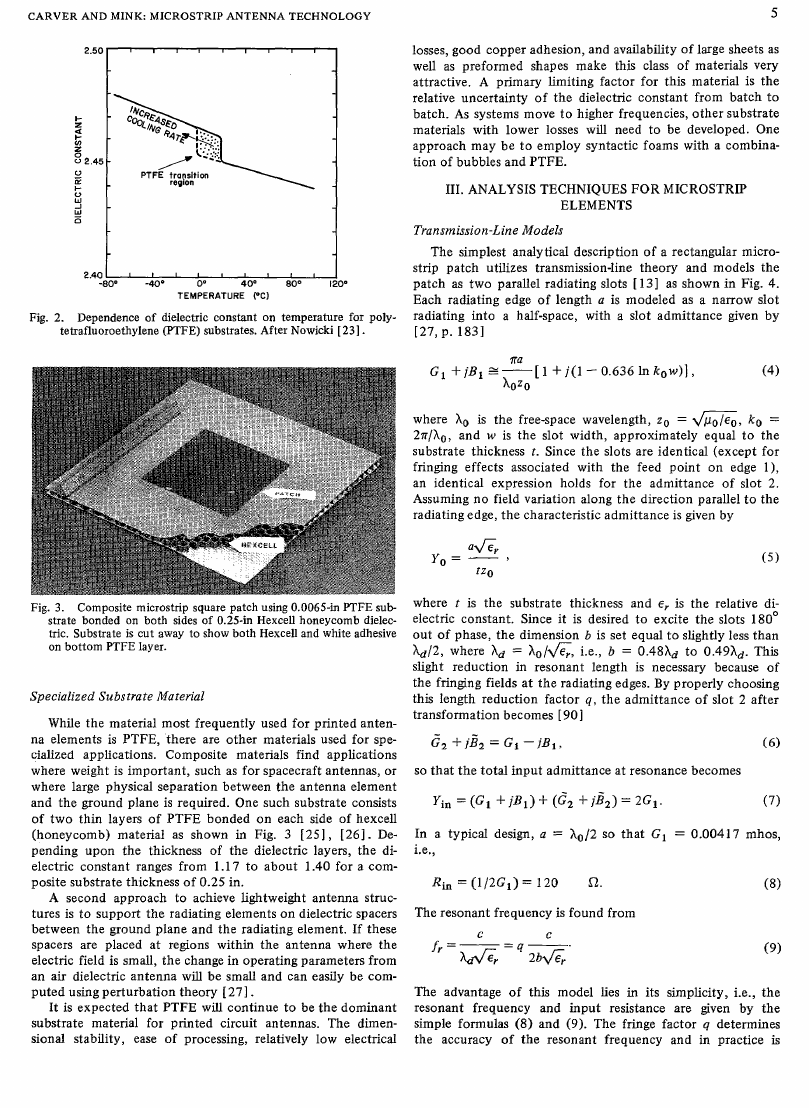The dielectric constant substrate materials tends ature as shown in Fig. 2. For this material the average change in dielectric constant over to to decrease with increasing temper- is an uncertainty associated with the temperature range -75'C Because of fringing fields the microwave region, is about C ~ E = 96 ppm/'C. An abrupt transition +100'C change of about 6 e = 0.01 1, which occurs at a temperature 2OoC, is characteristic of PTFE-based between zero and materials. The exact temperature at which this change occurs is a function of the rate at which the temperature is changing. Over the temperature range of -75'C to 100°C the relative change in operating frequencies is about 0.8 percent due to changes the change of dielectric to com- in linear dimensions due pensate the effect constant. Com- bining (1) and (2) one obtains constant. It turns out that to thermal expansion tend of a changing dielectric range from -75'C Over the temperature net change of resonant frequency with proper selection of materials, eliminate temperature effects on the resonant frequency a microstrip patch antenna. is 0.03 percent. Thus, it is possible to almost of to 100' a typical of PTFE-based

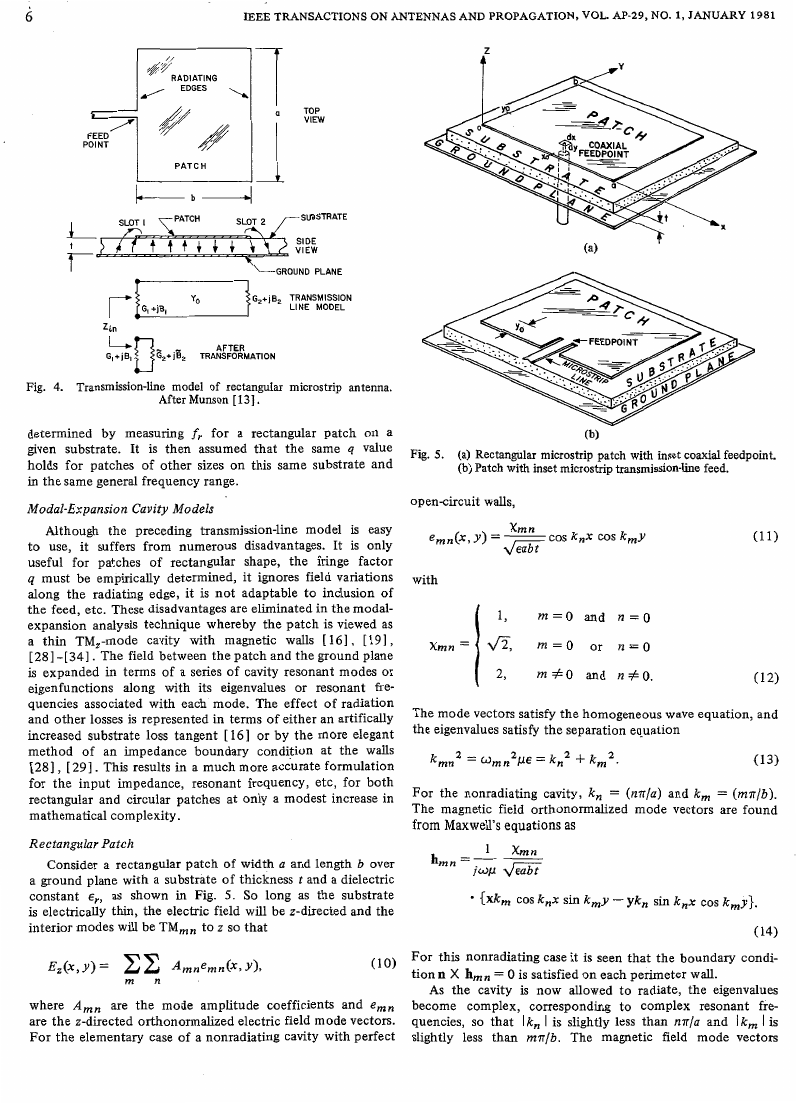
CARVER t t 2 . -800 4 0 - 4 0 0 1 i ~ 800 I ~ 120- ' ~ ' " 0" 1 TEMPERATURE PC1 ~ 40- Fig. 2. Dependence of dielectric constant on temperature for poly- tetrafluoroethylene (PTFE) substrates. After Nowicki [23]. Fig. 3. Composite microstrip square patch using 0.0065-in FTFE sub- strate bonded on both sides of 0.25-in Hexcell honeycomb dielec- tric. Substrate is cut away to show both Hexcell and white adhesive on bottom F'TFE layer. Specialized Substrate Material While the material most frequently used for printed anten- used for spe- na elements is PTFE, there are other materials cialized applications. Composite materials find applications where weight is important, such as for spacecraft antennas, or where large physical separation between the antenna element and the ground plane is required. One such substrate consists of two thin layers of PTFE bonded (honeycomb) material pending upon electric constant ranges from posite substrate thickness of 0.25 in. on each side of hexcell as shown in Fig. 3 [251, [261. De- the di- the thickness of the dielectric layers, 1 .I 7 to about 1.40 for a com- A second approach to achieve lightweight antenna struc- tures is to support the radiating elements on dielectric spacers between the ground plane and the radiating element. If these spacers are placed electric field is small, the change in operating parameters from an air dielectric antenna will be small and can easily be com- puted using perturbation theory [ 271. the antenna where the at regions within It is expected that PTFE will continue to be the dominant substrate material for printed circuit antennas. The dimen- sional stability, ease of processing, relatively low electrical 5 losses, good copper adhesion, and availability of large sheets as class of materials very well as preformed shapes make this attractive. A primary limiting factor for this material is the relative uncertainty of the dielectric constant from batch to batch. As systems move to higher frequencies, other substrate will need to be developed. One materials with lower losses approach may be to employ syntactic foams with a combina- tion of bubbles and PTFE. 111. ANALYSIS TECHNIQUES FOR MICROSTRIP ELEMENTS Transmission-Line Models The simplest analytical description of a rectangular micro- strip patch utilizes transmission-line theory and models the l patch as two parallel radiating slots Each radiating edge of length radiating into a [27, p. 183 1 a is modeled as a narrow slot half-space, with a [ 131 as shown in Fig. 4. slot admittance given by where ho is the free-space wavelength, zo = a, ko = t. Since the slots are identical (except for 2a/ho, and w is the slot width, approximately equal to the substrate thickness fringing effects associated with an identical expression holds for the admittance Assuming no field variation along the direction parallel to the radiating edge, the characteristic admittance is given by the feed point on edge l), of slot 2. where t is the substrate thickness and E, is the relative di- electric constant. Since it is desired to excite the slots 180' out of phase, the dimension b is set equal to slightly less than &/2, where & = hot&, i.e., b = 0.48hd to 0.49hd. This of slight reduction in resonant length the fringing fields at the radiating edges. By properly choosing q , the admittance of slot 2 after this length reduction factor transformation becomes [ 901 is necessary because z2 1- j i 2 = GI - j B 1 , ( 6) so that the total input admittance at resonance becomes ri, = (C, + j B , ) + (Gz + j&) = 2G1. typical design, a = X0/2 so that G1 = 0.00417 mhos, (7) In a i.e., R h = (1/2G,) = 120 a. The resonant frequency is found from C f y = - = q - . M r C 2 b G r The advantage of this model i.e., the resonant frequency and given by the simple formulas (8) and (9). The fringe factor q determines in practice is the accuracy of the resonant frequency and lies in its simplicity, input resistance are

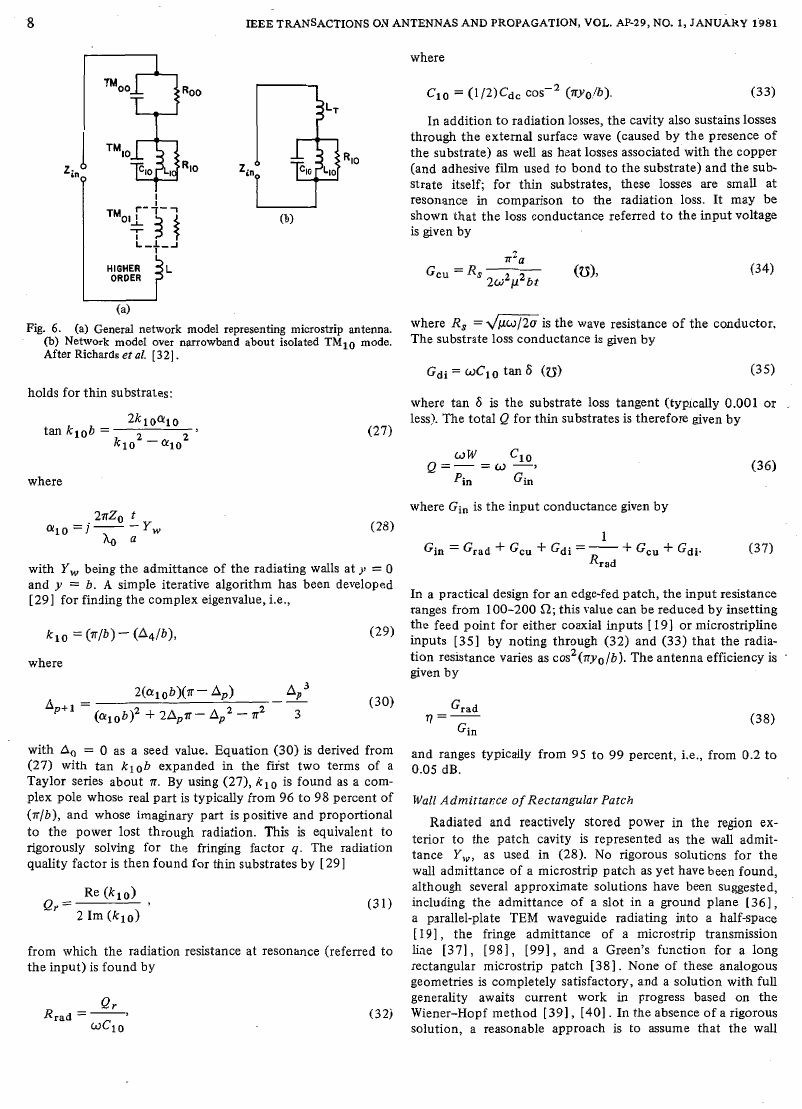
6 IEEE TRANSACTIONS ON ANTENNAS AND PROPAGATION, VOL. AP-29, NO. 1, JANUARY 1981 I 47’ I/ ” RADIATING EDGES -4 7 TOP VIEW i Fig. 5. (a) Rectangular microstrip patch with inset coaxial feedpoint. (b) Patch with inset microstrip transmission-line feed. (b) Zin g,+ji, AFTER TRANSFORMATION Fig. 4. Transmission-line model of rectangular microstrip antenna. After Munson [ 131. determined by measuring f, for a rectangular patch on a given substrate. It holds for patches of other sizes on this same substrate and in the same general frequency range. is then assumed that the same q value &iodal-Expansion Cavity Models open-circuit walls, of rectangular shape, Although the preceding transmission-line model is easy to use, it suffers from numerous disadvantages. It is only useful for patches the fringe factor q must be empirically determined, it ignores field variations along the radiating edge, it is not adaptable to inclusion of the feed, etc. These disadvantages are eliminated in the modal- expansion analysis technique whereby the patch is viewed as a thin TM,-mode cavity with magnetic walls [ 161. [ 191, [28] -[34]. The field between the patch and the ground plane is expanded in terms of a series of cavity resonant modes or eigenfunctions along with its eigenvalues or resonant fre- quencies associated with each mode. The effect of radiation and other losses is represented in terms of either an artifically [ 161 or by the more elegant increased substrate loss tangent method of an impedance boundary condition at the walls [28], [ 291. This results in a much more accurate formulation for the input etc, for both rectangular and circular patches at only a modest increase in mathematical complexity. impedance, resonant frequency, Rectangular Patch Consider a rectangular patch of width a and length b over a ground plane with a substrate of thickness f and a dielectric constant E,, as shown in Fig. 5. So long as the substrate is electrically thin, the electric field will be z-directed and the interior modes will be TM,, to z so that m n are the mode amplitude coefficients and where A,, are the z-directed orthonormalized electric field mode vectors. For the elementary case of a nonradiating cavity with perfect e,, with 1, m=O and n = O fi, 2, m = or n = ~ ~ m f O and n Z 0 . (12) The mode vectors satisfy the homogeneous wave equation, and the eigenvalues satisfy the separation equation kmn2 = u m n 2 p f = kn2 f k m 2 . (13) k , = (n.rr/a) and k , = (mn/b). For the nonradiating cavity, The magnetic field orthonormalized mode vectors are found from Maxwell’s equations as h,, =- - 1 Xmn j u p &iE * COS knx sin kmy - ykn sin knx COS k m y } . (14) For this nonradiating case it is seen that the boundary condi- tion n X hmn = 0 is satisfied on each perimeter wall. As the cavity is now allowed to radiate, the eigenvalues become complex, corresponding to complex resonant fre- quencies, so that I k, I is slightly less than RK/a and lk, I is slightly less than mx/b. The magnetic field mode vectors

CARVER AND MINK: MICROSTRIP ANTENNA TECHNOLOGY hmn no longer have a zero tangential component cavity sidewalls. However that the electric given by (1 1). field mode vectors are a perturbational solution shows still very accurately Consider now the effect of a z-directed current probe Zo of small rectangular cross section (d,dy) at (xo, y o ) as shown in Fig. 5(a). The coefficients of each electric mode vector are found from [ 271 : \\/.I em,, * dv, ?zn A m n = k2 - k m n 2 which then reduces to A m , = il, fi k2 2 Gmn COS kmY0 coskrt~o on the Therefore the input impedance 2, = 5 = -jZokr x 9 m n 2 ( X o , Y O ) is m m G m n . 10 m=o n=o k2 - kmn 7 ( 2 2 ) 5 ) .~ The (0, 0) term with koo = 0 is the static capacitance 0) term represents the dominant . term with a shunt resistance to represent loss in the sub- strate. The (1, RF mode transmission-line mode discussed in and is identical to the is the previous section; for this mode, cos (nylb) variation no field variation and in the y direction. This mode is equivalent to a parallel R-L-C network where losses. If the patch is square or nearly so, the (0, 1) mode can also be excited modes have negligible losses and sum L . All the higher order to form a net inductance as a degenerate mode. (1 1 ) shows that there the x direction R represents copper radiation, and substrate, a in Fig. 6(a) shows a general network representation of the (l6) input impedance, and Fig. 6(b) shows a network model over a narrow band about an isolated TMlo mode, where the net series inductance is LT. The feed probe diameter as expressed by the factor G,, is the major factor in determin- ing L T , since it governs the convergence of the series. Equation ( 2 2 ) can be written as where Gmn = sin (nndX/2a) sin (mndy/2b) m?rdy/2b nndX/2a (17) In (18) ij,, is the complex resonant frequency of the mnth mode as found from (13). The relation (1 5 ) for the coefficients is based on the orthogonality of the mode vectors; although the introduction of the radiation condition means that these mode vectors are no longer orthogonal in the strict sense, for electrically thin substrates the error due to this assumption is negligible. The factor G,, feed; for coaxial feeds is set equal d,d, probe. For patches yo = 0, set d , = 0 and use d , as the feed line width as a zero- order approximation ignoring junction capacitance effects. to the effective cross-section area fed by a microstrip transmission line at d, = d , and the cross-section area accounts for the width of the of the Substituting (16) into (10) we obtain with Cdc being the dc patch capacitance ( ~ ~ b l t ) , Q the quality factor for the TMlo mode, and w10 the radian frequency at both w10 and Q resonance. A simple means for determining will be given in a subsequent paragraph. The series inductive reactance is given by m n f O O Xmn 9 m n =- fi cos k,x cos kmy The voltage at the feed is now computed as v, =-fEz(Xo,Yo) which shows that the substrate thickness. series reactance is proportional to the The next problem is to find the complex eigenvalues kmn. also the Except near the TMlo mode resonant frequency (or TMol resonant frequency for nearly square patches), kmn2 (mn/b)' -I- (n ?r/a)'. The complex eigenvalue kl may be found by either lumping all the losses into an effective electric loss tangent [ 3 2 ] , or by incorporating the into the conductance of impedance-type boundary conditions to a complex transcendental eigenvalue equation di- losses of the radiating walls and imposition [28], which leads [ 2 9 ] which

8 IEEE TRANSACTIONS ON ANTENNAS AND PROPAGATION, VOL. AP-29, NO. 1, JANUARY 1.981 1 where (Wo/b). (33) clo = (1 /2)cdc COS-2 In addition to radiation losses, the cavity also sustains losses through the external surface wave (caused by the presence of the substrate) as well as heat losses associated with the copper (and adhesive fiim used to bond to the substrate) and the sub- strate itself; for thin substrates, these resonance in comparison to the shown that the loss conductance referred to the input voltage is given by radiation loss. It may be losses are small at where Rs = d The substrate loss conductance is given by a is the wave resistance of the conductor. Gdi = WC10 tan 6 (IT) (35) where tan 6 is the substrate loss tangent (typically 0.001 or less). The total Q for thin substrates is therefore given by W W Q =- = 'lo w -' Gin TMo QRo0 I I (a) Fig. 6. (a) General network model representing microstrip antenna. 0) Network model over narrowband about After Richards et al. [ 321. isolated T M ~ o mode. holds for thin substrates: tan klob = 2kl O Q 1 0 k1o2 - a102 where Pin with Y , being the admittance of the radiating walls at y = 0 and y = b. A simple iterative algorithm has been developed [29] for Tiding the complex eigenvalue, i.e., where with A0 = 0 as a seed value. Equation (30) is derived from (27) with tan k l o b expanded in the Test two terms of a Taylor series about T . By using (27), klo is found as a com- plex pole whose real part is typically from 96 to 98 percent of (rib), and whose imaginary part is positive and proportional This is equivalent to to the fringing factor q. The radiation rigorously solving quality factor is then found for thin substrates by [291 power lost through radiation. for the from which the radiation resistance at resonance (referred the input) is found by to where Gin is the input conductance given by In a practical design for an edge-fed patch, the input resistance ranges from 100-200 R; this value can be reduced by insetting the feed point for either coaxial inputs [ 191 or microstripline inputs [351 by noting through (32) and (33) that the radia- tion resistance varies as cos2(Tyo/b). The antenna efficiency is ' given by 77=: Grad Gin and ranges typically from 95 to 99 percent, i.e., from 0.2 to 0.05 dB. Wall Admittance of Rectangular Patch Radiated and reactively stored power in the region ex- cavity is represented as the wall admit- in (28). No rigorous solutions for the terior to the patch tance Y,v, as used wall admittance of a microstrip patch as yet have been found, although several approximate solutions have been suggested, including the admittance of a slot in a ground plane a parallel-plate [ 191, the line [37], [981, [99], rectangular microstrip patch [38]. None of these analogous geometries is completely satisfactory, and a solution with full on the generality awaits Wiener-Hopf method [39], [40]. In the absence of a rigorous solution, a reasonable approach is to assume that the wall fringe admittance of a microstrip transmission and a Green's function for a long current work in progress based TEM waveguide radiating [ 361, half-space into a

CARVER AND MINK: MICROSTRIP ANTENNA TECHNOLOGY 9 angle of incidence, which on the exterior grounded substrate. that the wall admittance is which can propagate Importantly, this analysis shows a function of both frequency and then shows that Y , cannot be rigorously represented by the (39) or (40) which assume normal approximate expressions Y , will depend incidence. We may therefore anticipate that on both dimensions a and b. Carver [ 291, by near-field prob- ing of the fields near the wall, has shown empirically that the wall admittance expressions modified by multiplying Y , by an aspect ratio factor F,,(a/b) given by F ~ ( Q / ~ ) = 0.7747 + 0.5977 (a/b - I ) - 0.1638 (a/b - 112, (44) and (43) may be (39), (40), to better agreement of the predicted resonant and S-band than by assuming that fre- to its validity is unknown; clearly more work which leads resistance and resonant frequency versus aspect ratio with measured results at L-band F,, = 1. Nonetheless, (44) is empirical, and quency limit in the numerical evaluation of the Wiener-Hopf solution is. needed, perhaps reducing this to as given in (44). Radiation Pattern of Rectangular Patch curve-fit polynomials such the upper The far-field radiation pattern of a rectangular microstrip is broad in both the in the TMlo mode E ground by modeling the radiator as either of length a, sep- patch operating and H planes. The pattern of a patch over a large plane may be calculated two parallel uniform magnetic line sources arated by distance current sources as suggested in Fig. 7. The effect of the ground an plane and substrate electrical distance k t . If the slot voltage across either radiating edge is taken as VO, the calculated fields are b [96], or as two equivalent electric is handled by imaging the slot at J ko - sin 0 sin q5 a 2 conductance is that of a wave normally incident on a pard- lel-plate TEM waveguide slot radiating into a half-space [271; for electrically small slot widths, length is given by n/(376ho) mho/m. If it is further assumed is excited, then the wave that only the dominant TMlo mode the field is normally incident on the radiating edges with intensity being uniform across both this case the total wall conductance is given by the conductance per unit of these edges. In G, = (n/376)(a/b) (7Jr). (39) The wall susceptance may be approximated from Hammer- stad’s formula for circuit [ 371 and assumes the form the capacitance of an open microstrip B , = 0.01668 (AZ/t)(a/x,)e, where (u), (40) a - + 0.262 t - + 0.813 a t 1 +l&]-”’ and E, is an effective dielectric constant given by [41] ee=-+- e,+ 1 2 2 E,--- 1 so that the TMlo edges is lumped wall admittance of the radiating Y , = G, i- jB,. (43) It should be noted that the susceptance given by (40) is based on Hammerstad’s nondispersive and disagrees with the susceptance given in (4) which is based is rigorously on a dynamic capacitance. Neither formula correct for the microstrip antenna, and better relations await theoretical work in progress. static capacitance relation It will be shown in a subsequent section that (39) and (40) lead to a prediction of resonant input resistance and resonant frequency which is in good agreement with measured results for the aspect ratios 1 < a/b < 2; for larger aspect ratios, the assumption of a uniform field and normal incidence on the ra- diating edges is no longer very good, so that (39) and (40) are in- sufficiently accurate. The advantage to this impedance bound- ary condition method field through Y , is that it explicitly provides (through the eigen- value equation (27)) for improved solutions problem, when these are published in future literature. of representing the exterior to the exterior It should be mentioned that the mode vectors of (1 1) may be viewed as spatial harmonics resulting from the resonance of quasi-TEM plane waves launched from the feed which, by zig-zagging off the cavity parameter wall, travel a total distance the walls so as to produce con- and experience phase shifts at structive interference. An analysis of this resonance condition as a function of the patch aspect ratio a/b has been provided by Chang and Kuester [ 421 , who have shown that an optimum in the sense of low-Q opera- range for the aspect ratio exists tion. The Wiener-Hopf technique was used to obtain the wall reflection coefficient in p M - strate thickness, and dielectric constant) ciple then be used re- flection coefficient involves two infinite integrals, the evalua- tion of which reveals both LSE and LSM surface-wave modes (as a function of incidence angle, sub- to obtain the wall admittance. The which may

资料库

Microstrip Antenna Technology.pdf

相关推荐

移动开发

热门标签

最新资料