Finite size effects on the helical edge states on the Lieb latti....pdf

发布时间：2022-05-29 发布人：admin 分类：说明书资料大小：1.01M 资料格式：pdf 举报版权申诉

weixin_38612648-12151314-4744302542847850545.pdf-第1页.png

第1页 / 共16页

weixin_38612648-12151314-4744302542847850545.pdf-第2页.png

第2页 / 共16页

weixin_38612648-12151314-4744302542847850545.pdf-第3页.png

第3页 / 共16页

weixin_38612648-12151314-4744302542847850545.pdf-第4页.png

第4页 / 共16页

weixin_38612648-12151314-4744302542847850545.pdf-第5页.png

第5页 / 共16页

weixin_38612648-12151314-4744302542847850545.pdf-第6页.png

第6页 / 共16页

weixin_38612648-12151314-4744302542847850545.pdf-第7页.png

第7页 / 共16页

weixin_38612648-12151314-4744302542847850545.pdf-第8页.png

第8页 / 共16页

文本预览

http://www.paper.edu.cn Lieb晶格中螺旋边界态的有限尺寸效应陈锐，周斌湖北大学物理与电子科学学院，武汉　430062 摘要：在本文，我们研究在Lieb晶格中螺旋边界态的有限尺寸效应，我们考虑了三种不同的边界情况：直边界，胡须型边界和不对称边界。我们解析地得到了紧束缚模型下的Lieb晶格边界模式的能谱和波函数。在直边界的条状Lieb模型中，本征自旋轨道耦合导致类狄拉克体态的波函数局域到边界上，形成了具有同样类狄拉克能谱的螺旋边界态。在直边界情况，无能隙的类狄拉克能谱随着材料的宽度减小保持不变，量子自旋霍尔态的有限尺寸效应没有出现。在另两种情况下，随着条状样品宽度的减小，有限尺寸效应导致的能隙将被打开。关键词：量子自旋霍尔效应；有限尺寸效应；自旋轨道耦合；拓扑绝缘体中图分类号： O469 Finite size eﬀects on the helical edge states on the Lieb lattice Chen Rui, Zhou Bin Faculty of Physics and Electronic Technology, Hubei University, Wuhan 430062 Abstract: In this paper, we investigate the ﬁnite size eﬀect of helical edge states on the Lieb lattice with ISO coupling under three diﬀerent kinds of boundary conditions, i.e., the straight, bearded and asymmetry edges. The spectrum and wave function of edge modes are derived analytically for a tight-binding model on the Lieb lattice. For a strip Lieb lattice with two straight edges, the ISO coupling induces the Dirac-like bulk states to localize at the edges to become the helical edge states with the same Dirac-like spectrum. Moreover, it is found that in the case with two straight edges the gapless Dirac-like spectrum remains unchanged with decreasing the width of the strip Lieb lattice, and no gap is opened in the edge band, that is, ﬁnite size eﬀect of QSH states is absent. However, in the other two cases with the bearded and asymmetry edges, the energy gap induced by ﬁnite size eﬀect is still opened with decreasing the width of the strip. Key words: quantum spin Hall state; ﬁnite size eﬀect; spin-orbit coupling; topological insulators 基金项目： National Natural Science Foundation of China (Grant No. 11274102), Program for New Century Excellent Talents in University of Ministry of Education of China (Grant No. NCET-11-0960), and Specialized Research Fund for the Doctoral Program of Higher Education of China (Grant No. 20134208110001). 作者简介： Rui Chen (1992-), male, postgraduate student, semiconductor spintronics. Corresponding Author: Zhou Bin (1972-), male, professor, semiconductor spintronics. Email: binzhou@hubu.edu.cn - 1 -

http://www.paper.edu.cn 0 Introduction A two-dimensional Lieb lattice, [1] also called a line-centered square lattice, has attracted considerable research interest due to the speciﬁc properties induced by its topology. Each unit cell on the Lieb lattice contains three atoms (A, B, and C) (cf. Fig. 1(a)). On the other hand, quantum spin Hall (QSH) eﬀects, also called two-dimensional topological insulators, and three- dimensional topological insulators have become immensely popular and active research ﬁelds in the condensed matter physics community. [2, 3] When the intrinsic spin-orbit (ISO) coupling is introduced to the Lieb lattice, a topologically nontrivial bulk gap is opened and it gives rise to QSH eﬀect characterized by two pairs of gapless helical edge states within the bulk gap. [4] Recently, topological phase transitions driven by diﬀerent parameters on the Lieb lattice have also been furtherly investigated. [5–9] Noted that the ﬁnite size eﬀect in topological insulators was ﬁrstly proposed in QSH states of HgTe quantum well, due to the fact the edge states on the two sides can couple together to produce a gap in the spectrum. [10] Later, the ﬁnite size eﬀect has also been predicted theoretically in three-dimensional topological insulator thin ﬁlms. [11–14] Moreover, the gap due to the ﬁnite size eﬀect has been conﬁrmed experimentally. [15, 16] It is proposed that the role of the ﬁnite size eﬀect in topological insulators should be considered seriously in potential use of the topologically protected edge (surface) states in application on the nanometer scale. Fig 1: (Color online) Illustration of the Lieb lattice. (a) A two-dimensional Lieb lattice. (b), (c) and (d) Scheme of the strip lattice with the straight, bearded and asymmetry edges. In this paper, we focus on the inﬂuence of the diﬀerent edge geometries of the strip Lieb lattices on the ﬁnite size eﬀect of helical edge state. Three kinds of edge geometries, i.e., straight, bearded and asymmetry edges (cf. Fig. 1(b-d)), are investigated, respectively. Based on the tight-binding model, the spectrum and wave function of edge modes are derived analytically. - 2 - xay(a)ABC(b)(d)(c)

http://www.paper.edu.cn 1 The edge modes under semi-inﬁnite boundary conditions In this section, we discuss the edge modes on the Lieb lattice with a semi-inﬁnite geometry. We start from the Hamiltonian of the tight-binding model for a two-dimensional Lieb lattice with ISO coupling [4] (1) where the spin-independent nearest-neighbor (NN) hopping term H0 and ISO coupling term Hso are given as follows, respectively, H = H0 + Hso, † c iσcjσ, i,jσ iσ(dk × dl) · σσσcjσ. † c H0 = t i,jσσ Hso = iλ (2) (3) iσ (ciσ) is the creation (annihilation) operator for a particle with spin σ (=↑, ↓) on site † Here, c i of the Lieb lattice, t is the NN hopping amplitude, λ presents the amplitude of ISO coupling induced by the spin-dependent next-nearest-neighbor (NNN) hopping (shown by the dashed lines in Fig. 1), σ is the vector of Pauli spin matrices, dk and dl are the two displacement unit vectors of the NNN hopping process connecting sites i and j. 1.1 The case with the straight edge Firstly, we focus on the case of semi-inﬁnite geometry with a straight edge. Here, we mention that in the y-axis direction the half plane begins with the type A and B sites (see the upper boundary in Fig. 1(b)). Here and hereafter, a periodic boundary condition along the x-axis direction is considered. The corresponding Schr¨odinger equation for spin-up part is given by where t† a εa . . . a t† . . . ta . . . εa . . . εa ta  −E εa = τ0 t −E −τso τ0 t −τso −E   ta = φ1 φ2 ... φj ...  = 0, 0 0 0 0 τso 0 0 0 t a t† . . .  , - 3 - (4) (5)  ,

http://www.paper.edu.cn and φj =ψA T j , ψB j , ψC j with τ0 = 2tcx and τso = 2λsx. Here we only consider the edge modes and try an edge state solution with property: φj+1 = ρφj ≡ ρjφ with |ρ| < 1. Then Eq. (4) can be reduced into the two equations as follows, an initial equation (εa + ρt† a)φ = 0, ρj−2(ta + εaρ + t† aρ2)φ = 0, and the inner one with j = 2, 3, ··· , ∞. By solving Eqs. (6) and (7), one can get the energy spectrum of spin-up edge state and the corresponding eigenstate φs↑ = Es↑(kx) = 2t cos(kx/2), T 1, 1, 0 , with ρ = τso − t τso + t . (6) (7) (8) (9) (10) It is indicated that the edge mode is composed only from orbitals of the type A and B of the Lieb lattice with a straight edge. 1.2 The case with the bearded edge Let us turn to the case of a diﬀerent boundary geometry, the bearded edge geometry. In the y-axis direction, the half plane begins with the C-type sites (see the upper boundary in Fig. 1(c)). Under the semi-inﬁnite boundary condition, the corresponding Schr¨odinger equation for εd td  −E spin-up part is given by with j =ψC and φ j , ψA j , ψB j εd = T t † d εd . . . † t d . . . td t τso t −E τ0 τ0 −E τso  = 0   , td = † t d . . . . . . εd . . . 1 2 j φ φ ... φ ...  0  , t −τso 0 0 0 0 0 0 (11) (12) (13) . We assume an edge state solution as follows φ j+1 = χφ j ≡ χjφ, - 4 -

edge modes with the energy bands τsot Eb↑±(kx) = and the corresponding eigenstates √ τ0± τso where ωA± = φb↑± = t , ωB± = 0 +4t2 so − t2 τ 2 2(τ 2 so + t2)2 so+τ 2 4τ 2 so+t2) 2(τ 2 χ± = , so + τ 2 0 + 4t2 τ0 ±4τ 2 1, ωA±, ωB± √ τ0± τ 2 so + t2 T 0 +4t2 so + 2t2 ± τ0 so+τ 2 4τ 2 so+t2) 2(τ 2 , , and (16) (17) (18) . http://www.paper.edu.cn with |χ| < 1. Substituting Eq. (13) into Eq. (11), one can get two equations as follows (εd + χt † d)φ = 0, † dχ2)φ = 0. χj−2(td + εdχ + t (14) (15) By solving Eqs. (14) and (15), one ﬁnds that for spin-up part there are two branches of τ 2 0 + 2τ 2 4τ 2 so + τ 2 0 + 4t2 Two branches of edge modes Eb↑± given by Eq. (16) are plotted in blue and red dashed lines, respectively, in Fig. 2(a). Diﬀerent from the case of the straight edge, the edge mode with the bearded edge is composed from orbitals of the type A, B and C of the Lieb lattice. 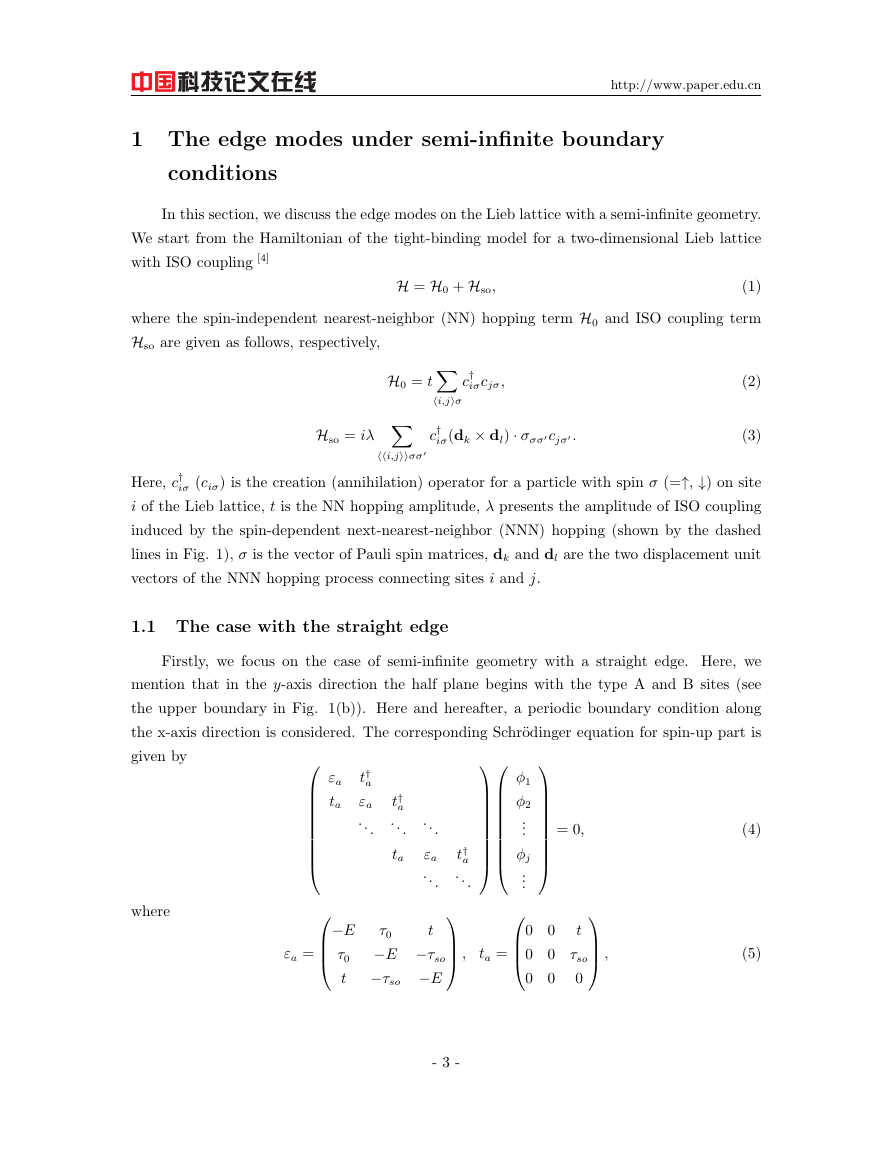Fig 2: (Color online) Band structure and the parameters χ± varies with kx. spectrum for spin-up part with λ = 0.6t under bearded boundary condition. The blue and red dashed lines correspond to the energy Eb↑± given by Eq. (16). (b) The variations χ± with kx. The blue and red solid lines correspond to χ+ and χ−, respectively. Here, the green dashed lines locate at kc± x . (a) Energy - 5 - 0.00.51.01.52.0-2-101-202(b) ckx/p E/t(a)

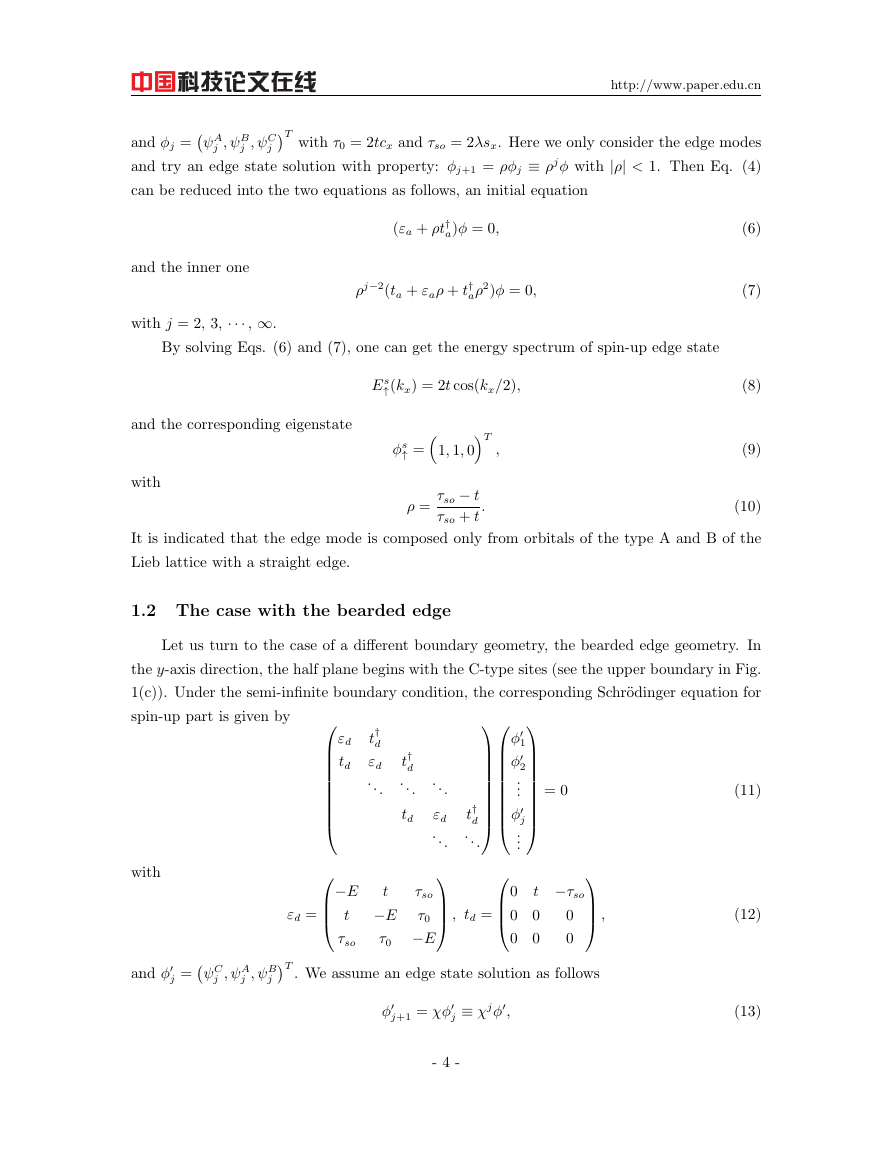
1.3 The characteristic parameter of the edge modes http://www.paper.edu.cn The parameters ρ and χ (with |ρ|, |χ| < 1) characterize the localization scale of the edge 0 ≡ |ln|χ||), then l0 denotes the localization length of the 0 ≡ |ln|ρ|| (or l−1 modes. Deﬁning l−1 edge mode. From Eq. (10), it is found that when kx = 0 and 2π, |ρ| = 1. Thus, for the case with the straight edge, the edge mode merges into the bulk excitations at the center of the Brillouin zone. By solving |χ±| = 1, one can obtain cos2 kc± x 2 = 32λ4 √ t4 − 4t2λ2 + 32λ4 + t2 t4 − 8t2λ2 + 80λ4 , (19) x here kc± the bearded edge. In Fig. 2, kc± |χ+| 1, and for kc− is the momentum where the edge states merge into the bulk states for the case with x are highlighted by green dashed lines. For 0 kx kc+ x , kx 2π, |χ−| 1, therefore, the edge mode is absent. x For the case with the straight edge, the edge energy bands Es σ(kx) are unrelated to the amplitude of ISO coupling λ, while the characteristic parameter ρ varies with λ. However, for the case with the bearded edge, both the edge energy bands Eb σ±(kx) and the characteristic parameters χ± are dependent on λ. At kx = π, ρ = ρ0 = (2λ − t) / (2λ + t) and χ± = χ0 = (4λ2 − t2) / (4λ2 + t2). The variations of |ρ0| and |χ0| with λ are shown in Fig. 3. With the increase of λ from zero, |ρ0| and |χ0| decrease ﬁrst up to zero at λ = 0.5t and then increase. It is indicated that the localization lengths of the edge modes vary non-monotonically with the amplitude of ISO coupling λ. At λ = 0.5t, |ρ0| = |χ0| = 0, that is, the edge states are completely localized on the most boundary unit cell. Additionally, from Fig. 3, it is shown that the localization length of the edge mode in the case with the straight edge is smaller than the case with the bearded edge for the same value of λ (except λ = 0.5t). Fig 3: The variations of |ρ0| and |χ0| with λ. The red and blue lines correspond to |ρ0| and |χ0|, respectively. - 6 - 0.00.20.40.60.81.00.00.20.40.60.81.0 |r0|,|c0|λ/t |r0| |c0|

http://www.paper.edu.cn 2 The edge modes with the strip geometry In this section, we will investigate the edge modes on the Lieb lattice with the strip geometry. When the width of the strip model is comparable to the localization length of the edge modes, the ﬁnite size eﬀect of QSH states has to be considered. 2.1 The case with the straight edges For the strip geometry with the straight edges, as shown in Fig. 1(b), both the upper and lower edges are composed by A and B type atoms. Considering a strip Lieb lattice with n unit cells along the y-axis direction, the total atom number is 3n− 1. A and B type atoms have the same atom number n, while the number of C type atoms is n − 1. The general eigenstates and the determinant of the secular equation for spin-up part are written as follows Ψ↑ s(n) = (ψA 1 , ψB 1 , ψC 1 , ψA 2 ,··· , ψB n−1, ψC n−1, ψA n , ψB n )T , Then we obtain the general expression of Fs(n), where Q± = α ± β, α =E2 − τ 2 Fs(n) = 0 − 4τ 2 Letting Fs(n) = 0, it is easy to ﬁnd E + − Q2n− ) En−1(E2 − τ 2 0 )(Q2n (−1)n+122nαβ so and β =E2 − τ 2 0 − 4t2. ↑s± = ±τ0 and E ↑ 0 = 0 are always the solutions ↑s± are of the secular equation. The components of eigenstates corresponding to eigenenergy E obtained ψA i (E ψB i (E ψC i (E ↑s± ) = ρi−1± , ↑s± ) = ±ρi−1± , ↑s± ) = 0, (i = 1, 2,··· , n), (i = 1, 2,··· , n), (i = 1, 2,··· , n − 1). (24) - 7 - and with Fs(n) = H↑ s (n) − E εa ta t† a εa . . . a t† . . . ta = . . . εa tb −E τ0 τ0 −E , tb = εb = 0 0 0 0 τso . t † b εb t , , (20) (21) (22) (23)

http://www.paper.edu.cn Thus, E to the spectra E ↑s± are the enengy spectra of edge modes for spin-up part. The edge states corresponding ↑s− localize at the upper and lower boundary of the strip geometry ↑s + in the strip geometry with the straight edges are the same as the case under semi-inﬁnite boundary It is emphasized that the edge spectrum E with the straight edges, respectively. ↑s + and E conditions with the straight edge (see Eq. (8)). In other words, in this case with two straight ↑s± remains unchanged with decreasing the width of the strip Lieb lattice, and no gap is opened in the edge band. Thus, it is concluded that ﬁnite size edges the gapless Dirac-like spectrum E eﬀect of QSH states does not occur on the Lieb lattice in the strip geometry with the straight edges, due to the fact that the wave functions of the two edge states localized at the upper and lower boundary do not couple together at the Dirac point, while the width of the strip model is comparable to the localization length of the edge modes. Such a ﬁnding is a new result, which is strikingly diﬀerent from the ﬁnite size eﬀect on HgTe quantum well discussed previously. [10] Additionally, the edge modes are composed only from orbitals of the types A and B and irrelevant to C type atoms, which is consistent with the result obtained under the semi-inﬁnite boundary condition. The degeneracy of the ﬂat bands (E 0 = 0) is n− 1, which is equal to the number of C type ↑ atom along the y-axis direction on the Lieb lattice. The dispersive bulk energy spectra can be n , where N = 1, 2, ··· , n − 1. So the spectra of the given by solving the equation Q− Q+ 2n . We notice N±(kx) = ± 0 + 4t2 cos2 N π τ 2 so sin2 N π 2n + 4τ 2 = eiπ N dispersive bulk states have the form Es that for λ t/2, at the momenta the dispersive bulk energy spectra are (n − 1)-fold degeneracy and can be given by N± = ±t Es 8 − x± = 2 arcsin(± t k0 2λ ), t 2 . λ (25) (26) The energy spectrum and wave function distribution for spin-up part are plotted in Fig. 4, obtained by numerical diagonalization of the tight-binding Hamiltonian of a strip lattice geometry. In fact, the above results given analytically are consistent with these of numerical diagonalization. In the absence of ISO coupling (i.e., λ = 0), it is shown in Fig. 4(a) that the gapless Dirac-like spectrum (±2t|cos(kx/2)|) is intersected by the ﬂat bands. By checking the wave function distribution of the Dirac-like spectrum (see the upper layer in Fig. 4(d)), it is found that this Dirac-like spectrum actually corresponds to the bulk state. Taking into account of ISO coupling (i.e., λ = 0), the Dirac-like spectrum remain still unchanged (see Fig. In Fig. 4(c), we plot the 4(b)), but now it corresponds to the edge mode (see Fig. 4(d)). dependence of the spectra on λ at kx = π + 0.1, in which three straight lines appear: the green line corresponding to the ﬂat bands and two red line to the edge states. It is indicated that for - 8 -

分享到：

赞收藏

资料库

Finite size effects on the helical edge states on the Lieb latti....pdf

相关推荐

开发技术

热门标签

最新资料