radon transform.pdf

发布时间：2022-06-08 发布人：admin 分类：说明书资料大小：0.26M 资料格式：pdf 举报版权申诉

xiapeng666-6710711-4744302543293707875.pdf-第1页.png

第1页 / 共10页

xiapeng666-6710711-4744302543293707875.pdf-第2页.png

第2页 / 共10页

xiapeng666-6710711-4744302543293707875.pdf-第3页.png

第3页 / 共10页

xiapeng666-6710711-4744302543293707875.pdf-第4页.png

第4页 / 共10页

xiapeng666-6710711-4744302543293707875.pdf-第5页.png

第5页 / 共10页

xiapeng666-6710711-4744302543293707875.pdf-第6页.png

第6页 / 共10页

xiapeng666-6710711-4744302543293707875.pdf-第7页.png

第7页 / 共10页

xiapeng666-6710711-4744302543293707875.pdf-第8页.png

第8页 / 共10页

文本预览

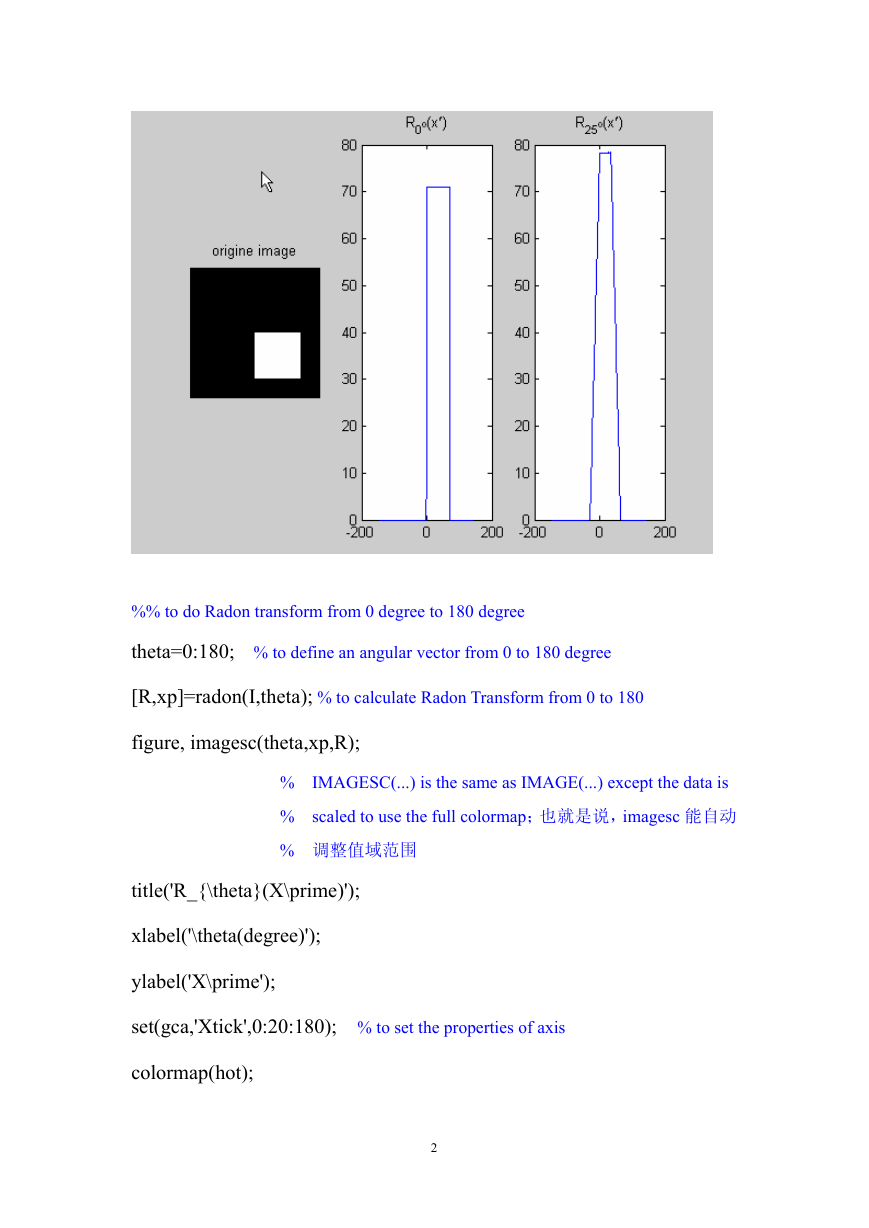
§5.4 Radon 变换 5.4.4 Matlab 中的实现[4] 例、对一个正方形黑框进行 Radon 变换。 I=zeros(200,200); I(100:170,100:170)=1; % to produce a square black frame Figure,imshow(I)； title('origine image')；％％ to do Radon transform of 0 and 25 degree [R,xp]=radon(I,[0 25]); % to calculate the Radon transform of the black frame， % R shows the Radon transform values for theta angles: % 0 and 25 degree; % xp 矢量表示沿 ˆx′ 轴相应的坐标值 subplot(1,3,2),plot(xp,R(:,1)); title('R(0^o)(x\prime)'); subplot(1,3,3),plot(xp,R(:,2)); title('R(25^o)(x\prime)'); 1

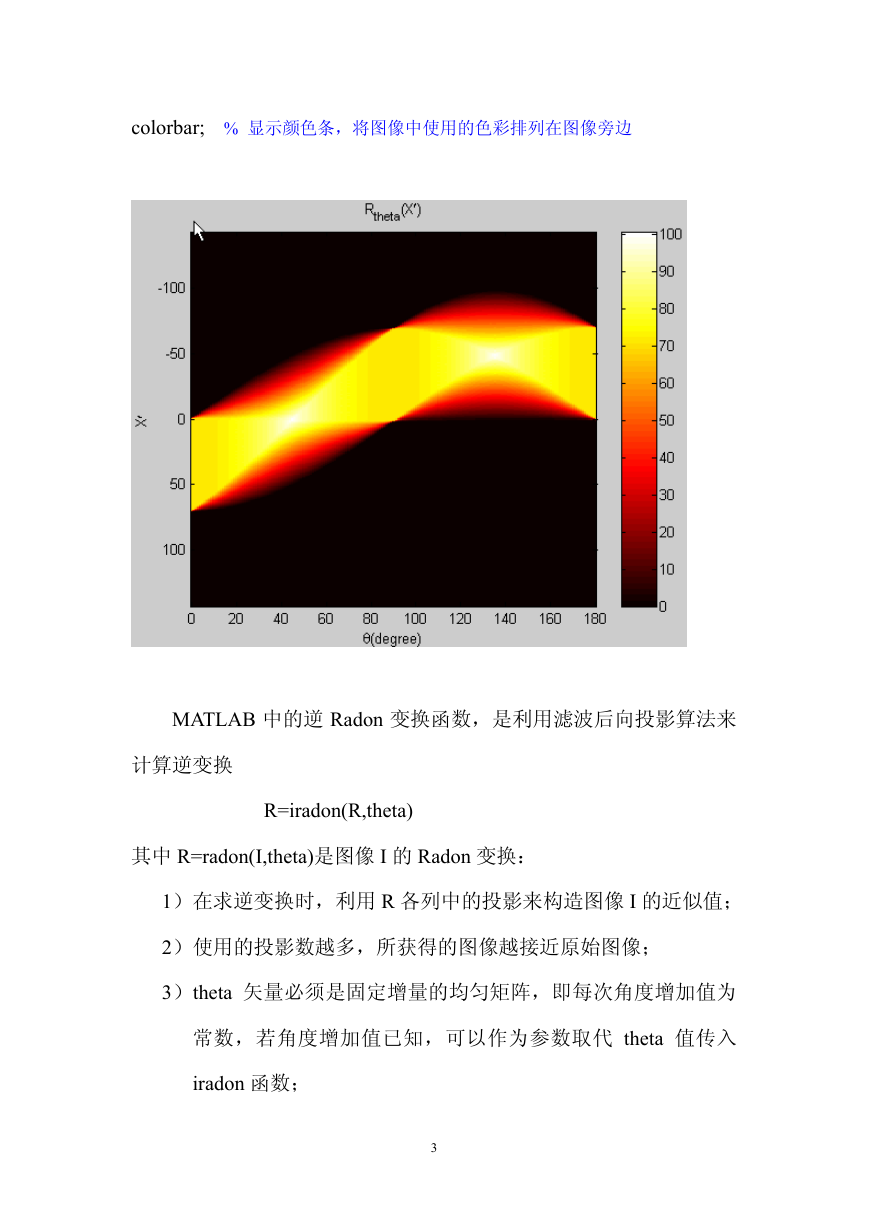
%% to do Radon transform from 0 degree to 180 degree theta=0:180; % to define an angular vector from 0 to 180 degree [R,xp]=radon(I,theta); % to calculate Radon Transform from 0 to 180 figure, imagesc(theta,xp,R); % IMAGESC(...) is the same as IMAGE(...) except the data is % scaled to use the full colormap；也就是说，imagesc 能自动 % 调整值域范围 title('R_{\theta}(X\prime)'); xlabel('\theta(degree)'); ylabel('X\prime'); set(gca,'Xtick',0:20:180); % to set the properties of axis colormap(hot); 2

colorbar; % 显示颜色条，将图像中使用的色彩排列在图像旁边 MATLAB 中的逆 Radon 变换函数，是利用滤波后向投影算法来计算逆变换 R=iradon(R,theta) 其中 R=radon(I,theta)是图像 I 的 Radon 变换： 1）在求逆变换时，利用 R 各列中的投影来构造图像 I 的近似值； 2）使用的投影数越多，所获得的图像越接近原始图像； 3）theta 矢量必须是固定增量的均匀矩阵，即每次角度增加值为常数，若角度增加值已知，可以作为参数取代 theta 值传入 iradon 函数； 3

4）投影值含有噪声时，可以通过加窗消去高频噪声： IR=iradon(R,theta,'Shepp-Logan'); % 采用 Shepp-Logan 窗做滤波： The Shepp-Logan filter multiplies % the Ram-Lak filter by a sinc function. IR=iradon(R,theta,' Cosine'); % 采用 Cosine 窗做滤波：The cosine filter multiplies the Ram-Lak % filter by a cosine function. IR=iradon(R,theta, 'Hamming'); % 采用 Hamming 窗做滤波：The Hamming filter multiplies the % Ram-Lak filter by a Hamming window. IR=iradon(R,theta,0.90); % iradon允许指定归一化频率 D，高于 D 的滤波器响应为零，整 % 个滤波器压缩在[0,D]的范围内。假若系统不含高频信息而存在 % 高频噪声时可用来完全拟制噪声，又不会影响图像重建。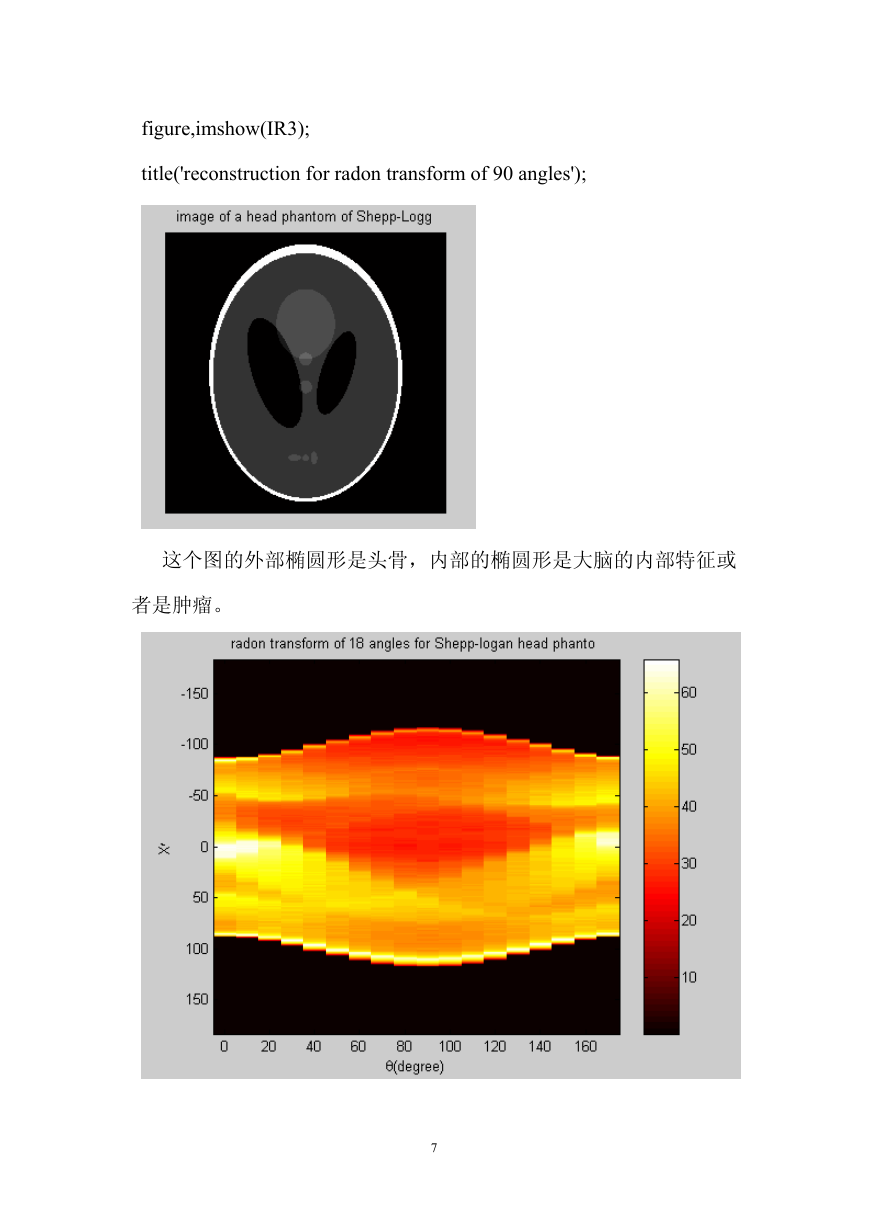例、从一个简单图像的投影重建图像在 Matlab 图像处理工具箱中的 Phantom 函数，可以产生 Shepp －Logan 的大脑图，该图作为一个测试图，可反映人大脑的许多性质。 P=phantom(256); % P = PHANTOM(DEF,N) generates an image of a head phantom that can % be used to test the numerical accuracy of RADON and IRADON or other % 2-D reconstruction algorithms. P is a grayscale intensity image that % consists of one large ellipse (representing the brain) containing % several smaller ellipses (representing features in the brain). figure,imshow(P); title('image of a head phantom of Shepp-Logg'); 4

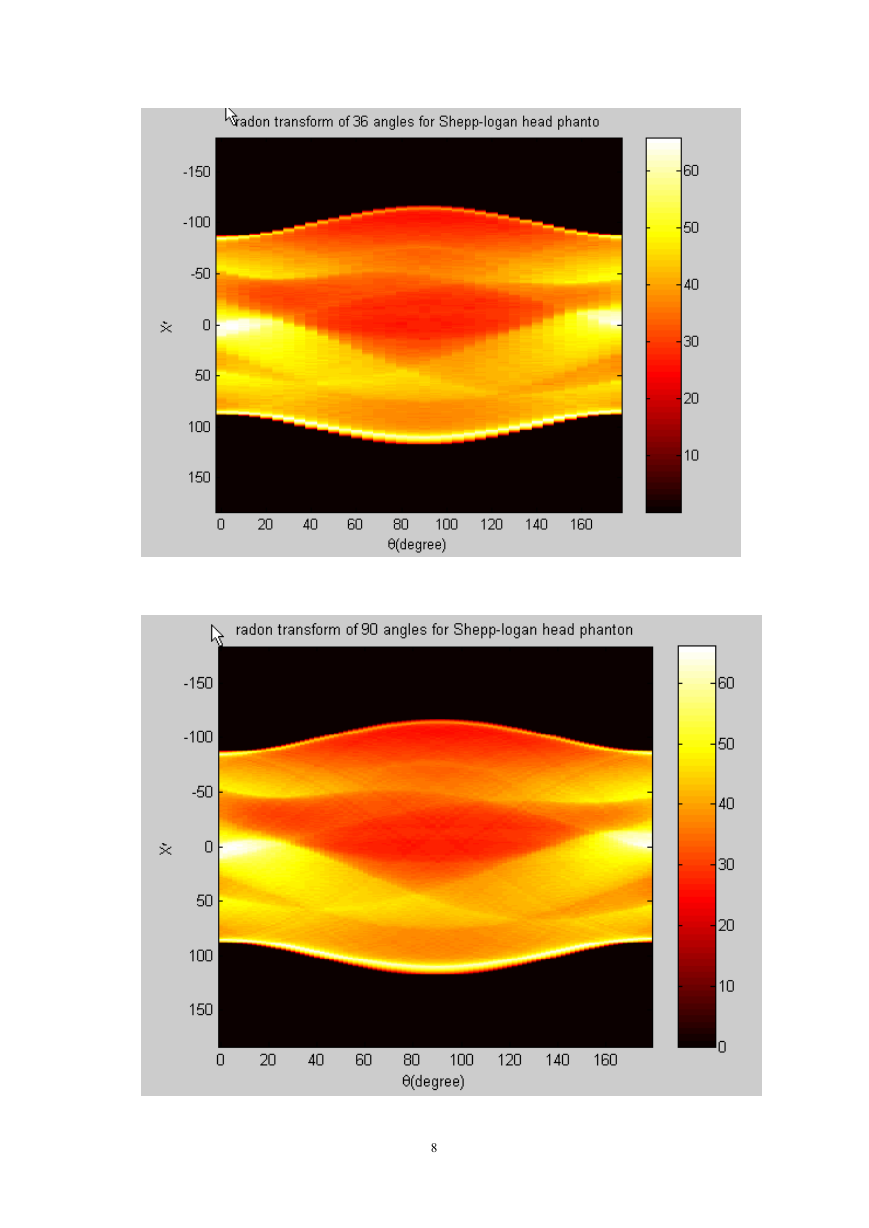
theta1=0:10:170; [R1,xp]=radon(P,theta1); % to calculate radon transform of R1 which includes 18 angles theta2=0:5:175; [R2,xp]=radon(P,theta2); % to calculate radon transform of R2 which includes 36 angles theta3=0:2:178; [R3,xp]=radon(P,theta3); % to calculate radon transform of R3 which includes 90 angles figure,imagesc(theta1,xp,R1); % IMAGESC(...) is the same as IMAGE(...) except the data is % scaled to use the full colormap.title('R_{theta}(X\prime)'); title('radon transform of 18 angles for Shepp-logan head phanto '); xlabel('\theta(degree)'); ylabel('X\prime'); set(gca,'Xtick',0:20:180); % to set the properties of axis colormap(hot); colorbar; figure, imagesc(theta2,xp,R2); 5

title('radon transform of 36 angles for Shepp-logan head phanto'); xlabel('\theta(degree)'); ylabel('X\prime'); set(gca,'Xtick',0:20:180); % to set the properties of axis colormap(hot); colorbar; figure, imagesc(theta3,xp,R3); title('radon transform of 90 angles for Shepp-logan head phanton'); xlabel('\theta(degree)'); ylabel('X\prime'); set(gca,'Xtick',0:20:180); % to set the properties of axis colormap(hot); colorbar; IR1=iradon(R1,theta1,0.85); % 指定归一化频率为 0。85 figure,imshow(IR1); title('reconstruction for radon transform of 18 angles'); IR2=iradon(R2,theta2,0.85); figure,imshow(IR2); title('reconstruction for radon transform of 36 angles'); IR3=iradon(R3,theta3,0.85); 6

figure,imshow(IR3); title('reconstruction for radon transform of 90 angles'); 这个图的外部椭圆形是头骨，内部的椭圆形是大脑的内部特征或者是肿瘤。 7

分享到：

赞收藏

资料库

radon transform.pdf

相关推荐

课程资源

热门标签

最新资料