logo资料库

Discrete Differential Geometry.pdf

发布时间：2022-06-13 发布人：admin 分类：说明书 资料大小：18.84M 资料格式：pdf 举报 版权申诉
第1页 / 共303页
第2页 / 共303页
第3页 / 共303页
第4页 / 共303页
第5页 / 共303页
第6页 / 共303页
第7页 / 共303页
第8页 / 共303页
资料共303页，剩余部分请下载后查看
    1
    Discrete Differential Geometry: An Applied Introduction
    Differential Geometry
    A Bit of History
    Getting Started
    Getting Started
    Discretized
    Discrete Geometry
    Discrete Diff.Geometry
    Discr. Diff. Geometry
    What Matters?
    Warmup: Smooth Setting
    Warmup: Smooth Setting
    Warmup: Smooth Setting
    Warmup: Smooth Setting
    Gauß Map:
    Turning Number
    Turning Number Thm.
    Warmup: Discr. Setting
    Inscribed Polygon:
    Length
    Length
    Total Signed Curvature
    Discrete Gauß Map
    Discrete Gauß Map
    Turning Number Theorem
    Structure-Preservation
    Convergence
    Total Signed Curvature
    Another Definition
    Curvature normal
    Gradient of Length
    Gradient of Length
    Gradient of Length
    Gradient of Length
    Gradient of Length
    Gradient of Length
    Gradient of Length
    Moral of the Story
    Themes for Today
    Themes for Today
    What it All Means
    The Program for Today
    The Program for Today
    The Program for Today
    2
    3
    4
    5
    6
    Three Points Make a Triangle… Or a Circle
    In This Section
    Geometries
    Conformal Mappings
    Paramaterizations
    Conformal Maps
    Conformal Maps
    Conformal Maps
    Sounds Great!
    Sounds Great!
    An Old Idea
    An Old Idea
    An Old Idea
    An Old Idea
    History
    Basic Setup
    Circle Pattern Problem
    Circle Pattern Problem
    Circle Pattern Problem
    Geometry at an Edge
    Energy
    Algorithm
    Results
    Boundary Control
    Quasi-Conformal Distr.
    Quasi-Conformal Distr.
    Robustness
    Problems
    Piecewise Flat
    Cone Singularities
    Examples
    Examples
    Properties
    Properties
    Properties
    Summary
    Software
    More Fun with Circles
    More Fun with Circles
    More Fun with Circles
    More Fun with Circles
    Disc. Willmore Energy
    Properties
    Properties
    Properties
    Properties I
    Properties I
    Results I
    Results II
    Results III
    Results III
    Results III
    Results III
    Results III
    Results III
    Results III
    Results III
    Results III
    Results III
    Results IV
    Summary
    Circle Summary
    7
    Discrete Differential Geometry: An Applied Introduction Eitan Grinspun with Mathieu Desbrun, Konrad Polthier, Peter Schröder, & Ari Stern DDG Course SIGGRAPH 2006 1
    Differential Geometry Why do we care? geometry of surfaces mothertongue of physical theories Springborn Grape (u. of Bonn) computation: simulation/processing Elcott et al. Alliez et al. Grinspun et al. Desbrun DDG Course SIGGRAPH 2006 2
    A Bit of History Geometry is the key! studied for centuries Hermann Schwarz, 1890 DiMarco, Physics, Montana Cartan, Poincaré, Lie, Hodge, de Rham, Gauss, Noether,… mostly differential geometry differential and integral calculus The study of invariants and symmetries DDG Course SIGGRAPH 2006 Bobenko and Suris 3
    Getting Started How to apply DiffGeo ideas? surfaces as collections of samples and topology (connectivity) apply continuous ideas BUT: setting is discrete what is the right way? discrete vs. discretized DDG Course SIGGRAPH 2006 4
    Getting Started How to apply DiffGeo ideas? surfaces as collections of samples and topology (connectivity) apply continuous ideas BUT: setting is discrete what is the right way? discrete vs. discretized DDG Course SIGGRAPH 2006 5
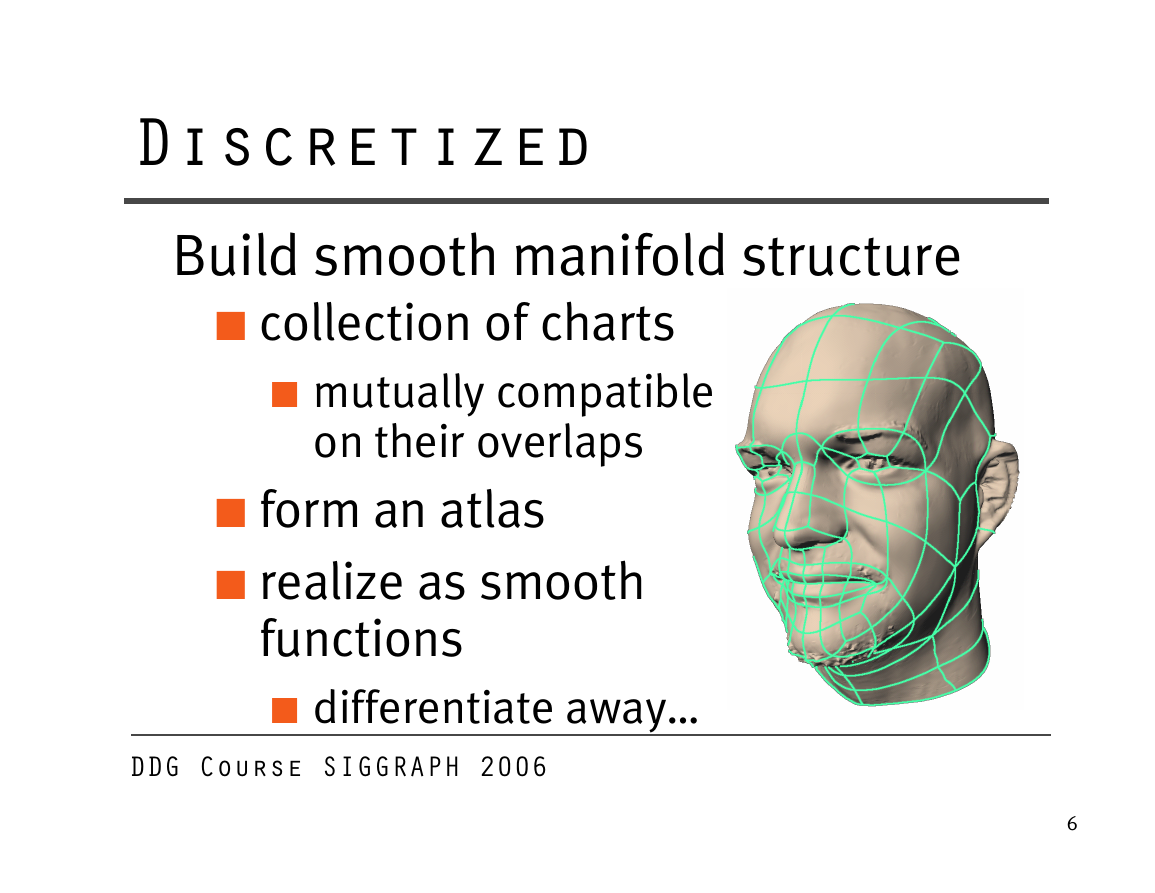
    Discretized Build smooth manifold structure collection of charts mutually compatible on their overlaps form an atlas realize as smooth functions differentiate away… DDG Course SIGGRAPH 2006 6
    Discrete Geometry Basic tool differential geometry metric, curvature, etc. Discrete realizations “meshes” computational geom. graph theory DDG Course SIGGRAPH 2006 Hermann Schwarz, 1890 DiMarco, Physics, Montana Uli Heller, 2002 Boy’s Surface, Oberwolfach Black Rock City, 2003 Frei Otto, Munich 1968 7
    Discrete Diff.Geometry Building from the ground up discrete geometry given meshes: triangles, tets more general: cell complex how to do calculus? DDG Course SIGGRAPH 2006 8
    分享到：
    赞 收藏

    相关推荐

    资料库

    课程资源

    共收录17145份资料，累计13个分类，关注成员有19位，主要包括：PHP,网络管理,网页制作,Java,.Net,数据库,3G/移动开发,C/C++,游戏开发,嵌入式,讲义,软件测试,专业指导

    热门标签

    PHP 网络管理 网页制作 Java .Net 数据库 3G/移动开发 C/C++ 游戏开发 嵌入式 讲义 软件测试 专业指导

    最新资料