Ansoft_Metamaterial_DesignGuide.pdf

发布时间：2022-06-08 发布人：admin 分类：说明书资料大小：2.81M 资料格式：pdf 举报版权申诉

qq_28542831-8746551-4744302543378311592.pdf-第1页.png

第1页 / 共55页

qq_28542831-8746551-4744302543378311592.pdf-第2页.png

第2页 / 共55页

qq_28542831-8746551-4744302543378311592.pdf-第3页.png

第3页 / 共55页

qq_28542831-8746551-4744302543378311592.pdf-第4页.png

第4页 / 共55页

qq_28542831-8746551-4744302543378311592.pdf-第5页.png

第5页 / 共55页

qq_28542831-8746551-4744302543378311592.pdf-第6页.png

第6页 / 共55页

qq_28542831-8746551-4744302543378311592.pdf-第7页.png

第7页 / 共55页

qq_28542831-8746551-4744302543378311592.pdf-第8页.png

第8页 / 共55页

文本预览

Left-Handed Metamaterial Design Using Ansoft Designer and HFSS by Anthony Lai Ansoft Corporation Draft Copy August 2007

Abstract This paper provides a design guide for left-handed metamaterials using Ansoft Designer (Method of Moments) and Ansoft HFSS (Finite Element Method). Detailed discussion about the de- sign of the left-handed metamaterial unit-cells along with detailed setup for analysis of these unit-cells in Ansoft Designer and HFSS are presented. In addition, a leaky-wave antenna and a negative refractive index ﬂat lens based on the analyzed left-handed unit-cells are simulated in Designer and HFSS. In Chapter 1, the concept of metamaterials is explained and the following questions are ex- amined: “What are left-handed metamaterials?,” “Why are left-handed metamaterials impor- tant?,” and “How are left-handed metamaterials realized?” Chapter 2 provides a design guide for a one-dimensional, dominant-mode leaky-wave antenna based on the left-handed metamaterial’s unique dispersion characteristics. This chapter begins with a brief introduction on leaky-wave antennas and discusses the advantages of a left-handed metamaterial implementation over a conventional implementation of the antenna. The chapter continues with the design of a dominant-mode leaky-wave antenna using Ansoft Designer. The analysis of the required one-dimensional left-handed metamaterial unit-cell based on microstrip technology is presented in detail; unit-cell setup, analysis options, dispersion diagram genera- tion, and Bloch impedance diagram generation using Ansoft Designer are outlined. Next, the designed unit-cell is cascaded to form a leaky-wave antenna. S-parameter and far-ﬁeld patterns are generated using Ansoft Designer to verify the dominant mode backﬁre to endﬁre frequency scanning capability of the realized antenna. Chapter 3 deals with the analysis and design of two-dimensional left-handed metamaterials. A negative refractive index ﬂat lens is realized in Ansoft HFSS based on the Sievenpiper mush- room unit-cell. First, the two-dimensional unit-cell is discussed and its dispersion diagram is generated using two approaches; driven mode like Chapter 1 and eigenmode solver provided in Ansoft HFSS. The dispersion diagram is used to characterize the refractive index of the left-handed metamaterial and to determine the operational frequency for the lens application. Then, the unit-cell is cascaded in two-dimensions in order to form a bulk left-handed metama- terial. The HFSS setup for the lens simulation is discussed; ﬁeld plots are used to conﬁrm the negative refraction in the lens. In addition, the HFSS ﬁeld calculator is used to plot the phase of the electric ﬁeld in the lens.

Contents 1 Left-Handed Metamaterials 1.1 What Are Left-Handed Metamaterials? . . . . . . . . . . . . . . . . . . . . . . 1.1.1 Backward Waves . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 1.1.2 Negative Refractive Index . . . . . . . . . . . . . . . . . . . . . . . . . . 1.1.3 Frequency Dispersion . . . . . . . . . . . . . . . . . . . . . . . . . . . . 1.2 Why Are Left-Handed Metamaterials Important? . . . . . . . . . . . . . . . . . 1.3 How Are Left-Handed Metamaterials Realized? . . . . . . . . . . . . . . . . . . 1.3.1 Transmission Line Approach . . . . . . . . . . . . . . . . . . . . . . . . 1.3.2 Composite Right/Left-Handed Transmission Line . . . . . . . . . . . . . 1.4 Physical Realization and Analysis: Dispersion and Bloch Impedance Diagrams 1.4.1 Dispersion Diagram . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 1.4.2 Bloch Impedance Diagram . . . . . . . . . . . . . . . . . . . . . . . . . . 1.5 Summary . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 2 Ansoft Designer: Dominant-Mode Leaky-Wave Antenna 2.1 2.2 One-Dimensional CRLH Unit-Cell Introduction . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 2.2.1 Design Guidelines - Initial Parameter Values . . . . . . . . . . . . . . . 2.2.2 Dispersion Diagram Extraction . . . . . . . . . . . . . . . . . . . . . . . 2.2.3 1st Variation . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 2.2.4 2nd Variation . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 2.2.5 Final Variation . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 2.2.6 Bloch Impedance Extraction . . . . . . . . . . . . . . . . . . . . . . . . 2.3 Leaky-Wave Antenna Design . . . . . . . . . . . . . . . . . . . . . . . . . . . . 2.3.1 Feed Network Design . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 2.3.2 Ansoft Designer Analysis . . . . . . . . . . . . . . . . . . . . . . . . . . S-Parameter and Far-Field Results . . . . . . . . . . . . . . . . . . . . . 2.3.3 2.4 Summary . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 3 Ansoft HFSS: Negative Refractive Flat Lens 3.1 3.2 Two-Dimensional CRLH Unit-Cell Introduction . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 3.2.1 Design: 1st Order Approximation . . . . . . . . . . . . . . . . . . . . . . 3.2.2 Design: Driven Mode Dispersion Diagram . . . . . . . . . . . . . . . . . 3.2.3 Veriﬁcation: Eigenmode Dispersion Diagram . . . . . . . . . . . . . . . Eigenmode Solver Setup . . . . . . . . . . . . . . . . . . . . . . . . . . . Linked Boundary Conditions . . . . . . . . . . . . . . . . . . . . . . . . Eigenmode Analysis Setup . . . . . . . . . . . . . . . . . . . . . . . . . . Dependent Solve Setup . . . . . . . . . . . . . . . . . . . . . . . . . . . 3 3 4 4 5 5 6 6 8 9 10 10 11 15 15 16 17 18 22 24 24 25 26 26 27 28 32 34 34 36 36 37 39 39 40 42 43 1

CONTENTS Driven Mode versus Eigen Mode Dispersion Diagram . . . . . . . . . . . 3.3 Flat Lens Simulation . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 3.3.1 Phase Matching Condition . . . . . . . . . . . . . . . . . . . . . . . . . 3.3.2 HFSS Model Setup . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 3.3.3 Analysis and Results . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 3.4 Summary . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 2 46 48 48 48 50 52

Chapter 1 Left-Handed Metamaterials 1.1 What Are Left-Handed Metamaterials? Electromagnetic metamaterials are eﬀectively homogeneous artiﬁcial structures engineered to provide electromagnetic properties not readily observable in nature. Eﬀectively homogeneous means that scattering/diﬀraction is a minor/non-existent eﬀect for a propagating wave in a metamaterial; lattice constants of the metamaterial are much smaller than the electromagnetic wave. So what kind of unique electromagnetic properties can metamaterials provide? First, lets deﬁne what we mean by electromagnetic properties. 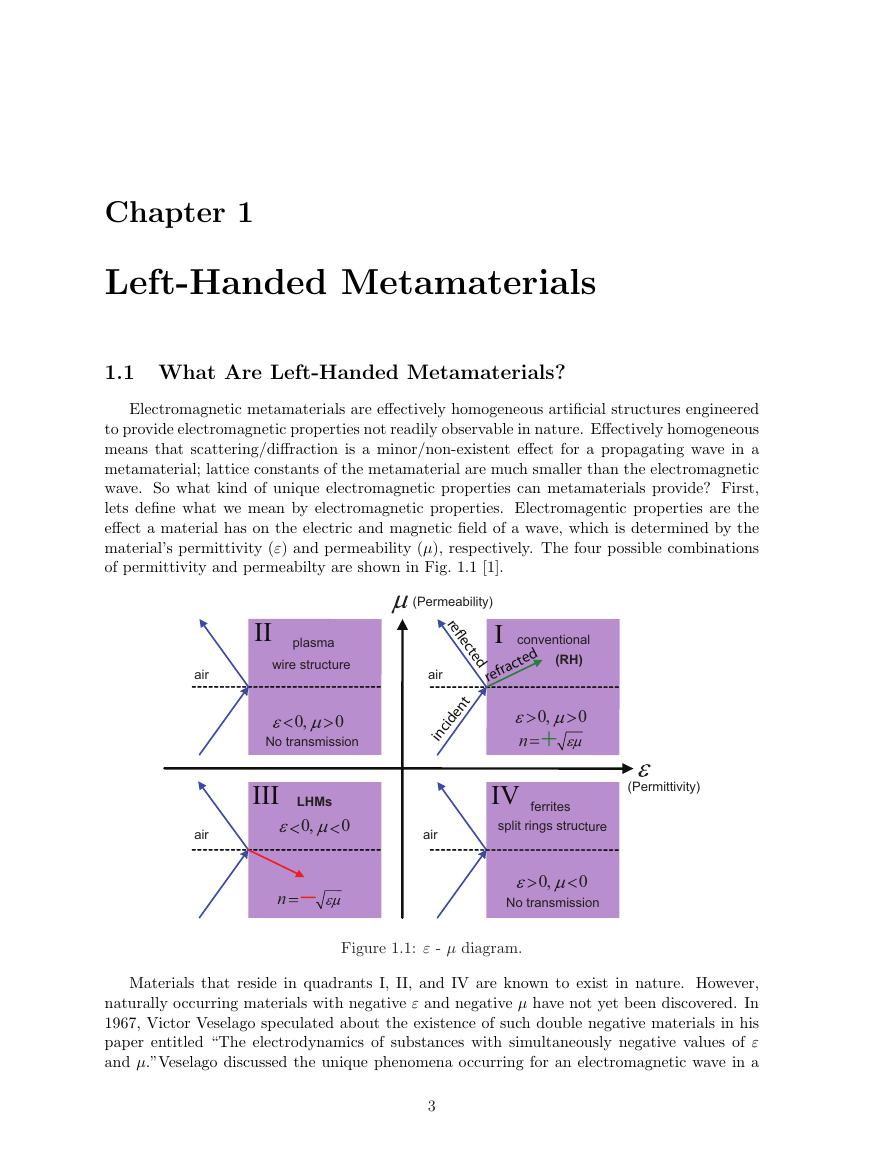Electromagentic properties are the eﬀect a material has on the electric and magnetic ﬁeld of a wave, which is determined by the material’s permittivity (ε) and permeability (µ), respectively. The four possible combinations of permittivity and permeabilty are shown in Fig. 1.1 [1]. Figure 1.1: ε - µ diagram. Materials that reside in quadrants I, II, and IV are known to exist in nature. However, naturally occurring materials with negative ε and negative µ have not yet been discovered. In 1967, Victor Veselago speculated about the existence of such double negative materials in his paper entitled “The electrodynamics of substances with simultaneously negative values of ε and µ.”Veselago discussed the unique phenomena occurring for an electromagnetic wave in a 3 conventionalplasmawire structure split rings structureferritesLHMs0,0n0,00,00,0No transmissionNo transmissionn(RH)airairairair(Permittivity)(Permeability)IIIIIIIVincidentrefractedre ected

CHAPTER 1. LEFT-HANDED METAMATERIALS 4 double negative material: 1. Electric ﬁeld, magnetic ﬁeld, and wavevector form a left-handed (LH) triad. 2. Negative refractive index leads to reversal of Snell’s Law, Doppler Eﬀect, and Vavilov- Cerenkov radiation. 3. Frequency dispersion. 1.1.1 Backward Waves Since an electromagnetic wave in a double negative material forms a LH triad, double negative materials are generally referred to as LH materials. The LH triad means that power ﬂows away from the source (group velocity is positive) while the phase front travels towards the source (phase velocity is negative). Therefore, LH materials support backward waves: wave with anti-parallel group and phase velocities. This backward wave phenomenon can be observed in Fig. 1.2, which shows the electric ﬁeld magnitude plot of an air-ﬁlled rectangular waveguide with its middle section ﬁlled with a ﬁctional LH material of εr=-1 and µr=-1. The magnitude plot shows that power is transfered from the input to the output of the waveguide and that the phase front travels backwards. Figure 1.2: Eﬀective medium LH material simulation [2] in Ansoft HFSS. Rectangular waveg- uide with middle section ﬁlled with LH material (εr=-1, µr=-1); backward wave can be observed by observing phase front for diﬀerent phases. 1.1.2 Negative Refractive Index Since ε and µ are negative, the refractive index of a LH material is negative1: 1The sign of the refractive index is usually taken as positive. However, Veselago showed that if a medium has both negative permittivity and negative permeability, the negative sign must be taken. vpvpvpPinPoutbackward wave (vp= -vg)ε<0, μ<0ε>0, μ>0ε>0, μ>0vpvpvpvgvgvgθ=0°θ=45°θ=90°θ=135°θ=180°vpvpvpPinPoutbackward wave (vp= -vg)ε<0, μ<0ε>0, μ>0ε>0, μ>0vpvpvpvgvgvgθ=0°θ=45°θ=90°θ=135°θ=180°RHLHRH

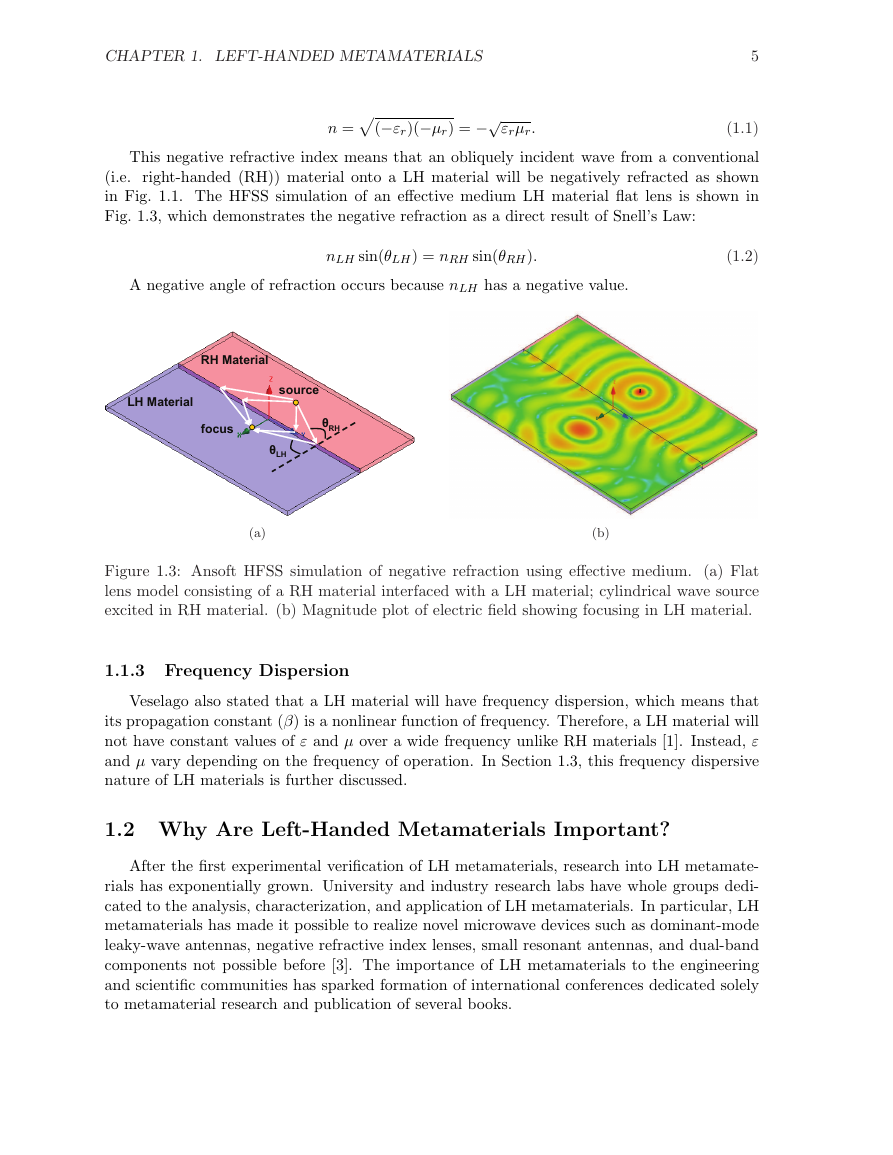
CHAPTER 1. LEFT-HANDED METAMATERIALS (−εr)(−µr) = −√ εrµr. n = 5 (1.1) This negative refractive index means that an obliquely incident wave from a conventional (i.e. right-handed (RH)) material onto a LH material will be negatively refracted as shown in Fig. 1.1. The HFSS simulation of an eﬀective medium LH material ﬂat lens is shown in Fig. 1.3, which demonstrates the negative refraction as a direct result of Snell’s Law: A negative angle of refraction occurs because nLH has a negative value. nLH sin(θLH) = nRH sin(θRH). (1.2) (a) (b) Figure 1.3: Ansoft HFSS simulation of negative refraction using eﬀective medium. (a) Flat lens model consisting of a RH material interfaced with a LH material; cylindrical wave source excited in RH material. (b) Magnitude plot of electric ﬁeld showing focusing in LH material. 1.1.3 Frequency Dispersion Veselago also stated that a LH material will have frequency dispersion, which means that its propagation constant (β) is a nonlinear function of frequency. Therefore, a LH material will not have constant values of ε and µ over a wide frequency unlike RH materials [1]. Instead, ε and µ vary depending on the frequency of operation. In Section 1.3, this frequency dispersive nature of LH materials is further discussed. 1.2 Why Are Left-Handed Metamaterials Important? After the ﬁrst experimental veriﬁcation of LH metamaterials, research into LH metamate- rials has exponentially grown. University and industry research labs have whole groups dedi- cated to the analysis, characterization, and application of LH metamaterials. In particular, LH metamaterials has made it possible to realize novel microwave devices such as dominant-mode leaky-wave antennas, negative refractive index lenses, small resonant antennas, and dual-band components not possible before [3]. The importance of LH metamaterials to the engineering and scientiﬁc communities has sparked formation of international conferences dedicated solely to metamaterial research and publication of several books. θRHθLHsourcefocusRH MaterialLH MaterialθRHθLHsourcefocusRH MaterialLH Material

CHAPTER 1. LEFT-HANDED METAMATERIALS 6 1.3 How Are Left-Handed Metamaterials Realized? In his paper, Veselago also stated that although LH materials do not exist in nature, they can be artiﬁcially constructed. In particular, Veselago concluded that the realization of a LH metamaterial will be possible with the discovery or construction of an isotropic negative µ material. When Veselago published his paper, materials with µ < 0 were not known to exist. For 30 years, Veselago’s paper and its theory was not investigated any further. Interest in Veselago’s paper and LH materials begin to materialize when Professor Pendry at Imperial College demonstrated the ﬁrst non-ferrite negative µ metamaterial based on split ring resonators (SRRs) in 1998 [4]. 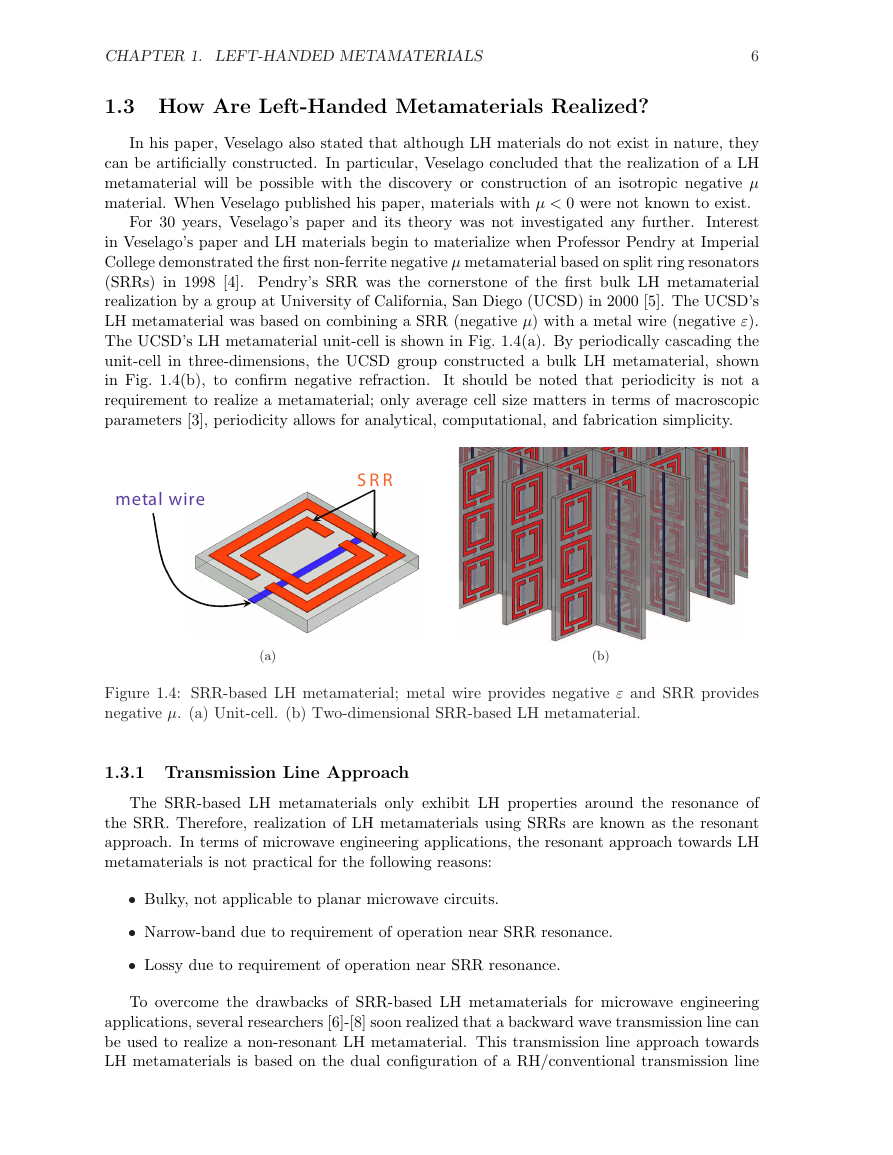Pendry’s SRR was the cornerstone of the ﬁrst bulk LH metamaterial realization by a group at University of California, San Diego (UCSD) in 2000 [5]. The UCSD’s LH metamaterial was based on combining a SRR (negative µ) with a metal wire (negative ε). The UCSD’s LH metamaterial unit-cell is shown in Fig. 1.4(a). By periodically cascading the unit-cell in three-dimensions, the UCSD group constructed a bulk LH metamaterial, shown in Fig. 1.4(b), to conﬁrm negative refraction. It should be noted that periodicity is not a requirement to realize a metamaterial; only average cell size matters in terms of macroscopic parameters [3], periodicity allows for analytical, computational, and fabrication simplicity. (a) (b) Figure 1.4: SRR-based LH metamaterial; metal wire provides negative ε and SRR provides negative µ. (a) Unit-cell. (b) Two-dimensional SRR-based LH metamaterial. 1.3.1 Transmission Line Approach The SRR-based LH metamaterials only exhibit LH properties around the resonance of the SRR. Therefore, realization of LH metamaterials using SRRs are known as the resonant approach. In terms of microwave engineering applications, the resonant approach towards LH metamaterials is not practical for the following reasons: X Bulky, not applicable to planar microwave circuits. X Narrow-band due to requirement of operation near SRR resonance. X Lossy due to requirement of operation near SRR resonance. To overcome the drawbacks of SRR-based LH metamaterials for microwave engineering applications, several researchers [6]-[8] soon realized that a backward wave transmission line can be used to realize a non-resonant LH metamaterial. This transmission line approach towards LH metamaterials is based on the dual conﬁguration of a RH/conventional transmission line SRR metal wire

分享到：

赞收藏

资料库

Ansoft_Metamaterial_DesignGuide.pdf

相关推荐

课程资源

热门标签

最新资料