第1页 / 共107页
第2页 / 共107页
第3页 / 共107页
第4页 / 共107页
第5页 / 共107页
第6页 / 共107页
第7页 / 共107页
第8页 / 共107页
Functional Analysis Notes (2011) Mr. Andrew Pinchuck.pdf
FUNCTIONAL ANALYSIS NOTES
(2011)
Mr. Andrew Pinchuck
Department of Mathematics (Pure & Applied)
Rhodes University
Contents
Introduction
1 Linear Spaces
Introducton . . . .
. . . . . . .
1.1
. . . . . . . .
. . . . . . .
1.2 Subsets of a linear space . . . . .
. . . . . . .
1.3 Subspaces and Convex Sets . . . .
. . . . . . .
1.4 Quotient Space . .
. . . . . . . .
1.5 Direct Sums and Projections . . .
. . . . . . .
1.6 The H¨older and Minkowski Inequalities . . . .
. . . . . . . .
. . . . . . . .
. . . . . . . .
. . . . . . . .
. . . . . . . .
. . . . . . . .
. . . . . . .
. . . . . . .
. . . . . . .
. . . . . . .
. . . . . . .
. . . . . . .
. . . . . . . .
. . . . . . . .
. . . . . . . .
. . . . . . . .
. . . . . . . .
. . . . . . . .
2 Normed Linear Spaces
. . .
. . . . . . . .
. . . . . . . .
2.1 Preliminaries
. . . . . . .
. . . . . . . .
2.2 Quotient Norm and Quotient Map . . . . . . .
. . . . . . . .
2.3 Completeness of Normed Linear Spaces . . . .
2.4 Series in Normed Linear Spaces
. . . . . . . .
. . . . . . .
2.5 Bounded, Totally Bounded, and Compact Subsets of a Normed Linear Space . . . . . . .
. . . . . . . .
2.6 Finite Dimensional Normed Linear Spaces . . .
2.7 Separable Spaces and Schauder Bases . . . . .
. . . . . . . .
. . . . . . . .
. . . . . . . .
. . . . . . . .
. . . . . . . .
. . . . . . .
. . . . . . .
. . . . . . .
. . . . . . .
. . . . . . . .
. . . . . . . .
. . . . . . .
. . . . . . .
.
3 Hilbert Spaces
. . . . . . . .
Introduction . . . .
3.1
. . . . . . .
3.2 Completeness of Inner Product Spaces . . . . .
3.3 Orthogonality . . .
. . . . . . .
3.4 Best Approximation in Hilbert Spaces . . . . .
3.5 Orthonormal Sets and Orthonormal Bases . . .
. . . . . . . .
4 Bounded Linear Operators and Functionals
Introduction . . . .
4.1
. . . . . . . .
4.2 Examples of Dual Spaces . . . . .
4.3
. . . . . . .
. . . . . . .
The Dual Space of a Hilbert Space . . . . . . .
. . . . . . . .
. . . . . . . .
. . . . . . . .
. . . . . . . .
. . . . . . . .
. . . . . . .
. . . . . . .
. . . . . . .
. . . . . . .
. . . . . . .
. . . . . . . .
. . . . . . . .
. . . . . . . .
. . . . . . . .
. . . . . . . .
. . . . . . . .
. . . . . . . .
. . . . . . . .
. . . . . . .
. . . . . . .
. . . . . . .
. . . . . . . .
. . . . . . . .
. . . . . . . .
5 The Hahn-Banach Theorem and its Consequences
. . . . . . .
Introduction . . . .
. . . . . . . .
5.1
. . . . . . . .
5.2 Consequences of the Hahn-Banach Extension Theorem . . . . . . . . . . . . . . . . . . .
5.3 Bidual of a normed linear space and Reflexivity . . . . . . . .
. . . . . . . .
. . . . . . . .
. . . . . . . .
5.4 The Adjoint Operator . . . . . . .
5.5 Weak Topologies .
. . . . . . . .
. . . . . . . .
. . . . . . . .
. . . . . . .
. . . . . . .
. . . . . . .
. . . . . . .
. . . . . . .
. . . . . . . .
. . . . . . .
1
2
2
5
5
7
8
9
13
13
18
19
24
26
28
32
36
36
42
42
45
49
62
62
72
77
81
81
85
88
90
91
1
2011
FUNCTIONAL ANALYSIS
ALP
6 Baire’s Category Theorem and its Applications
Introduction . . . .
6.1
. . . . . . . .
6.2 Uniform Boundedness Principle .
6.3 The Open Mapping Theorem . . .
6.4 Closed Graph Theorem . . . . . .
. . . . . . .
. . . . . . .
. . . . . . .
. . . . . . .
. . . . . . . .
. . . . . . . .
. . . . . . . .
. . . . . . . .
. . . . . . .
. . . . . . .
. . . . . . .
. . . . . . .
99
. . . . . . . .
99
. . . . . . . . 101
. . . . . . . . 102
. . . . . . . . 104
2
2011
FUNCTIONAL ANALYSIS
ALP
Introduction
These course notes are adapted from the original course notes written by Prof. Sizwe Mabizela when
he last gave this course in 2006 to whom I am indebted. I thus make no claims of originality but have made
several changes throughout. In particular, I have attempted to motivate these results in terms of applications
in science and in other important branches of mathematics.
Functional analysis is the branch of mathematics, specifically of analysis, concerned with the study of
vector spaces and operators acting on them. It is essentially where linear algebra meets analysis. That is,
an important part of functional analysis is the study of vector spaces endowed with topological structure.
Functional analysis arose in the study of tansformations of functions, such as the Fourier transform, and in
the study of differential and integral equations. The founding and early development of functional analysis
is largely due to a group of Polish mathematicians around Stefan Banach in the first half of the 20th century
but continues to be an area of intensive research to this day. Functional analysis has its main applications in
differential equations, probability theory, quantum mechanics and measure theory amongst other areas and
can best be viewed as a powerful collection of tools that have far reaching consequences.
As a prerequisite for this course, the reader must be familiar with linear algebra up to the level of a
standard second year university course and be familiar with real analysis. The aim of this course is to
introduce the student to the key ideas of functional analysis. It should be remembered however that we
only scratch the surface of this vast area in this course. We examine normed linear spaces, Hilbert spaces,
bounded linear operators, dual spaces and the most famous and important results in functional analysis
such as the Hahn-Banach theorem, Baires category theorem, the uniform boundedness principle, the open
mapping theorem and the closed graph theorem. We attempt to give justifications and motivations for the
ideas developed as we go along.
Throughout the notes, you will notice that there are exercises and it is up to the student to work through
these. In certain cases, there are statements made without justification and once again it is up to the student
to rigourously verify these results. For further reading on these topics the reader is referred to the following
texts:
G. BACHMAN, L. NARICI, Functional Analysis, Academic Press, N.Y. 1966.
E. KREYSZIG, Introductory Functional Analysis, John Wiley & sons, New York-Chichester-Brisbane-
Toronto, 1978.
G. F. SIMMONS, Introduction to topology and modern analysis, McGraw-Hill Book Company, Sin-
gapore, 1963.
A. E. TAYLOR, Introduction to Functional Analysis, John Wiley & Sons, N. Y. 1958.
I have also found Wikipedia to be quite useful as a general reference.
1
Chapter 1
Linear Spaces
1.1
Introducton
In this first chapter we review the important notions associated with vector spaces. We also state and prove
some well known inequalities that will have important consequences in the following chapter.
Unless otherwise stated, we shall denote by R the field of real numbers and by C the field of complex
numbers. Let F denote either R or C.
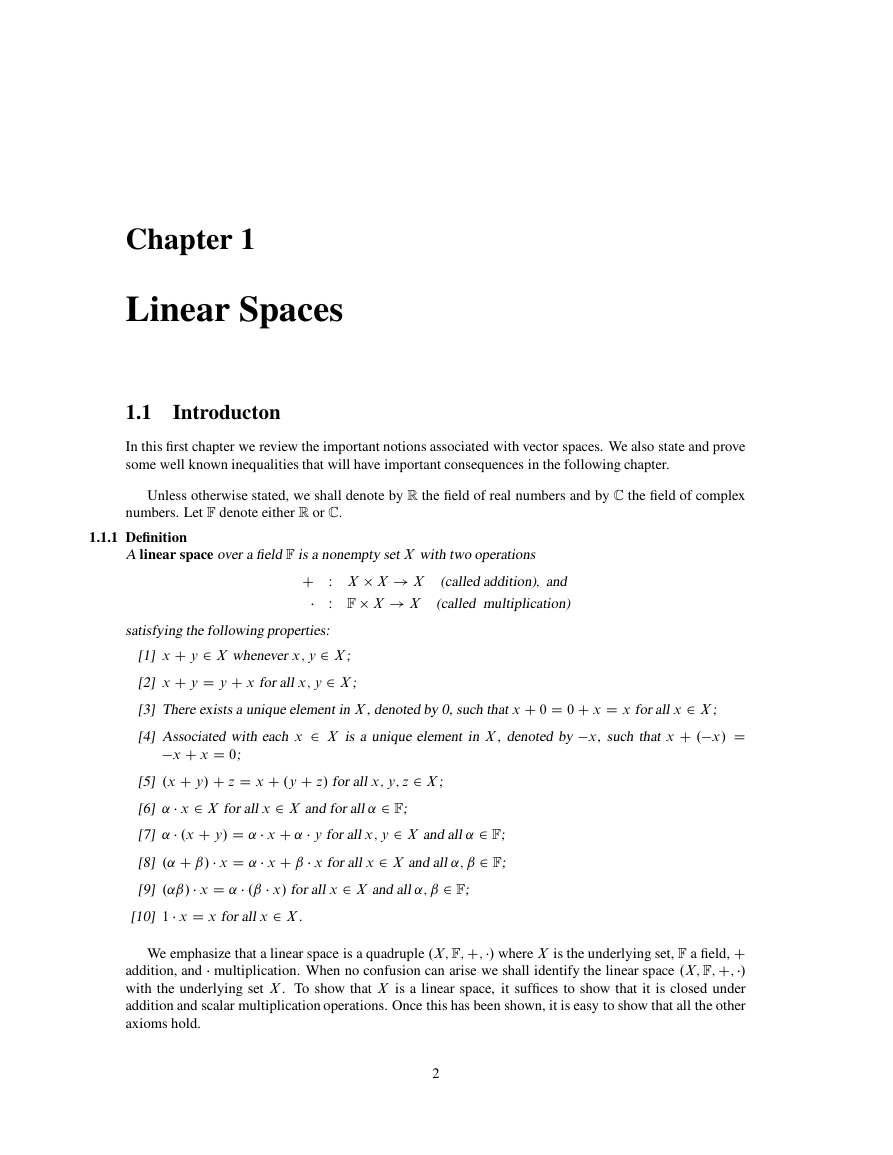
1.1.1 Definition
A linear space over afield F isa nonempty set X with twooperations
C W X X ! X (called addition); and
W F X ! X (called multiplication)
satisfyingthefollowingproperties:
[1] x C y 2 X whenever x; y 2 X ;
[2] x C y D y C x for all x; y 2 X ;
[3] There existsa uniqueelement in X , denotedby 0, such that x C 0 D 0 C x D x forall x 2 X ;
[4] Associated with each x 2 X is a unique element in X , denoted by x, such that x C .x/ D
x C x D 0;
[5] .x C y/ C z D x C .y C z/ for all x; y; z 2 X ;
[6] ˛ x 2 X for all x 2 X and forall ˛ 2 F;
[7] ˛ .x C y/ D ˛ x C ˛ y for all x; y 2 X and all ˛ 2 F;
[8] .˛ C ˇ/ x D ˛ x C ˇ x for all x 2 X and all ˛; ˇ 2 F;
[9] .˛ˇ/ x D ˛ .ˇ x/ for all x 2 X and all ˛; ˇ 2 F;
[10] 1 x D x for all x 2 X .
We emphasize that a linear space is a quadruple .X; F; C; / where X is the underlying set, F a field, C
addition, and multiplication. When no confusion can arise we shall identify the linear space .X; F; C; /
with the underlying set X . To show that X is a linear space, it suffices to show that it is closed under
addition and scalar multiplication operations. Once this has been shown, it is easy to show that all the other
axioms hold.
2
2011
FUNCTIONAL ANALYSIS
ALP
1.1.2 Definition
A real (resp. complex) linear space isalinear space over thereal (resp. complex) field.
A linear space is also called a vector space and its elements are called vectors.
1.1.3 Examples
[1] For a fixed positive integer n, let X D Fn D fx D .x1; x2;
: : : ; xn/ W xi 2 F; i D
1; 2; : : : ; ng – the set of all n-tuples of real or complex numbers. Define the operations
of addition and scalar multiplication pointwise as follows: For all x D .x1; x2;
y D .y1; y2; : : : ; yn/ in Fn and ˛ 2 F,
: : : ; xn/;
x C y D .x1 C y1; x2 C y2; : : : ; xn C yn/
˛ x D .˛x1; ˛x2; : : : ; ˛xn/:
Then Fn is a linear space over F.
[2] Let X D CŒa; b D f x W Œa; b ! F j x is continuous g. Define the operations of addition and
scalar multiplication pointwise: For all x; y 2 X and all ˛ 2 R, define
.x C y/.t/ D x.t/ C y.t/ and
.˛ x/.t/ D ˛x.t/
Then CŒa; b is a real vector space.
for all t 2 Œa; b:
Sequence Spaces: Informally, a sequence in X is a list of numbers indexed by N. Equivalently,
a sequence in X is a function x W N ! X given by n 7! x.n/ D xn. We shall denote a
sequence x1; x2; : : : by
x D .x1; x2; : : :/ D .xn/11 :
[3] The sequence space s. Let s denote the set of all sequences x D .xn/11 of real or complex
numbers. Define the operations of addition and scalar multiplication pointwise: For all x D
.x1; x2; : : :/, y D .y1; y2; : : :/ 2 s and all ˛ 2 F, define
x C y D .x1 C y1; x2 C y2; : : :/
˛ x D .˛x1; ˛x2; : : :/:
Then s is a linear space over F.
[4] The sequence space `1. Let `1 D `1.N/ denote the set of all bounded sequences of real or
complex numbers. That is, all sequences x D .xn/11 such that
Define the operations of addition and scalar multiplication pointwise as in example (3). Then
`1 is a linear space over F.
sup
i2N jxij < 1:
[5] The sequence space `p D `p.N/;
1 p < 1. Let `p denote the set of all sequences
x D .xn/11 of real or complex numbers satisfying the condition
1XiD1
jxijp < 1:
Define the operations of addition and scalar multiplication pointwise: For all x D .xn/, y D
.yn/ in `p and all ˛ 2 F, define
x C y D .x1 C y1; x2 C y2; : : :/
˛ x D .˛x1; ˛x2; : : :/:
3
2011
FUNCTIONAL ANALYSIS
ALP
Then `p is a linear space over F.
Proof. Let x D .x1; x2; : : :/, y D .y1; y2; : : :/ 2 `p. We must show that x C y 2 `p. Since,
for each i 2 N,
jxi C yijp Œ2 maxfjxij; jyijgp 2p maxfjxijp; jyijpg 2p .jxijp C jyijp/ ;
it follows that
1XiD1
Thus, x C y 2 `p. Also, if x D .xn/ 2 `p and ˛ 2 F, then
jxijp C
jxi C yijp 2p 1XiD1
j˛xijp D j˛jp 1XiD1
1XiD1
jyijp! < 1:
1XiD1
jxijp < 1:
That is, ˛ x 2 `p.
[6] The sequence space c D c.N/. Let c denote the set of all convergent sequences x D .xn/11 of
xn
real or complex numbers. That is, c is the set of all sequences x D .xn/11 such that
exists. Define the operations of addition and scalar multiplication pointwise as in example
(3). Then c is a linear space over F.
lim
n!1
[7] The sequence space c0 D c0.N/. Let c0 denote the set of all sequences x D .xn/11 of real
or complex numbers which converge to zero. That is, c0 is the space of all sequences
x D .xn/11 such that
xn D 0. Define the operations of addition and scalar multiplication
pointwise as in example (3). Then c0 is a linear space over F.
lim
n!1
[8] The sequence space `0 D `0.N/. Let `0 denote the set of all sequences x D .xn/11 of real or
complex numbers such that xi D 0 for all but finitely many indices i . Define the operations
of addition and scalar multiplication pointwise as in example (3). Then `0 is a linear space
over F.
4
2011
FUNCTIONAL ANALYSIS
ALP
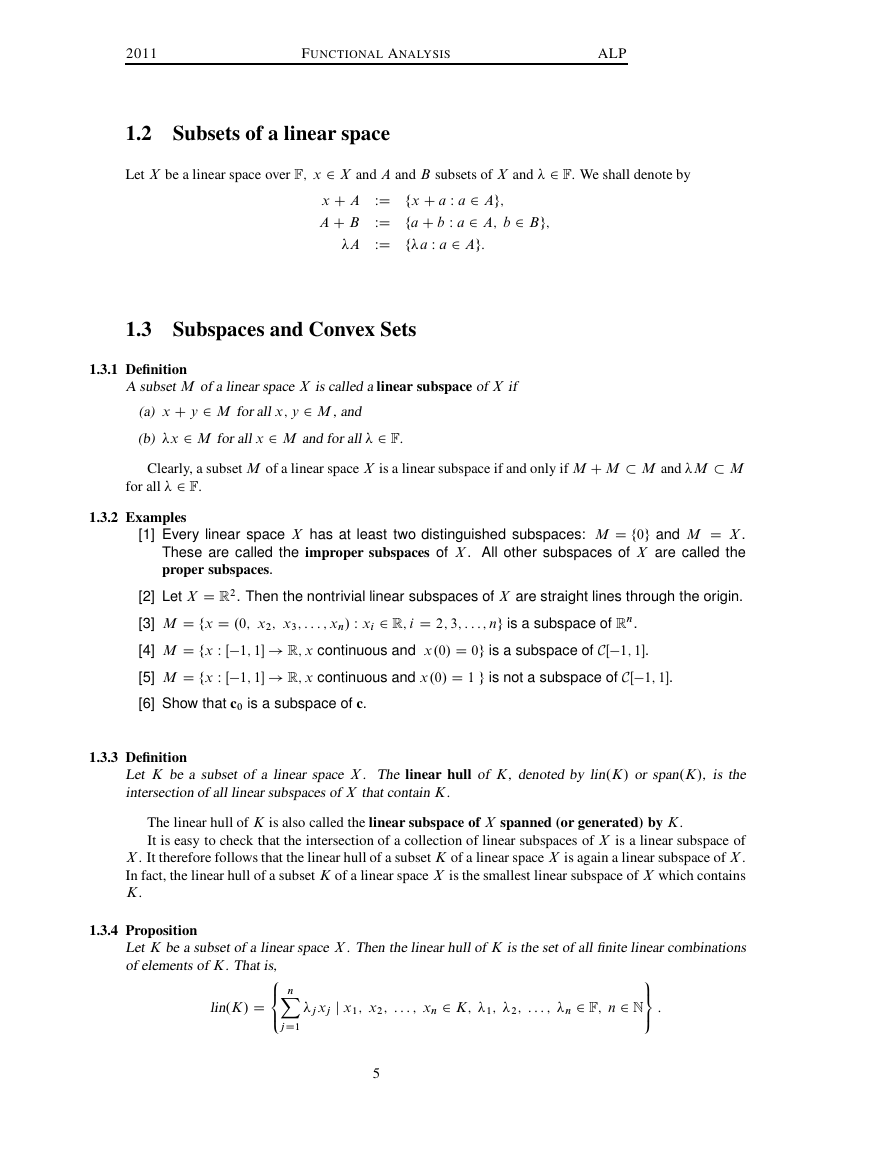
1.2 Subsets of a linear space
Let X be a linear space over F; x 2 X and A and B subsets of X and 2 F. We shall denote by
x C A WD fx C a W a 2 Ag;
A C B WD fa C b W a 2 A; b 2 Bg;
A WD fa W a 2 Ag:
1.3 Subspaces and Convex Sets
1.3.1 Definition
Asubset M of alinear space X iscalled a linear subspace of X if
(a) x C y 2 M forall x; y 2 M , and
(b) x 2 M for all x 2 M and for all 2 F.
Clearly, a subset M of a linear space X is a linear subspace if and only if M C M M and M M
for all 2 F.
1.3.2 Examples
[1] Every linear space X has at least two distinguished subspaces: M D f0g and M D X .
These are called the improper subspaces of X . All other subspaces of X are called the
proper subspaces.
[2] Let X D R2. Then the nontrivial linear subspaces of X are straight lines through the origin.
[3] M D fx D .0; x2; x3; : : : ; xn/ W xi 2 R; i D 2; 3; : : : ; ng is a subspace of Rn.
[4] M D fx W Œ1; 1 ! R; x continuous and x.0/ D 0g is a subspace of CŒ1; 1.
[5] M D fx W Œ1; 1 ! R; x continuous and x.0/ D 1 g is not a subspace of CŒ1; 1.
[6] Show that c0 is a subspace of c.
1.3.3 Definition
Let K be a subset of a linear space X . The linear hull of K, denoted by lin.K/ or span.K/, is the
intersectionof all linear subspaces of X that contain K.
The linear hull of K is also called the linear subspace of X spanned (or generated) by K.
It is easy to check that the intersection of a collection of linear subspaces of X is a linear subspace of
X . It therefore follows that the linear hull of a subset K of a linear space X is again a linear subspace of X .
In fact, the linear hull of a subset K of a linear space X is the smallest linear subspace of X which contains
K.
1.3.4 Proposition
Let K be a subset of a linear space X . Then thelinear hull of K is the set of all finitelinear combinations
of elements of K. That is,
lin.K/ D8<:
nXjD1
j xj j x1; x2; : : : ; xn 2 K; 1; 2; : : : ; n 2 F; n 2 N9=;
:
5
相关推荐
2023年江西萍乡中考道德与法治真题及答案.doc
2012年重庆南川中考生物真题及答案.doc
2013年江西师范大学地理学综合及文艺理论基础考研真题.doc
2020年四川甘孜小升初语文真题及答案I卷.doc
2020年注册岩土工程师专业基础考试真题及答案.doc
2023-2024学年福建省厦门市九年级上学期数学月考试题及答案.doc
2021-2022学年辽宁省沈阳市大东区九年级上学期语文期末试题及答案.doc
2022-2023学年北京东城区初三第一学期物理期末试卷及答案.doc
2018上半年江西教师资格初中地理学科知识与教学能力真题及答案.doc
2012年河北国家公务员申论考试真题及答案-省级.doc
2020-2021学年江苏省扬州市江都区邵樊片九年级上学期数学第一次质量检测试题及答案.doc
2022下半年黑龙江教师资格证中学综合素质真题及答案.doc
